A brass of length 2m and cross-sectional area 2.0cm2 is attached end to end to a steel rod of length and cross-sectional area 1.0cm2. The compound rod is subjected to equal and oppsite pulls of magnitude 5×104N at its ends. If the elongations of the two rods are equal, the length of the steel rod (L) is
{YBrass=1.0×1011N/m2 and Ysteel=2.0×1011N/m2
1.5 m
1.8 m
1 m
2 m
The correct Answer is:D
To solve the problem, we need to find the length of the steel rod (L) when a brass rod and a steel rod are connected end to end and subjected to equal and opposite pulls. The elongations of both rods are equal.
1. Identify Given Data:
- Length of brass rod, Lbrass=2m
- Cross-sectional area of brass rod, Abrass=2.0cm2=2.0×10−4m2
- Cross-sectional area of steel rod, Asteel=1.0cm2=1.0×10−4m2
- Young's modulus of brass, Ybrass=1.0×1011N/m2
- Young's modulus of steel, Ysteel=2.0×1011N/m2
- Force applied, F=5×104N
2. Use the Formula for Young's Modulus:
The formula for Young's modulus is given by:
Y=StressStrain=F/AΔL/L0
Rearranging gives:
ΔL=FL0AY
3. Calculate Elongation of Brass Rod:
For the brass rod:
ΔLbrass=FLbrassAbrassYbrass=(5×104)×2(2.0×10−4)×(1.0×1011)
Simplifying this:
ΔLbrass=1052.0×10−4×1.0×1011=1052.0×107=0.005m=5mm
4. Set Up Equation for Steel Rod:
Since the elongations are equal:
ΔLsteel=ΔLbrass
Thus,
ΔLsteel=FLsteelAsteelYsteel=5mm
5. Calculate Elongation of Steel Rod:
5×10−3=(5×104)Lsteel(1.0×10−4)×(2.0×1011)
Rearranging gives:
Lsteel=5×10−3×(1.0×10−4)×(2.0×1011)5×104
Simplifying:
Lsteel=(1.0×10−4)×(2.0×1011)104=2.0×107104=2000m=2m
6. Final Answer:
The length of the steel rod Lsteel=2m.
- Length of brass rod, Lbrass=2m
- Cross-sectional area of brass rod, Abrass=2.0cm2=2.0×10−4m2
- Cross-sectional area of steel rod, Asteel=1.0cm2=1.0×10−4m2
- Young's modulus of brass, Ybrass=1.0×1011N/m2
- Young's modulus of steel, Ysteel=2.0×1011N/m2
- Force applied, F=5×104N
The formula for Young's modulus is given by:
Y=StressStrain=F/AΔL/L0
Rearranging gives:
ΔL=FL0AY
For the brass rod:
ΔLbrass=FLbrassAbrassYbrass=(5×104)×2(2.0×10−4)×(1.0×1011)
Simplifying this:
ΔLbrass=1052.0×10−4×1.0×1011=1052.0×107=0.005m=5mm
Since the elongations are equal:
ΔLsteel=ΔLbrass
Thus,
ΔLsteel=FLsteelAsteelYsteel=5mm
5×10−3=(5×104)Lsteel(1.0×10−4)×(2.0×1011)
Rearranging gives:
Lsteel=5×10−3×(1.0×10−4)×(2.0×1011)5×104
Simplifying:
Lsteel=(1.0×10−4)×(2.0×1011)104=2.0×107104=2000m=2m
The length of the steel rod Lsteel=2m.
Topper's Solved these Questions
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Match the columns
4 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Medical entrances s gallery
21 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Check point 12.3
15 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
469 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer
17 Videos
Similar Questions
A copper rod with length 1.4 m and area of cross-section of 2cm2 is fastened to a steel rod with length L and cross-sectional area 1cm2. The compound rod is subjected to equal and opposite pulls to magnitude 6.00×104 N at its ends .
(a) Find the length L of the steel rod if the elongation of the two rods are equal .
(b) What is stress in each rod ?
(c ) What is the strain in each rod ? [Ysteel=2×1011Pa,YCu=1.1×1011Pa]

View SolutionDetermine the force required to double the length of the steel wire of area of cross-section 5×10−5m2. Give Y for steel =2×1011Nm−2.
View SolutionA steel rod 2.0 m long has a cross-sectional area of 0.30cm2. The rod is now hung by one end from a support structure, and a 550 kg milling machine is hung from the strain, and the elongation of the rod.
View SolutionA uniform cylindrical rod of length L and cross-sectional area by forces as shown in figure.
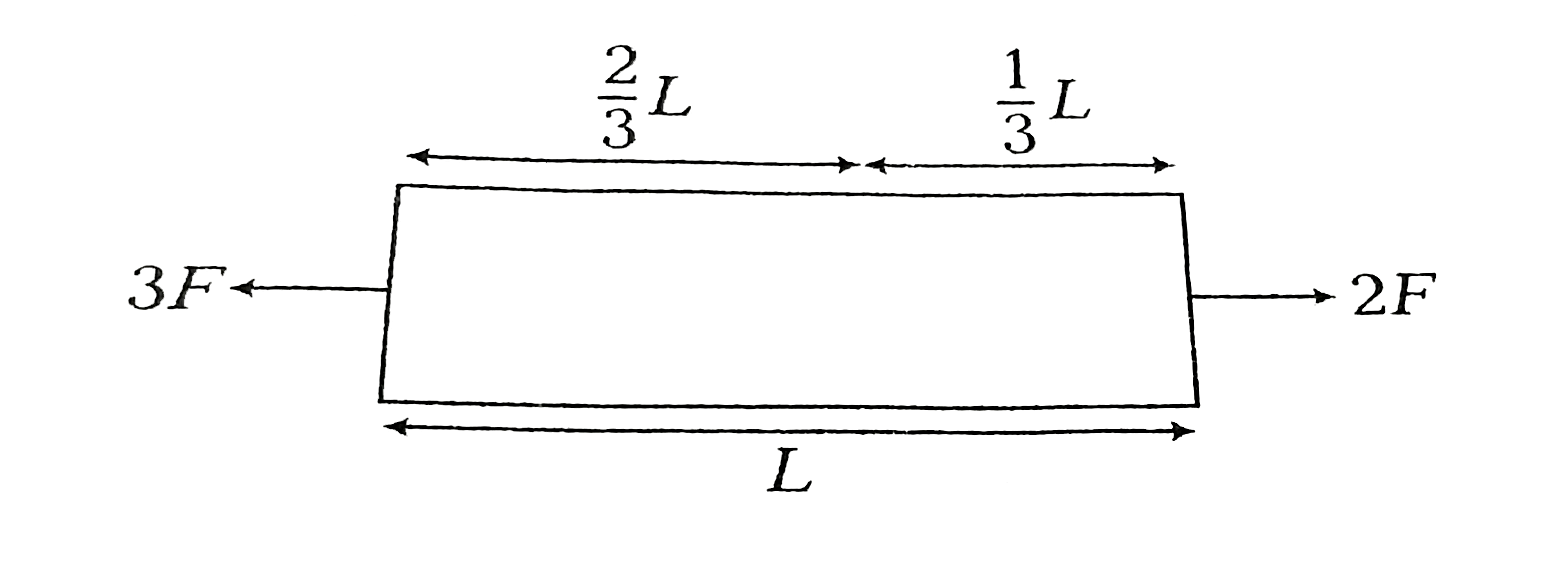
The elongation produced in the rod is
View SolutionDetermine the elongation of the steel bar 1m long and 1.5cm2 cross-sectional area when subjected to a pull of 1.5×104N.
(TakeY=2.0×1011N/m2).
View SolutionDetermine the elongation of the steel bar 1m long and 1.5cm2 cross-sectional area when subjected to a pull of 1.5×104N.
(Take Y=2.0×1011N/m2).
View SolutionIn an experiment, brass and steel wires of length 1 m each with areas of cross section 1mm2 are used. The wires are connected in series and one end of the combined wire is connected to a rigid support and other end is subjected to elongation. The stress required to produce a net elongation of 0.2 mm is, [Given, the Young’s Modulus for steel and brass are, respectively, 120×109N/m2and60×109N/m2
View SolutionIn an experiment, brass and steel wires of length 1 m each with areas of cross section 1mm2 are used. The wires are connected in series and one end of the combined wire is connected to a rigid support and other end is subjected to elongation. The stress required to produce a net elongation of 0.2 mm is, [Given, the Young’s Modulus for steel and brass are, respectively, 120×109N/m2and60×109N/m2
View SolutionA steel wire and a copper wire of equal length and equal cross- sectional area are joined end to end and the combination is subjected to a tension. Find the ratio of
copper/steel
(a) the stresses developed . In the two wires,
(b) the strains developed. (Y of steel =2×1011N/m2)
View SolutionA composite rod consists of a steel rod of length 25cm and area 2A and a copper rod of length 50cm and area A. The composite rod is subjected to an axial load F. If the Young's moduli of steel and copper are in the ratio 2:1 then
View Solution
