A man weighing 60 kg is standing on a trolley weighing 240 kg. The trolley is resting on a frictionless horizontal rails. If the man starts walking on the trolley along the rails at speed 1m/s, then after 4 seconds, his displacement relative to the ground will be
6 m
4.8 m
3.2 m
2.4 m
The correct Answer is:c
To solve the problem step by step, we will use the principle of conservation of momentum. Here’s how we can approach it:
Step 1: Identify the masses and velocities
- Mass of the man (m1) = 60 kg
- Mass of the trolley (m2) = 240 kg
- Velocity of the man relative to the trolley (v1) = 1 m/s (forward)
- Velocity of the trolley (v2) = ? (backward, to be determined)
Step 2: Apply the conservation of momentum
Since the trolley is on frictionless rails, the total momentum before the man starts walking must equal the total momentum after he starts walking. Initially, both the man and the trolley are at rest, so the initial momentum is 0.
When the man starts walking, the momentum can be expressed as:
m1⋅v1+m2⋅v2=0
Substituting the known values:
60⋅1+240⋅(−v)=0
Here, we take the velocity of the trolley as negative because it moves in the opposite direction to the man.
Step 3: Solve for the trolley's velocity (v2)
Rearranging the equation:
60−240v=0
240v=60
v=60240=14=0.25 m/s
Step 4: Determine the man's velocity relative to the ground
The man is walking forward at 1 m/s, while the trolley moves backward at 0.25 m/s. Therefore, the man's velocity relative to the ground (v_relative) is:
vrelative=v1−v2
vrelative=1−0.25=0.75 m/s
Step 5: Calculate the displacement after 4 seconds
To find the displacement of the man relative to the ground after 4 seconds, we use the formula:
Displacement=vrelative⋅t
Where t=4 seconds.
Displacement=0.75⋅4=3 meters
Final Answer
The displacement of the man relative to the ground after 4 seconds is 3 meters.
---
- Mass of the man (m1) = 60 kg
- Mass of the trolley (m2) = 240 kg
- Velocity of the man relative to the trolley (v1) = 1 m/s (forward)
- Velocity of the trolley (v2) = ? (backward, to be determined)
Since the trolley is on frictionless rails, the total momentum before the man starts walking must equal the total momentum after he starts walking. Initially, both the man and the trolley are at rest, so the initial momentum is 0.
m1⋅v1+m2⋅v2=0
60⋅1+240⋅(−v)=0
Here, we take the velocity of the trolley as negative because it moves in the opposite direction to the man.
Rearranging the equation:
60−240v=0
240v=60
v=60240=14=0.25 m/s
The man is walking forward at 1 m/s, while the trolley moves backward at 0.25 m/s. Therefore, the man's velocity relative to the ground (v_relative) is:
vrelative=v1−v2
vrelative=1−0.25=0.75 m/s
To find the displacement of the man relative to the ground after 4 seconds, we use the formula:
Displacement=vrelative⋅t
Where t=4 seconds.
Displacement=0.75⋅4=3 meters
The displacement of the man relative to the ground after 4 seconds is 3 meters.
Topper's Solved these Questions
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - Dynamics ( WORK POWER ENERGY )
35 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - FRICTION
22 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - Dynamics (PROJECTILE MOTION)
31 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- DIMENSIONSBOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
9 Videos
Similar Questions
A man weighing 80kg is standing on a trolley weighting 320kg. The trolley is resting on frictionless horizontal rails. If the man starts walking on the trolley along the rails at speed 1m/s (w.r.t. to trolley) then after 4s his displacement relative to the ground will be :
View SolutionA man of mass m is standing at one end of of a plank of mass M. The length of the plank is L and it rests on a frictionless horizontal ground. The man walks to the other end of the plank. Find displacement of the plank and man relative to the ground.

View SolutionA man of mass m is standing on a stationary flat car of mass M. The car can move without friction along horizontal rails. The man starts walking with velocity v relative to the car. Work done by him
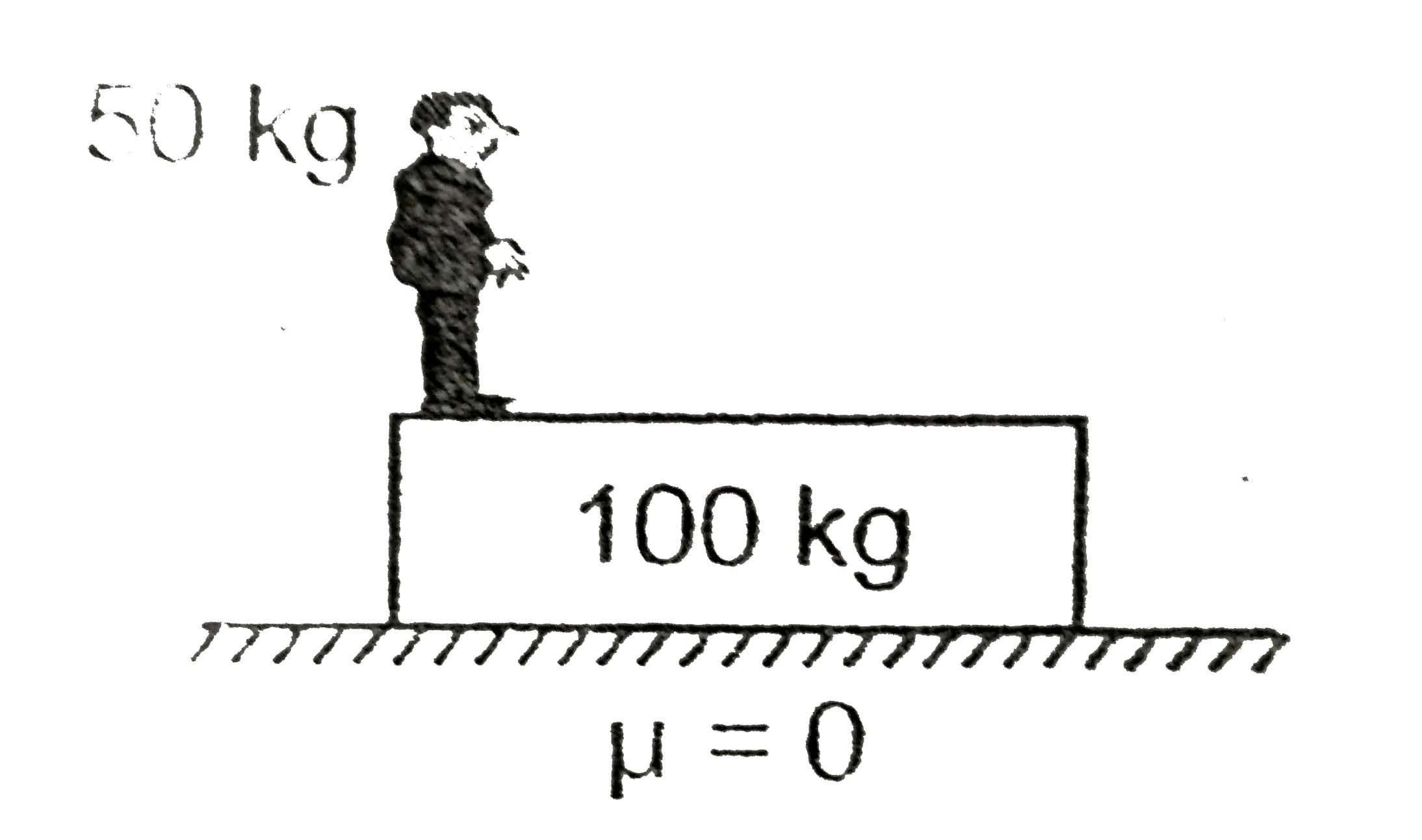
View SolutionA man of mass 50kg us standing on a 100kg plank kept on a fricitonless horizontal floor. Initially both are at rest. If the man starts walking on the plank with speed 6m/s towards right relative to the plank, then amount of muscle energy spent by the man is
View SolutionA man of mass m is standing on a plank of equal mass m resting on a smooth horizontal surface. The man starts moving on the plank with speed u relative to the plank. The speed of the man relative to the ground is

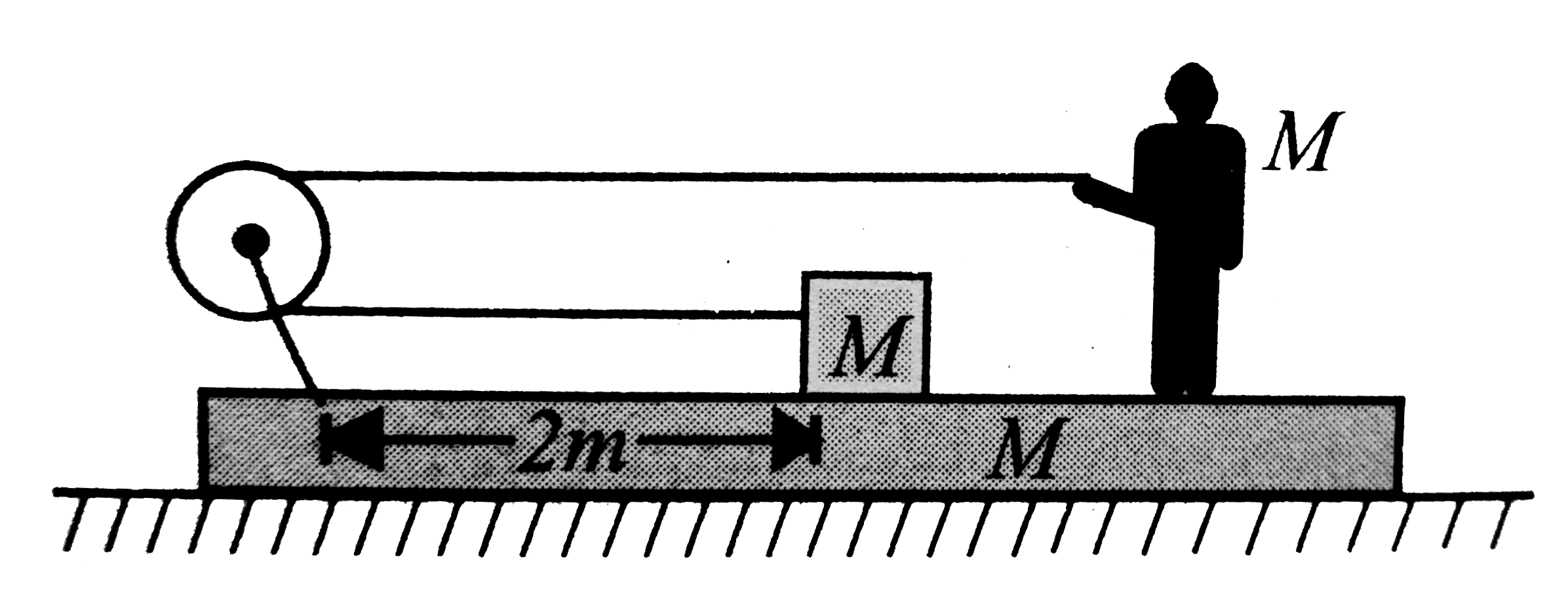
View SolutionA block of mass M is tied to one end of a massless rope. The other end of the rope is in the hands of a man of mass 2M as shown in Fig. The block and the man art resting on a rough wedge of mass M. The whole system is resting on a smooth horizontal surface. The man starts walking towards right while holding the rope in his hands. Pulley is massless and frictionless. Find the displacement of the wedge when the block meets the pulley. Assume wedge is sufficiently long so that man does not fall down.
View SolutionA man of mass M stands at one end of a plank of length L Which lies at rest on a frictionless surface . The man walks to the other end of the plank. If the mass of the plank is 3M, the distance that the man moves relative to the ground is
View SolutionA man of mass 80kg stands on a plank of mass 40kg. The plank is lying on a smooth horizontal floor. Initianlly both are at rest. The man starts walking on the plank towards north and stops after moving a distance of 6m on the plank. Then
View SolutionA man of mass 60 kg is standing on a boat of mass 140 kg, which is at rest in still water. The man is initially at 20 m from the shore. He starts walking on the boat for 4 s with constant speed 1.5 m/s towards the shore. The final distance of the man from the shore is
View SolutionA man stands at one end of the open truck which can run on frictionless horizontal rails. Initially, the man and the truck are at rest. Man now walks to the other end and stops. Then which of the following is true?
View Solution
