Five charges, q each are placed at the corners of a regular pentagon of side a.
(i) What will be the electric field at O if the charge from one of the corners (say A) is removed ?
(ii) What will be the electric field at O if the charge gat A is replaced by -q?
To solve the problem step by step, we will analyze both parts of the question regarding the electric field at point O when one charge is removed and when it is replaced with a negative charge.
Given:
- Five charges q are placed at the corners of a regular pentagon of side a.
- The center of the pentagon is denoted as point O.
Part (i): Electric Field at O when Charge at A is Removed
1. Understanding the Geometry:
- The pentagon has five corners (A, B, C, D, E) with a charge q at each corner.
- The center O is equidistant from all corners.
2. Electric Field Contribution:
- When charge at corner A is removed, we have four charges left (B, C, D, E).
- The electric field due to each of these charges at point O will be directed away from the charges since they are positive.
3. Symmetry Consideration:
- The electric fields due to charges at corners B, C, D, and E will have symmetrical components.
- The resultant electric field due to charges B, C, D, and E will not cancel out completely because charge A is missing.
4. Calculating the Electric Field:
- The electric field E due to one charge q at distance D from point O is given by:
E=kqD2
- Since there are four charges, and the symmetry leads to a resultant field along the line OA, the net electric field at O will be:
Enet=kqD2(in the direction of OA)
- Thus, the electric field at O when charge A is removed is:
Enet=kqD2^OA
Part (ii): Electric Field at O when Charge at A is Replaced by -q
1. Replacing Charge:
- Now, we replace the charge at corner A with a charge of −q.
2. Electric Field Contribution:
- The electric field due to the charge at A (which is now −q) will be directed towards charge A (attracting towards it).
- The electric fields due to charges at B, C, D, and E remain the same as in part (i).
3. Calculating the Electric Field:
- The electric field due to charge −q at point O is:
EA=k(−q)D2=−kqD2(towards A)
- The resultant electric field at O will be the vector sum of the fields due to charges B, C, D, E and the field due to charge A:
Enet=(kqD2+kqD2)^OA=2kqD2^OA
Final Answers:
- (i) The electric field at O when charge A is removed:
Enet=kqD2^OA
- (ii) The electric field at O when charge A is replaced by −q:
Enet=2kqD2^OA
- Five charges q are placed at the corners of a regular pentagon of side a.
- The center of the pentagon is denoted as point O.
- The pentagon has five corners (A, B, C, D, E) with a charge q at each corner.
- The center O is equidistant from all corners.
- When charge at corner A is removed, we have four charges left (B, C, D, E).
- The electric field due to each of these charges at point O will be directed away from the charges since they are positive.
- The electric fields due to charges at corners B, C, D, and E will have symmetrical components.
- The resultant electric field due to charges B, C, D, and E will not cancel out completely because charge A is missing.
- The electric field E due to one charge q at distance D from point O is given by:
E=kqD2
- Since there are four charges, and the symmetry leads to a resultant field along the line OA, the net electric field at O will be:
Enet=kqD2(in the direction of OA)
- Thus, the electric field at O when charge A is removed is:
Enet=kqD2^OA
- Now, we replace the charge at corner A with a charge of −q.
- The electric field due to the charge at A (which is now −q) will be directed towards charge A (attracting towards it).
- The electric fields due to charges at B, C, D, and E remain the same as in part (i).
- The electric field due to charge −q at point O is:
EA=k(−q)D2=−kqD2(towards A)
- The resultant electric field at O will be the vector sum of the fields due to charges B, C, D, E and the field due to charge A:
Enet=(kqD2+kqD2)^OA=2kqD2^OA
- (i) The electric field at O when charge A is removed:
Enet=kqD2^OA
- (ii) The electric field at O when charge A is replaced by −q:
Enet=2kqD2^OA
Similar Questions
Five charges, q each are placed at the coreners of a regular pentagone of side.
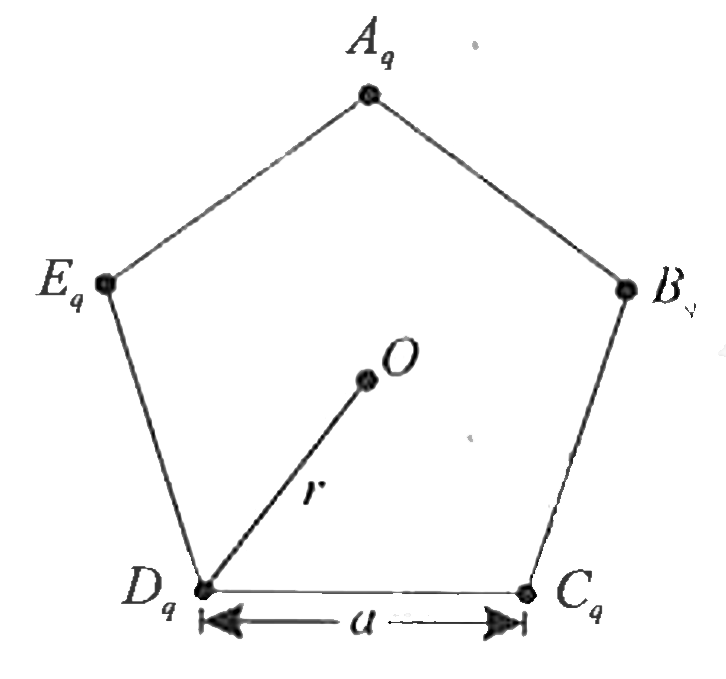
(a) (i) What will be the electric field at O, the centre of the pentagon ?
(ii) What will be the electric field 0 if the charge from one of the corners (say A) is removed ?
(iii) What will be the electric field at 0 if the charge q at A is replaced by −q ?
(b) How would your answer to (a) be affected if pentagon is replaced by n-sided regular polygon with charge q at each of its coreners ?
View SolutionFive equal charges each of value q are placed at the corners of a regular pentagon of side a. The electric field at the centre of the pentagon is

View Solution
Knowledge Check
In question number 45, what will be the electric field at centre O, if the charge from one of the corners (say A) is removed?
Aq4πε0r2 along OAB2q4πε0r2 along OBCq24πε0r2 along OCD2q4πε0r2 along OAIn question number 45, what will be the electric field at O if the charge q at A is replaced by -q?
Aq4πε0r2 along OBB2q4πε0r2 along OAC4q4πε0r2 along OCDzero
Three charges, each equal to q, are placed at the three. corners of a square of side a . Find the electric field at. the fourth corner.
View SolutionThree charges, each equal to q, are placed at the three. corners of a square of side a . Find the electric field at. the fourth corner.
View SolutionFive point charge (+q each) are placed at the five vertices of a regular hexagon of side 2a.what is the magnitude of the net electric field at the centre of the hexazon?
View SolutionA planet travels in an elliptical orbit about a star as shown. At what pair of points is the speed of the planet the same?
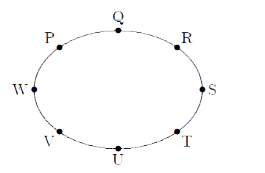
View SolutionThree eqal charges +Q each are at the corners of an equilibrium triangle as shown. The intensity of electric field at O will be

View SolutionThree equal charges, each +q are placed on the corners of an equilatreal triangel . The electric field intensity at the centroid of the triangle is
View Solution
