A uniform half metre rule balances horizontally on a knife edge at 29 cm mark when a weight of 20 gf is suspended from one end.
What is the weight of the half metre rule ?
To solve the problem, we need to find the weight of the half-meter rule (let's denote it as W). The rule balances horizontally at the 29 cm mark when a weight of 20 gf is suspended from one end (the 50 cm mark).
1. Identify the Setup:
- The half-meter rule is 50 cm long.
- The knife edge is at the 29 cm mark.
- A weight of 20 gf is suspended at the 50 cm mark.
2. Determine the Distances:
- The distance from the knife edge (29 cm) to the weight (50 cm) is:
d1=50cm−29cm=21cm
- The weight of the half-meter rule acts at its center of mass, which is at the 25 cm mark. The distance from the knife edge (29 cm) to the center of mass (25 cm) is:
d2=29cm−25cm=4cm
3. Set Up the Torque Balance Equation:
- The torque due to the weight of the half-meter rule (acting downwards) is:
Torque due to W=W×d2=W×4
- The torque due to the suspended weight (20 gf) is:
Torque due to 20gf=20gf×d1=20gf×21
4. Equate the Torques:
- For the system to be in equilibrium, the clockwise torque must equal the anticlockwise torque:
W×4=20×21
5. Solve for W:
- Rearranging the equation gives:
W=20×214
- Calculating the right side:
W=4204=105gf
6. Conclusion:
- The weight of the half-meter rule is 105gf.
- The half-meter rule is 50 cm long.
- The knife edge is at the 29 cm mark.
- A weight of 20 gf is suspended at the 50 cm mark.
- The distance from the knife edge (29 cm) to the weight (50 cm) is:
d1=50cm−29cm=21cm
- The weight of the half-meter rule acts at its center of mass, which is at the 25 cm mark. The distance from the knife edge (29 cm) to the center of mass (25 cm) is:
d2=29cm−25cm=4cm
- The torque due to the weight of the half-meter rule (acting downwards) is:
Torque due to W=W×d2=W×4
- The torque due to the suspended weight (20 gf) is:
Torque due to 20gf=20gf×d1=20gf×21
- For the system to be in equilibrium, the clockwise torque must equal the anticlockwise torque:
W×4=20×21
- Rearranging the equation gives:
W=20×214
- Calculating the right side:
W=4204=105gf
- The weight of the half-meter rule is 105gf.
Topper's Solved these Questions
Similar Questions
A uniform half metre rule balances horizontally on a knife edge at 29 cm mark when a weight of 20 gf is suspended from one end.
Draw a diagram of the arrangement.
View SolutionA uniform metre rule between horizontally on a knife edge placed at the 58cm mark when a weight of 20gf is suspended from one end.
(i) Draw a diagram of the arrangement.
(ii) What is the weight of the rule?
View Solution
Knowledge Check
Find the weight of half meter scale balanced at 5 cm mark when a weight of 10 gf is suspended at one of its ends.
A2.5gfB100gfC0.2gfD50gfA uniform rod of length 1m mass 4kg is supports on tow knife-edges placed10cm from each end. A 60N weight is suspended at 30cm from one end. The reactions at the knife edges is.
Aa. 60 N, 40 NBb. 75 N, 25 NCc. 65 N, 35 NDd. 55 N, 45 N
A uniform metre rule rests horizontally on a knife edge at the 60cm mark when a mass of 10g is suspended from one end. Draw a diagram of the arrangement.
(a) At which end must this mass be suspended
(b) What is the mass of the rule?
(c ) THe 10g mass is now shifted to the 90cm mark. In which direction must the knife edge be shifted to make the rule horizontal again?
View SolutionA uniform half metre rule can be balanced at the 29.0cm mark when a mass 20g is hung from its one end.
(a) Draw a diagram of the arrangement
(b) Find the mass of the half metre rule.
( c) In Which direction would the balancing point shift if 20g mass is shifted inside from its one end?
View SolutionA uniform metre rule is pivoted as its mid point. A weight of 50gf is suspended at one end of it. Where should a weight of 100gf be suspended to keep the rule horizontal?
View SolutionA uniform metre scale can be balanced at the 70.0 cm mark when a mass of 0.05 kg is hung from the 94.0 cm mark.
Find the mass of the metre scale.
View SolutionThe diagram alongside shows a uniform metre rule of weight 100 gf being balanced on a knife edge placed at the 40 cm mark, by suspending a weight w gf at the mark 20 cm, find:
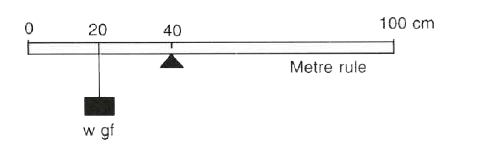
the position of another weight of 50 gf to balance the rule in part (ii).
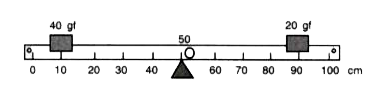
View SolutionFig.1.33 shows a uniform metre rule placed on a fulcrum at its mid point O and having a weight 40gf at the 10cm mark and a weight of 20gf at the 90cm mark. (i) Is the metre rule in equilibrium? If not , how will the turn?
(ii) How can the rule be brought in equilibrium by using an additional weight of 40 gf?
View Solution
