In a survery of 200 employees of a company, it was found that 89 liked the lunch packs provided by the Company while the rest did not. Out of these employees, if one employee is chosen at random, what is the probability that the chosen employee:
(i) likes the lunch pack? (ii) dislikes the lunch pack?
The correct Answer is:(i) 89200, (ii) 111200
To solve the problem step by step, we will calculate the probabilities for both parts of the question.
1. Identify the Total Number of Employees:
- The total number of employees surveyed is given as 200.
2. Identify the Number of Employees Who Liked the Lunch Packs:
- It is stated that 89 employees liked the lunch packs.
3. Calculate the Number of Employees Who Disliked the Lunch Packs:
- To find the number of employees who disliked the lunch packs, we subtract the number of employees who liked the lunch packs from the total number of employees.
- Number of employees who disliked the lunch packs = Total employees - Employees who liked lunch packs
- Number of employees who disliked the lunch packs = 200 - 89 = 111
4. Calculate the Probability That a Randomly Chosen Employee Likes the Lunch Pack:
- The probability (P) that a randomly chosen employee likes the lunch pack is given by the formula:
P(likes lunch pack)=Number of employees who liked lunch packsTotal number of employees
- Substituting the values:
P(likes lunch pack)=89200=0.445
5. Calculate the Probability That a Randomly Chosen Employee Dislikes the Lunch Pack:
- The probability that a randomly chosen employee dislikes the lunch pack can be calculated as:
P(dislikes lunch pack)=1−P(likes lunch pack)
- Substituting the value we found:
P(dislikes lunch pack)=1−0.445=0.555
Final Answers:
- (i) The probability that the chosen employee likes the lunch pack is 0.445.
- (ii) The probability that the chosen employee dislikes the lunch pack is 0.555.
- The total number of employees surveyed is given as 200.
- It is stated that 89 employees liked the lunch packs.
- To find the number of employees who disliked the lunch packs, we subtract the number of employees who liked the lunch packs from the total number of employees.
- Number of employees who disliked the lunch packs = Total employees - Employees who liked lunch packs
- Number of employees who disliked the lunch packs = 200 - 89 = 111
- The probability (P) that a randomly chosen employee likes the lunch pack is given by the formula:
P(likes lunch pack)=Number of employees who liked lunch packsTotal number of employees
- Substituting the values:
P(likes lunch pack)=89200=0.445
- The probability that a randomly chosen employee dislikes the lunch pack can be calculated as:
P(dislikes lunch pack)=1−P(likes lunch pack)
- Substituting the value we found:
P(dislikes lunch pack)=1−0.445=0.555
- (i) The probability that the chosen employee likes the lunch pack is 0.445.
- (ii) The probability that the chosen employee dislikes the lunch pack is 0.555.
Topper's Solved these Questions
- PROBABILITYBOOK - ICSECHAPTER - PROBABILITYEXERCISE - EXAMPLE
7 Videos
- PROBABILITYBOOK - ICSECHAPTER - PROBABILITYEXERCISE - EXERCISE 19.1
34 Videos
- PROBABILITYBOOK - ICSECHAPTER - PROBABILITYEXERCISE - TRY THIS
14 Videos
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - TRY THIS
41 Videos
- PROFIT AND LOSS BOOK - ICSECHAPTER - PROFIT AND LOSS EXERCISE - EXERCISE 10
18 Videos
Similar Questions
In a school, 300 students were surveyed. The survey showed that 186 students liked watching cricket match while the rest disliked it. Out of these students, if one student is chosen at random, what is the probability that the chosen student :
(i) likes watching cricket match?
(ii) dislikes watching cricket match?
View SolutionA survey of 300 girls of a school was conducted and it was found that 108 girls like tea and 192 dislike it. Out of these girls one girl is selected at random. What is the probability that the selected girl (i) likes tea (ii) does not like tea.
View SolutionTo know the opinion of the students about Mathematics, a survey of 200 students was conducted. The data is recorded in the following table: Find the probability that a student chosen at random (i) likes Mathematics (ii) does not like it.
View SolutionOn a particular day, at a crossing in a city, the various types of 280 vehicles going past during a time interval were observed as under:
∣∣∣Type of vehicleTwo wheelersThree wheelersFour wheelersFrequency 91 63 126∣∣∣
Out of these vechicles , one is chosen at random, what is the probability that the chosen vechicle is
(i) a four wheeler
(ii) a two wheeler
(iii) a three wheeler?
View SolutionFrom the employees of a company, 5 persons are selected to represent them in the managing committee of the company. Particulars of five persons are as follows:
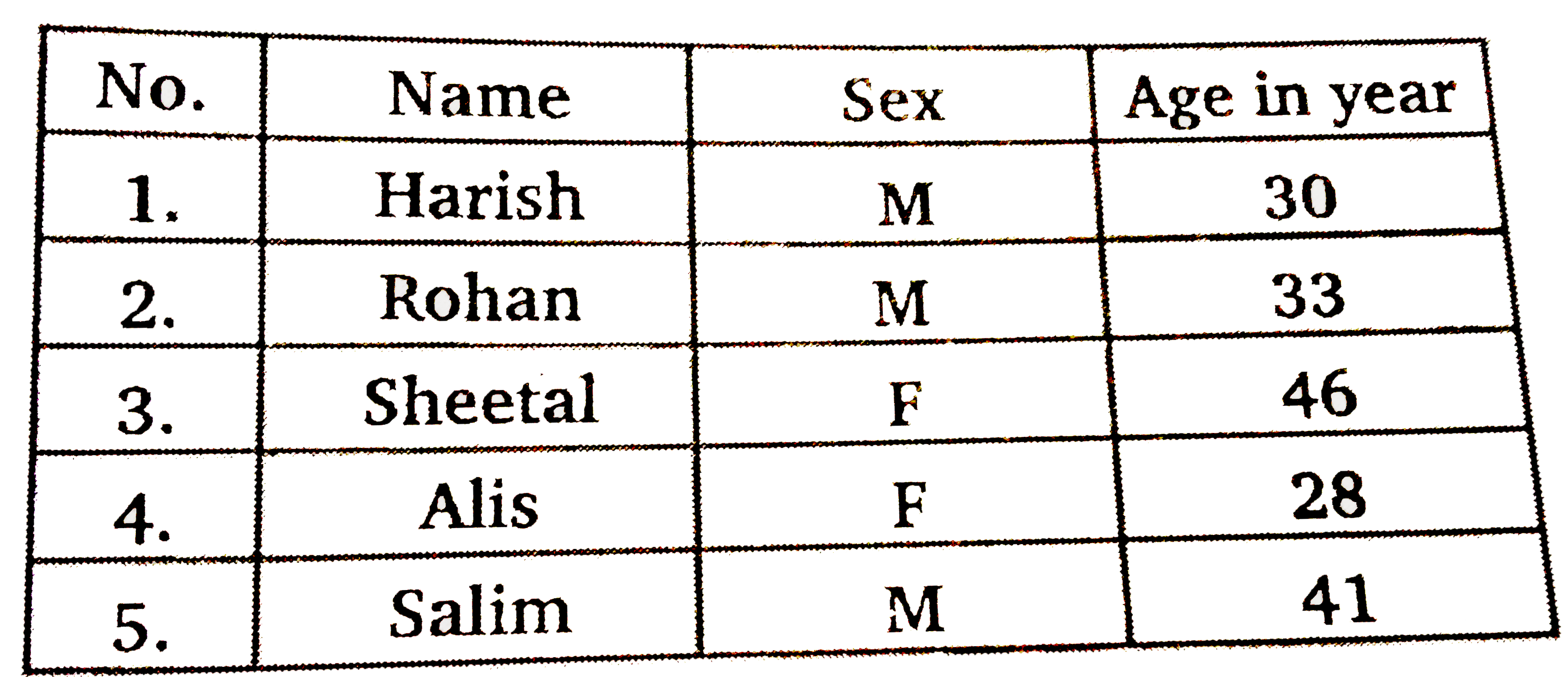
A person is selected at random from this group to act as a spokeperson. What is the probability that the spokesperson wil be either male or over 35 years?
View Solution10 packets of suger each marked 1 kg actually contained the following weights 0.6 kg, 1.050 kg, 1.1 kg, -0.98 kg, 0.92 kg, 1.3 kg, 1.4 kg, 1.00 kg, 0.94 kg, 1.03 kg. Out of these packets, one packet is chosen at random, what is the probability that the chosen packet contain
(i) more than 1 kg sugar,
(ii) 1 kg sugar,
(iii) less than 1 kg sugar?
View SolutionTo know the opinion of the students about Mathematics, a survey of 200 students was conducted. The data is recorded in the following table: Opinion: Like Dislike No. of students 135 65 Find the probability that a student chosen at random (i) like Mathematics (ii) does not like it.
View SolutionBulbs are packed in cartons each containing 40 bulbs, seven hundred cartons were examined for defective bulbs and the results are given in the following table.
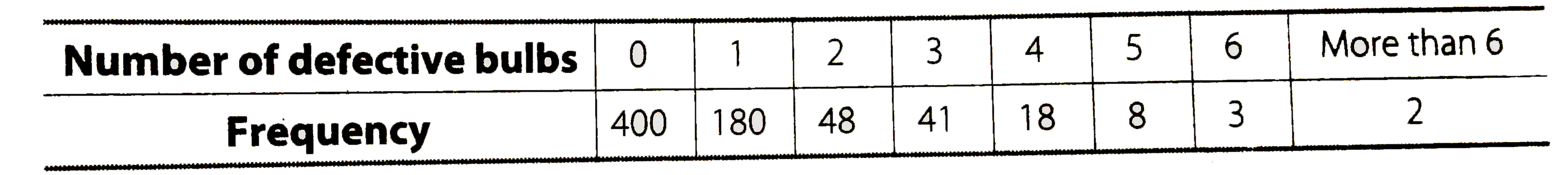
One carton was selected at random. What is the probability that it has
i) no defective bulb?
ii) defective bulbs from 2-6?
iii) defective bulbs less than 4?
View SolutionA company selected 2400 families at random and survey them to determine a relationship between income level and the number of vehicles in a home. The information gathered is listed in the table below: If a family is chosen, find the probability that the family is: (i) earning Rs.10000-13000 per month and owning exactly 2 vehicles. (ii) earning Rs. 16000 or more per month and owning exactly 1 vehicle (iii) Earning less than Rs. 7000 per month and does not own any vehicle. (iv) earning Rs.13000-16000 per month and owning more than 2 vehicle. (v) owning not more than 1 vehicle. (vi) owning at least one vehicle.
View SolutionOne card is drawn from a pack of 52 cards, each of the 52 cards being equally likely to be drawn. Find the probability that the card drawn is: (i) an ace (ii) red (iii) either red or king (iv) red and a king.
View Solution
