A company selected 2400 families at random and survey them to determine a relationship between income level and the number of vehicles in a home. The information gathered is listed in the table below: If a family is chosen, find the probability that the family is: (i) earning Rs.10000-13000 per month and owning exactly 2 vehicles. (ii) earning Rs. 16000 or more per month and owning exactly 1 vehicle (iii) Earning less than Rs. 7000 per month and does not own any vehicle. (iv) earning Rs.13000-16000 per month and owning more than 2 vehicle. (v) owning not more than 1 vehicle. (vi) owning at least one vehicle.
To solve the problem step by step, we will calculate the probabilities for each part of the question based on the provided data. Here’s the structured solution:
Given Data:
Total number of families surveyed = 2400
Step 1: Understanding the Table
We need to analyze the data in the table, which categorizes families based on their income levels and the number of vehicles they own. We will extract the relevant figures for each scenario.
Step 2: Calculating Probabilities
(i) Earning Rs. 10,000-13,000 per month and owning exactly 2 vehicles
- Number of families earning Rs. 10,000-13,000 and owning exactly 2 vehicles = 29
- Probability (P(A)) = Number of favorable outcomes / Total outcomes = 29 / 2400
Calculation:
P(A)=292400
(ii) Earning Rs. 16,000 or more per month and owning exactly 1 vehicle
- Number of families earning Rs. 16,000 or more and owning exactly 1 vehicle = 579
- Probability (P(B)) = 579 / 2400
Calculation:
P(B)=5792400
(iii) Earning less than Rs. 7,000 per month and does not own any vehicle
- Number of families earning less than Rs. 7,000 and owning 0 vehicles = 10
- Probability (P(C)) = 10 / 2400
Calculation:
P(C)=102400=1240
(iv) Earning Rs. 13,000-16,000 per month and owning more than 2 vehicles
- Number of families earning Rs. 13,000-16,000 and owning more than 2 vehicles = 25
- Probability (P(D)) = 25 / 2400
Calculation:
P(D)=252400
(v) Owning not more than 1 vehicle
- Families owning 0 vehicles: 10
- Families owning 1 vehicle: 160 + 305 + 535 + 469 + 579 = 2048
- Total families owning not more than 1 vehicle = 10 + 2048 = 2058
- Probability (P(E)) = 2058 / 2400
Calculation:
P(E)=20582400=10311200
(vi) Owning at least one vehicle
- Families owning at least 1 vehicle = Total families - Families owning 0 vehicles
- Families owning 0 vehicles = 10
- Families owning at least 1 vehicle = 2400 - 10 = 2390
- Probability (P(F)) = 2390 / 2400
Calculation:
P(F)=23902400=589600
Final Answers:
1. P(A)=292400
2. P(B)=5792400
3. P(C)=1240
4. P(D)=252400
5. P(E)=10311200
6. P(F)=589600
Total number of families surveyed = 2400
We need to analyze the data in the table, which categorizes families based on their income levels and the number of vehicles they own. We will extract the relevant figures for each scenario.
- Number of families earning Rs. 10,000-13,000 and owning exactly 2 vehicles = 29
- Probability (P(A)) = Number of favorable outcomes / Total outcomes = 29 / 2400
P(A)=292400
- Number of families earning Rs. 16,000 or more and owning exactly 1 vehicle = 579
- Probability (P(B)) = 579 / 2400
P(B)=5792400
- Number of families earning less than Rs. 7,000 and owning 0 vehicles = 10
- Probability (P(C)) = 10 / 2400
P(C)=102400=1240
- Number of families earning Rs. 13,000-16,000 and owning more than 2 vehicles = 25
- Probability (P(D)) = 25 / 2400
P(D)=252400
- Families owning 0 vehicles: 10
- Families owning 1 vehicle: 160 + 305 + 535 + 469 + 579 = 2048
- Total families owning not more than 1 vehicle = 10 + 2048 = 2058
- Probability (P(E)) = 2058 / 2400
P(E)=20582400=10311200
- Families owning at least 1 vehicle = Total families - Families owning 0 vehicles
- Families owning 0 vehicles = 10
- Families owning at least 1 vehicle = 2400 - 10 = 2390
- Probability (P(F)) = 2390 / 2400
P(F)=23902400=589600
1. P(A)=292400
2. P(B)=5792400
3. P(C)=1240
4. P(D)=252400
5. P(E)=10311200
6. P(F)=589600
Topper's Solved these Questions
Similar Questions
An organisation selected 2400 families at random and surveyed them to determine relationship between income level and the number of vechicles in a family. The information gathered is listed in the table below:

Suppose a family is chosen. Find the probability that the family chosen is :
(i) earning Rs. 10000-13000 per month and owning exactly 2 vehicles.
(ii) earning Rs. 16000 or more per month and owning exactly 1 vehicle.
(iii) earning less than Rs. 7000 per month and does not own any vehicle.
View SolutionA company selected 2400 families at random and survey them to determine a relationship between income level and the number of vehicles in a home. The information gathered is listed in the table below: Monthly income (in Rs.) Vehicles per family 0 1 2 Above 2 Less than 7000 10 160 25 0 7000-10000 0 305 27 2 10000-13000 1 535 29 1 13000-16000 2 469 29 25 16000 or more 1 579 82 88 If a family is chosen, find the probability that the family is: earning Rs. 10000-13000 per month and owning exactly 2 vehicles. earning Rs. 16000 or more per month and owning exactly 1 vehicles. Earning less than Rs. 7000 per month and does not own any vehicle. Earning Rs. 13000-16000 per month and owning more than 2 vehicle. owning not more than 1 vehicle owning at least one vehicle.
View SolutionA company selected 4000 households at random and surveyed them to find out a relationship between income level and the number of televisions sets in a home. The information, so obtained is listed in the following table.
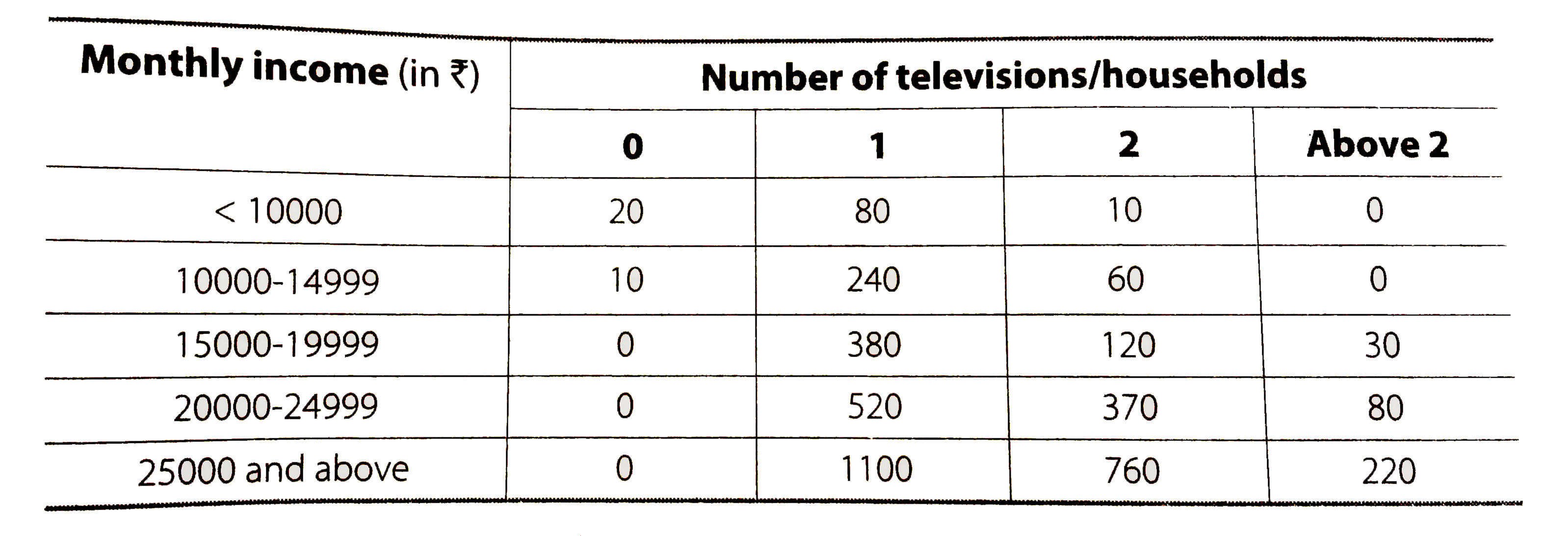
Find the probability
i) of a household earning Rs 10000- Rs 14999 per year and having exactly one television.
ii) of a household earning Rs 25000 and more per year owning 2 televisions.
iii) of a household not having any television.
View SolutionA mand earns Rs 75000 in 5 months
In hwo many months willhe earn Rs 45000
View SolutionIn a locality 100 families were chosen at random and the following data was collected:
∣∣ ∣ ∣∣Number of childeren 0 1 2 3 4 or moreis each familyNumber of families 3 40 45 12 0∣∣ ∣ ∣∣
If a family is chosen at random, find the probability that the chosen family has 3 or more children.
View SolutionThe ratio of the income to the expenditure of a family is 7:6. Find the saving if the income is Rs 1400.
View SolutionThe ratio of the income to the expenditure of a family is 7:6. Find the saving if the income is Rs 1400.
View SolutionThe probability that in a family of 5 members,exactly two members have birthday on sunday is:-
View SolutionThe probability that in a family of 5 members,exactly two members have birthday on sunday is:-
View SolutionLet 40% of employees earn more than Rs. 18000 per month. Then to find the percentage of employees earn less than or equal to Rs. 18000 you have to calculate which percentile?
View Solution
