A circle is inscribed in Δ PQR having sides 8 cm, 10 cm and 12 cm. A circle touches the sides PQ, QR and RP at D, E and F. Find PD
5 cm
6 cm
4 cm
10 cm
The correct Answer is:A
Step by step video, text & image solution for A circle is inscribed in Delta PQR having sides 8 cm, 10 cm and 12 cm. A circle touches the sides PQ, QR and RP at D, E and F. Find PD by Maths experts to help you in doubts & scoring excellent marks in Class 14 exams.
Related Playlists
Similar Questions
A circle is inscribed in Δ PQR having sides 8 cm, 10 cm and 12 cm. A circle touches the sides PQ, QR and RP at D, E and F. Find PD
View SolutionA circle in inscribed in a ΔABC having sides 8 cm , 10 cm and 12 cm in Fig Find AD, BE and CF.
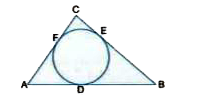 View Solution
View SolutionA circle is inscribed in a △ABC having sides 8 cm, 10 cm and 12 cm as shown in figure. Find AD, BE and CF.
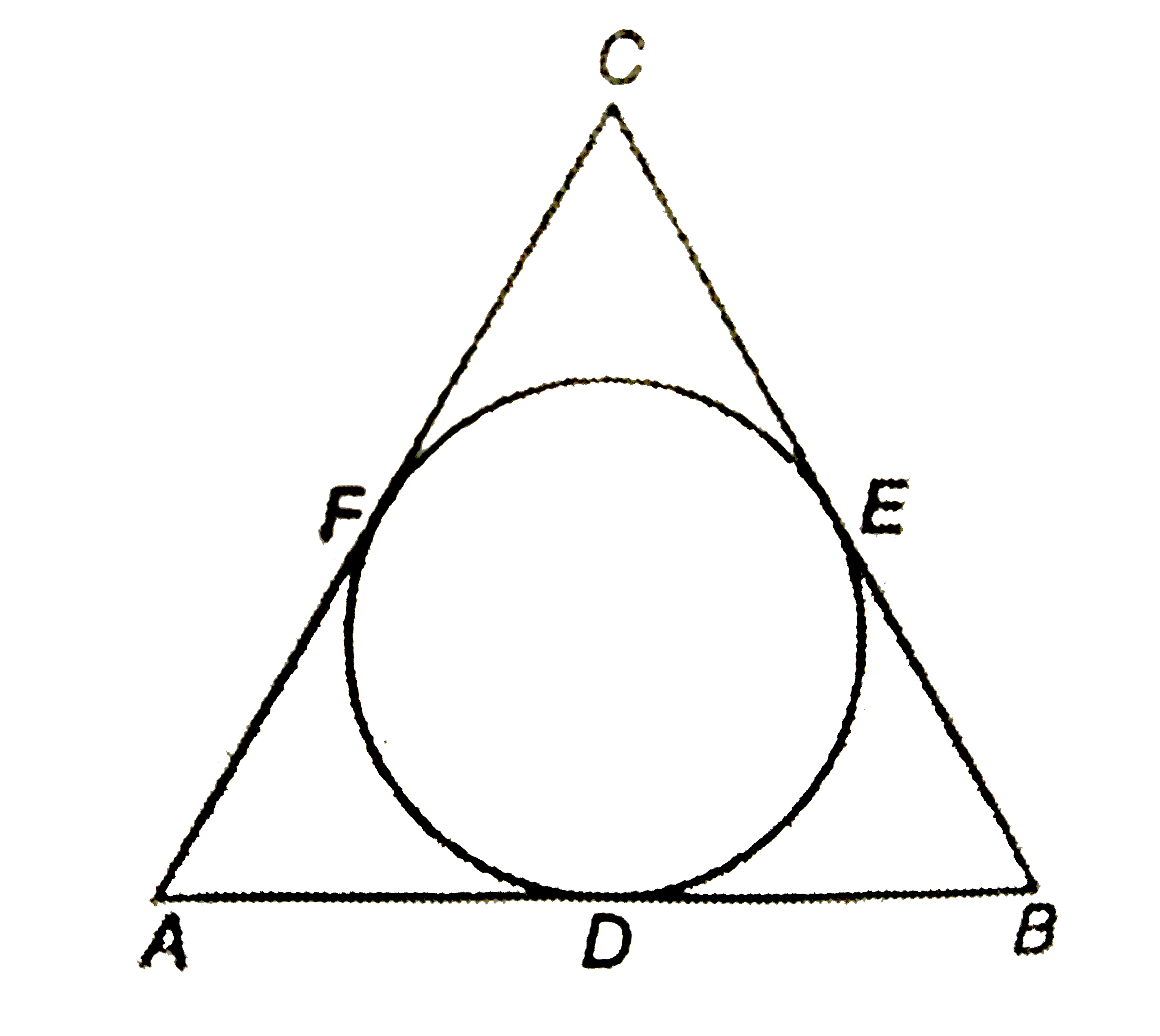 View Solution
View SolutionA circle is inscribed in △ABC having sides AB=8cm, BC=7cm and AC=5cm. Find AD, BE and CF.
View SolutionA circle is inscribed in a triangle PQR with PQ = 10 cm, QR = 8 cm and PR = 12 cm. Find the lengths QM, RN and PL.
View SolutionVarun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the

fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.
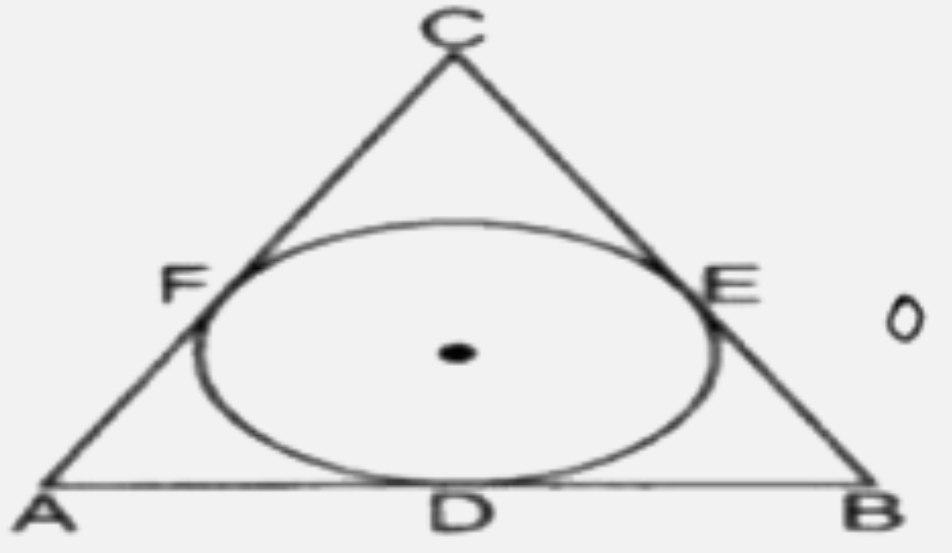
if radius of the circle is 4 cm , Find the area of ΔAOB.View SolutionIn fig , a circle isinscribed in a triangle PQR with PQ = 10 cm , QR = 8 cm and PR = 12 cm .Find the length of the QM , RN and PL.
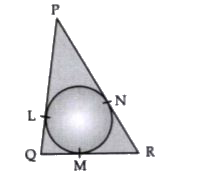 View Solution
View SolutionA circle C of radius 1 is inscribed in an equilateral triangle PQR. The points of contact of C with the sides PQ, QR, RP are D, E, F, respectively. The line PQ is given by the equation √3x+y−6=0 and the point D is (3 sqrt3/2, 3/2). Further, it is given that the origin and the centre of C are on the same side of the line PQ. The equation of circle C is
View SolutionThe sides of a triangle are 8 cm, 10 cm and 12 cm, find the greatest angle.
View SolutionThe area of circle inscribed in an equilateral triangle of side 12 cm is
View Solution


