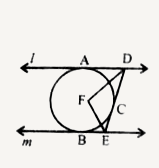
In a circle, PQ and RS are two parallel tangents at A and B. The tangent at makes an intercept DE between PQ and RS. Then ∠DOE is equal to : (where O is centre).
90∘
120∘
60∘
45∘
The correct Answer is:A
To solve the problem, we need to find the measure of angle DOE, where D and E are points on the tangent DE that intercepts between the two parallel tangents PQ and RS, and O is the center of the circle.
1. Identify the Elements:
- We have a circle with center O.
- There are two parallel tangents PQ and RS at points A and B respectively.
- The tangent DE intersects between the two parallel tangents.
2. Understanding Tangents:
- The radius of the circle is perpendicular to the tangent at the point of tangency. Therefore, OA ⊥ PQ and OB ⊥ RS.
3. Angles at the Center:
- Since OA and OB are radii of the circle, we know that angle AOB is 90 degrees because the tangents are parallel and the radius meets the tangent at a right angle.
4. Triangles Involved:
- Consider triangles OAD and OBE. Both triangles share the common radius (OA and OB) and the segments AD and BE are tangents from points A and B to points D and E respectively.
5. Congruent Triangles:
- By the property of tangents from a point to a circle being equal, we have AD = AM and BE = BM.
- Therefore, triangles OAD and OBE are congruent by the Side-Angle-Side (SAS) criterion.
6. Finding Angle DOE:
- Since triangles OAD and OBE are congruent, angle OAD = angle OBE.
- Also, since OA and OB are both perpendicular to the tangents at points A and B, we have angle OAD = angle OBE = 90 degrees.
7. Using Linear Pair:
- In triangle DOE, we know that angle AOB = 90 degrees. Therefore, angle DOE must also be 90 degrees, as it is formed by the tangents DE intersecting at points D and E.
8. Conclusion:
- Thus, the measure of angle DOE is 90 degrees.
Final Answer:
Angle DOE = 90 degrees.
- We have a circle with center O.
- There are two parallel tangents PQ and RS at points A and B respectively.
- The tangent DE intersects between the two parallel tangents.
- The radius of the circle is perpendicular to the tangent at the point of tangency. Therefore, OA ⊥ PQ and OB ⊥ RS.
- Since OA and OB are radii of the circle, we know that angle AOB is 90 degrees because the tangents are parallel and the radius meets the tangent at a right angle.
- Consider triangles OAD and OBE. Both triangles share the common radius (OA and OB) and the segments AD and BE are tangents from points A and B to points D and E respectively.
- By the property of tangents from a point to a circle being equal, we have AD = AM and BE = BM.
- Therefore, triangles OAD and OBE are congruent by the Side-Angle-Side (SAS) criterion.
- Since triangles OAD and OBE are congruent, angle OAD = angle OBE.
- Also, since OA and OB are both perpendicular to the tangents at points A and B, we have angle OAD = angle OBE = 90 degrees.
- In triangle DOE, we know that angle AOB = 90 degrees. Therefore, angle DOE must also be 90 degrees, as it is formed by the tangents DE intersecting at points D and E.
- Thus, the measure of angle DOE is 90 degrees.
Angle DOE = 90 degrees.
Topper's Solved these Questions
Similar Questions
Knowledge Check
PQ and RS are two parallel tangents to a circle with centre O and another tangent AB wih point of contact C intersect PQ at A and RS at B. then find ∠AOB
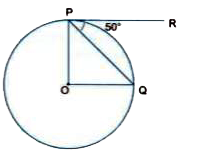
A60∘B120∘C90∘D180∘In figure, if O is the centre of a circle, PQ is a chord and the tangent PR at P makes an angle of 50∘ with PQ, then ∠POQ is equal to
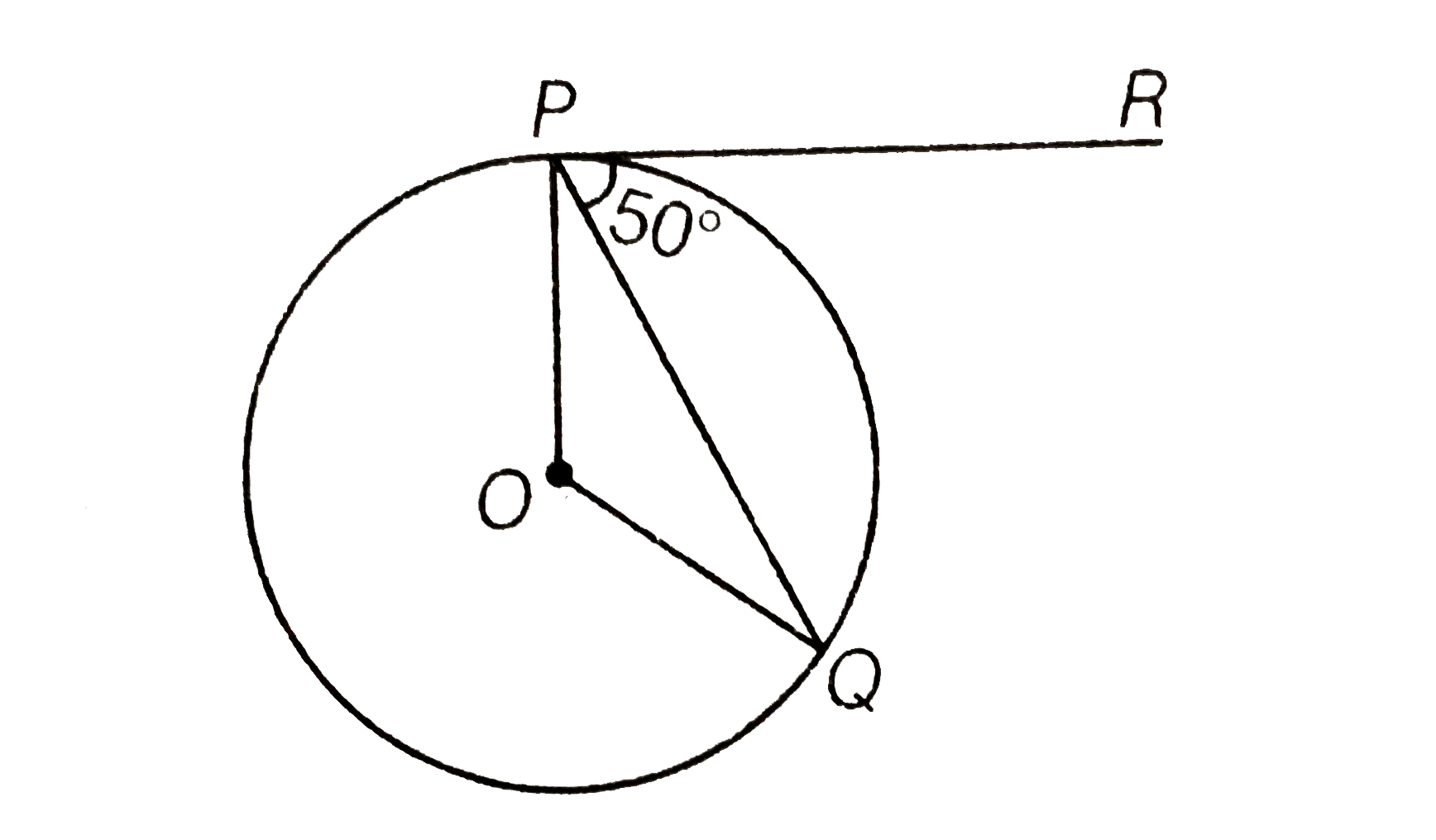 A100∘B80∘C90∘D75∘
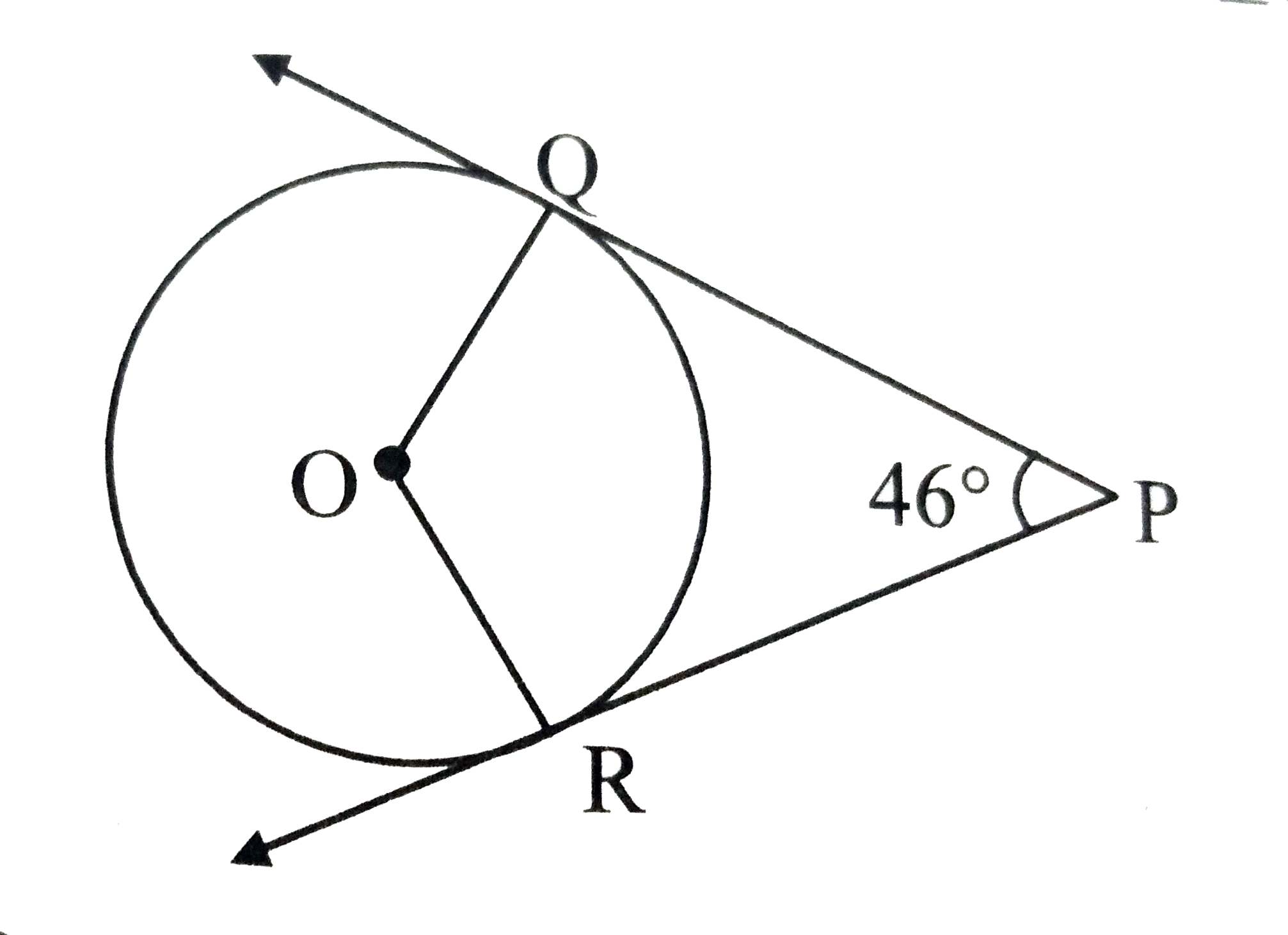
A100∘B80∘C90∘D75∘If PQ and PR are two tangents to ta circle with centre O. if ∠QPR=46∘, then ∠QOR is equal to
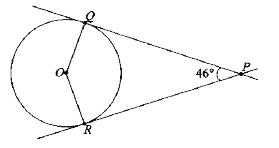 A67∘B46∘C44∘D134∘
A67∘B46∘C44∘D134∘
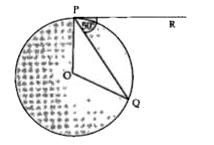
In the given figure ,'O is the centre of circle ,PQ is a chord and the tangent PR at P makes an angle of 50∘ with PQ ,then ∠POQ is equal to :
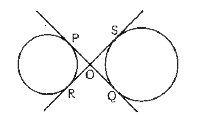
View SolutionIn the figure, PQ and RS are the common tangents to two circles intersecting at O.
Prove that PQ=RS
View SolutionIn Fig , O is the centre of a circle , PQ is a chord and the tangent PR at P makes an angle of 50∘ with PQ. Find ∠POQ .
View SolutionIn figure, PQ and PR are two tangents to a circle with centre O. IF ∠QPR=46∘ , then ∠QOR equals
View SolutionPQandRS are two parallel chords of a circle. If PQ=30 cm, RS=16 cm and distance between PQandRS is 23 cm, then find the radius of the circle.
View Solution