If 3−2x2>5x,x∈R , then the set of solutions is
A. (−∞,−12)
B. (3,∞)
C. (−12,3)
D. [−12,3]
The correct Answer is:C
To solve the inequality 3−2x2>5x, we will follow these steps:
Step 1: Rearranging the Inequality
Start by rearranging the inequality to one side:
3−2x2−5x>0
This can be rewritten as:
−2x2−5x+3>0
Step 2: Multiplying by -1
To make the leading coefficient positive, multiply the entire inequality by -1. Remember to reverse the inequality sign:
2x2+5x−3<0
Step 3: Factoring the Quadratic
Next, we need to factor the quadratic expression 2x2+5x−3. We can do this by finding two numbers that multiply to 2×−3=−6 and add to 5. These numbers are 6 and −1.
Now, we can rewrite 5x as 6x−x:
2x2+6x−x−3<0
Grouping the terms:
(2x2+6x)+(−x−3)<0
Factoring by grouping:
2x(x+3)−1(x+3)<0
Factoring out (x+3):
(2x−1)(x+3)<0
Step 4: Finding the Critical Points
Set each factor to zero to find the critical points:
1. 2x−1=0⇒x=12
2. x+3=0⇒x=−3
Step 5: Testing Intervals
Now we will test the intervals determined by the critical points x=−3 and x=12:
1. Interval 1: (−∞,−3)
- Choose x=−4: (2(−4)−1)(−4+3)=(−8−1)(−1)=9>0
2. Interval 2: (−3,12)
- Choose x=0: (2(0)−1)(0+3)=(−1)(3)=−3<0
3. Interval 3: (12,∞)
- Choose x=1: (2(1)−1)(1+3)=(2−1)(4)=4>0
Step 6: Writing the Solution
The inequality (2x−1)(x+3)<0 holds true in the interval:
(−3,12)
Final Solution
Thus, the set of solutions is:
x∈(−3,12)
Start by rearranging the inequality to one side:
3−2x2−5x>0
This can be rewritten as:
−2x2−5x+3>0
To make the leading coefficient positive, multiply the entire inequality by -1. Remember to reverse the inequality sign:
2x2+5x−3<0
Next, we need to factor the quadratic expression 2x2+5x−3. We can do this by finding two numbers that multiply to 2×−3=−6 and add to 5. These numbers are 6 and −1.
2x2+6x−x−3<0
Grouping the terms:
(2x2+6x)+(−x−3)<0
Factoring by grouping:
2x(x+3)−1(x+3)<0
Factoring out (x+3):
(2x−1)(x+3)<0
Set each factor to zero to find the critical points:
1. 2x−1=0⇒x=12
2. x+3=0⇒x=−3
Now we will test the intervals determined by the critical points x=−3 and x=12:
1. Interval 1: (−∞,−3)
- Choose x=−4: (2(−4)−1)(−4+3)=(−8−1)(−1)=9>0
2. Interval 2: (−3,12)
- Choose x=0: (2(0)−1)(0+3)=(−1)(3)=−3<0
3. Interval 3: (12,∞)
- Choose x=1: (2(1)−1)(1+3)=(2−1)(4)=4>0
The inequality (2x−1)(x+3)<0 holds true in the interval:
(−3,12)
Thus, the set of solutions is:
x∈(−3,12)
Topper's Solved these Questions
- LINEAR INEQUALITIES BOOK - ICSECHAPTER - LINEAR INEQUALITIES EXERCISE - MULTIPLE CHOICE QUESTION
16 Videos
- LIMITS AND DERIVATIVES BOOK - ICSECHAPTER - LIMITS AND DERIVATIVES EXERCISE - Multiple Choice Questions
31 Videos
- MATHEMATICAL INDUCTIONBOOK - ICSECHAPTER - MATHEMATICAL INDUCTIONEXERCISE - Exercise (a)
1 Videos
Similar Questions
Knowledge Check
If −5<2x−3≤x+2,x∈R, then the solution set of x on the number line is
A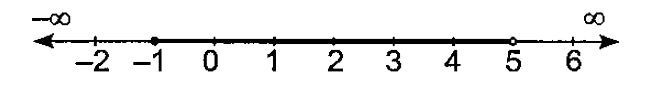 B
B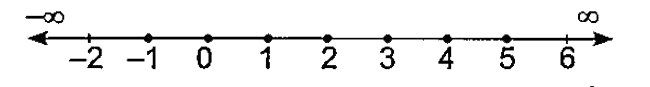 C
C D
D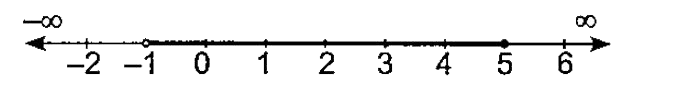
If x2−5≤x3−4,x∈R, then the solution set of x on the number line is:
A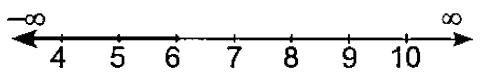 B
B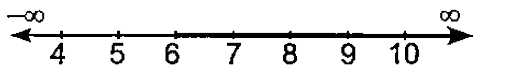 C
C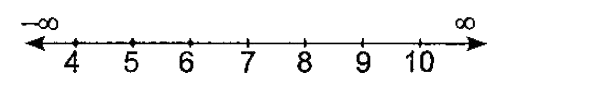 D
D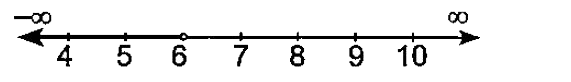
If 2≤2x−3≤5,x∈I, then the solution set of x on the number line is:
A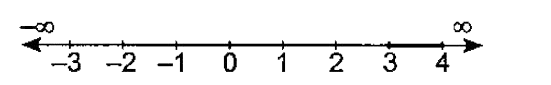 B
B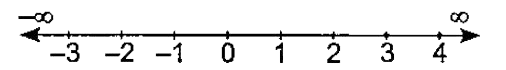 C
C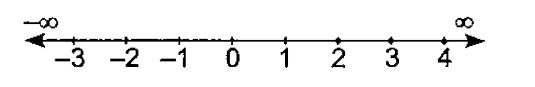 D
D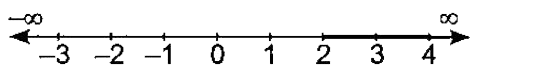
Solve the inequation 2x+5>−5x+12 If x ∈ R and represent the solution on the number line
View SolutionIf 2x−3<x+1<4x+7,x∈R then the solution set of x on the number line is:
View SolutionIf 2x−5≤5x+4<29 and x is an integer, then the solution set of x is
View SolutionAssertion: The solution set of 2x−3≤x+1≤4x+7,x∈N is {1, 2, 3,4} .
Reason: If the solution of a linear inequation is x>2,x∈R then on the number line, it is represented as
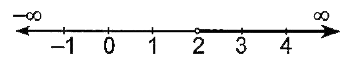
View SolutionThe solution set of 2x+13<3x−25x∈R on the number line is
View Solution
