If x is a negative integar , then the solution set of −12x>30 is
A. {−2,−1}
B. {…,−5,−4,−3}
C. {…,−5,−4,−3,−2}
D. {−2,−1,0,1,2…}
The correct Answer is:B
To solve the inequality −12x>30 given that x is a negative integer, we will follow these steps:
Step 1: Rewrite the Inequality
We start with the inequality:
−12x>30
Step 2: Divide by -12
To isolate x, we divide both sides of the inequality by −12. Remember, when we divide or multiply both sides of an inequality by a negative number, the direction of the inequality sign flips:
x<30−12
Step 3: Simplify the Right Side
Now, we simplify 30−12:
30−12=−3012=−52
So, we have:
x<−52
Step 4: Convert to Decimal
Next, we can convert −52 to a decimal:
−52=−2.5
Thus, we have:
x<−2.5
Step 5: Identify Negative Integers
Since x must be a negative integer, we look for integers that are less than −2.5. The negative integers less than −2.5 are:
−3,−4,−5,…
Step 6: Write the Solution Set
The solution set of negative integers satisfying the inequality is:
{−3,−4,−5,…} or (−∞,−3)
Conclusion
Thus, the correct answer is option B: −∞,−5,−4,−3.
---
We start with the inequality:
−12x>30
To isolate x, we divide both sides of the inequality by −12. Remember, when we divide or multiply both sides of an inequality by a negative number, the direction of the inequality sign flips:
x<30−12
Now, we simplify 30−12:
30−12=−3012=−52
So, we have:
x<−52
Next, we can convert −52 to a decimal:
−52=−2.5
Thus, we have:
x<−2.5
Since x must be a negative integer, we look for integers that are less than −2.5. The negative integers less than −2.5 are:
−3,−4,−5,…
The solution set of negative integers satisfying the inequality is:
{−3,−4,−5,…} or (−∞,−3)
Thus, the correct answer is option B: −∞,−5,−4,−3.
Topper's Solved these Questions
- LINEAR INEQUALITIES BOOK - ICSECHAPTER - LINEAR INEQUALITIES EXERCISE - MULTIPLE CHOICE QUESTION
16 Videos
- LIMITS AND DERIVATIVES BOOK - ICSECHAPTER - LIMITS AND DERIVATIVES EXERCISE - Multiple Choice Questions
31 Videos
- MATHEMATICAL INDUCTIONBOOK - ICSECHAPTER - MATHEMATICAL INDUCTIONEXERCISE - Exercise (a)
1 Video
Similar Questions
Knowledge Check
The solution set of |x−1|x>2 is
A0<x<13Bx<13Cx>13D13<x<1How many positive integers are there in the solution set of xx−2>5 ?
A0B2C4D5If x∈w, then the solution set of the inequation −x>−7, is:
A{8,9, 10 …}B{0, 1, 2, 3, 4,5,6}C{0, 1, 2, 3 …}D{-8, -9,- 10...}
If x is non -negative integer, solve the inequaton 2−3x<5−4x. Also show the solution set on number line
View SolutionIf the replacement set is the set of whole numbers, then the solution set of 12−x≤3x−2 is
View SolutionIf
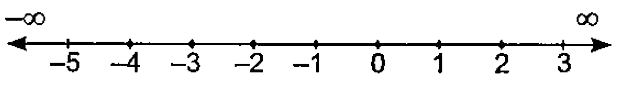 is the solution set of a linear in equation in x, then the solution set of x in set-builder form is:
is the solution set of a linear in equation in x, then the solution set of x in set-builder form is:
View SolutionIf
 is the solution set of a linear inequation in x, then the solution set of x in set-builder form is:
is the solution set of a linear inequation in x, then the solution set of x in set-builder form is:
View SolutionWhat are the solutions to 3x2+12x+6=0 ?
View Solution
