The solution set of system of linear inequalities 2(x+1)≤x+5,3(x+2)>2−x,x∈R is
[−1,3]
(−1,3)
(−1,3]
[−1,3)
The correct Answer is:C
To solve the system of linear inequalities given by:
1. 2(x+1)≤x+5
2. 3(x+2)>2−x
We will solve each inequality step by step.
Step 1: Solve the first inequality
Inequality:
2(x+1)≤x+5
Expanding the left side:
2x+2≤x+5
Rearranging the terms:
Subtract x from both sides:
2x−x+2≤5
This simplifies to:
x+2≤5
Subtracting 2 from both sides:
x≤3
Step 2: Solve the second inequality
Inequality:
3(x+2)>2−x
Expanding the left side:
3x+6>2−x
Rearranging the terms:
Add x to both sides:
3x+x+6>2
This simplifies to:
4x+6>2
Subtracting 6 from both sides:
4x>2−6
This simplifies to:
4x>−4
Dividing by 4:
x>−1
Step 3: Combine the results
From the first inequality, we have:
x≤3
From the second inequality, we have:
x>−1
Final Solution Set
Combining these results, we find that:
−1<x≤3
This can be represented in interval notation as:
(−1,3)
2. 3(x+2)>2−x
2(x+1)≤x+5
2x+2≤x+5
Subtract x from both sides:
2x−x+2≤5
This simplifies to:
x+2≤5
x≤3
3(x+2)>2−x
3x+6>2−x
Add x to both sides:
3x+x+6>2
This simplifies to:
4x+6>2
4x>2−6
This simplifies to:
4x>−4
x>−1
x≤3
x>−1
−1<x≤3
(−1,3)
Topper's Solved these Questions
- LINEAR INEQUALITIES Book:ICSEChapter:LINEAR INEQUALITIES Exercise:MULTIPLE CHOICE QUESTION
Explore 16 Videos
- LIMITS AND DERIVATIVES Book:ICSEChapter:LIMITS AND DERIVATIVES Exercise:Multiple Choice Questions
Explore 31 Videos
- MATHEMATICAL INDUCTIONBook:ICSEChapter:MATHEMATICAL INDUCTIONExercise:Exercise (a)
Explore 1 Video
Similar Questions
Knowledge Check
The solution set of the quadratic inequality 3−2x2≤5x is
A(a) (−∞,−3]B(b) [12,∞)C(c) [−3,12]D(d) (−∞,−3]∪[12,∞)The solution set for the linear inequation −8≤x−7<−4,x∈I is:
A{x:x∈R,−1≤x<3}B{0,1,2,3}C{−1,0,1,2,3}D{−1,0,1,2}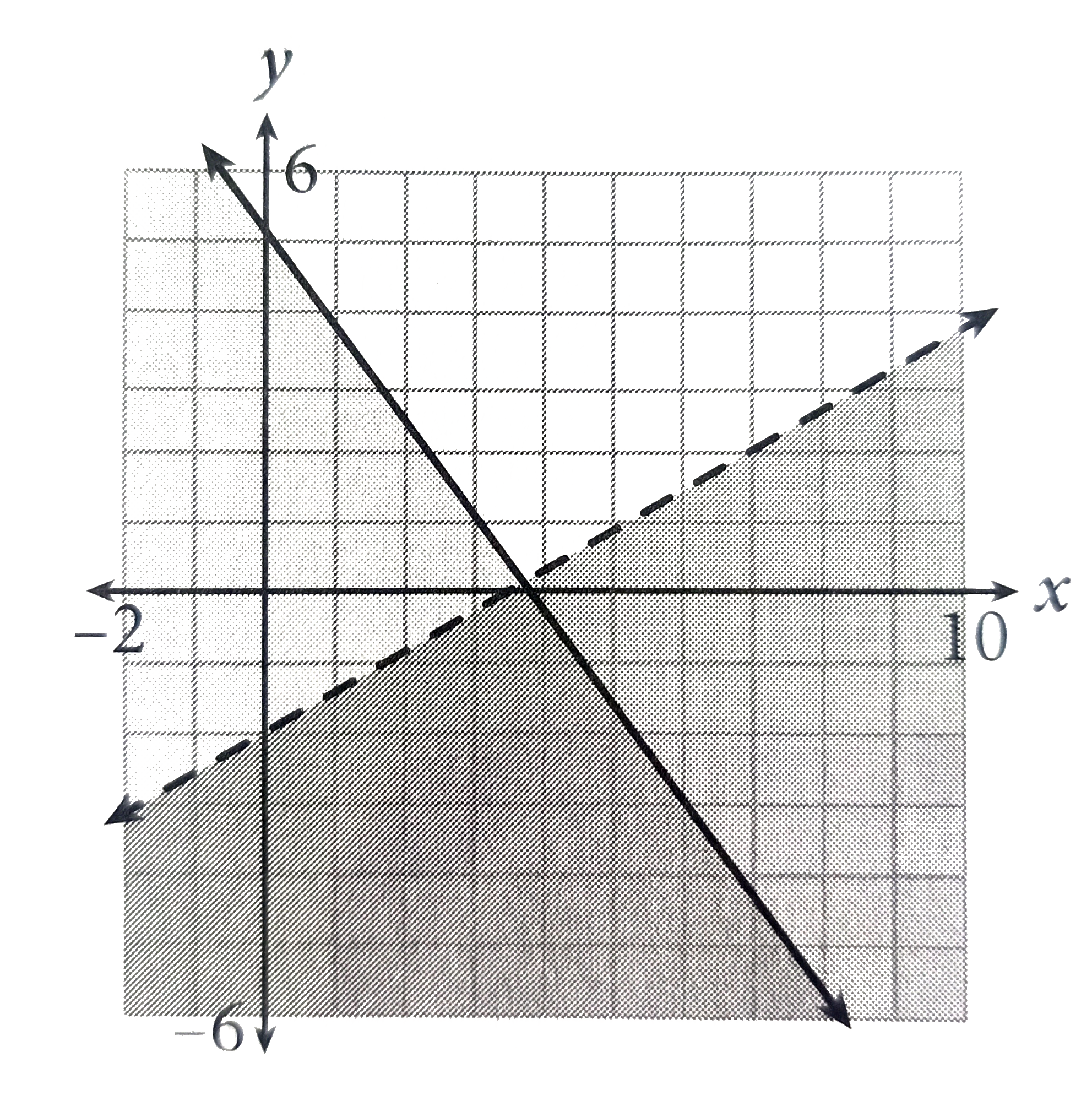
The figure above shows the solution set for this system of inequalities:
⎧⎨⎩x<35x−2y≤−43x+5
Which of the following is NOT is solution to this system ?A(−1,−4)B(1,−1)C(4,−1)D(6,−3)
The solution set of the inequation x−1x−2>2, is
View SolutionThe solution set of the inequation x+4x−3≤2, is
View SolutionThe solution set of the inequation ∣∣∣3x+1∣∣∣>2, is
View SolutionThe solution set of the inequation |2x−3|<|x+2|, is
View SolutionThe solution set of the inequality max {1−x2,|x−1|}<1 then
View Solution