Question

Obtain an expression for the force between two straight parallel conductor carrying current. Hence define ampere.

Explanation

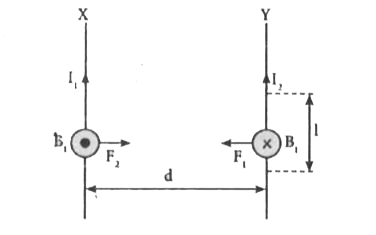
In the above figure .
X and Y are two infinitely long straight parallel conductors, `I_1` and `I_2` are the currents in the X and Y respectively and .d. is the perpendicular distance between the conductors .
Magnetic field due to the current `I_1` is
`B_1=(mu_0I_1)/(2pid) to` (1)
According to right hand clasp rule , the direction of `B_1` is perpendicular to the plane of the paper and directed inwards. The conductor Y lies in the magnetic field `B_1` and hence it experiences force.
The force on a segment .I. of the conductor Y is given by
`F_1 =B_1I_2 I sin theta " " (because F=BH sin theta)`
`F_1=B_1 I_2 I to (2) " " (because theta=90^@ " then sin" 90^@ =1)`
(1) in (2) `rArr F_1=(mu_0I_1I_2)/(2pid)`
similarly `F_2=(mu_0I_1I_2I)/(2pid)`
According to fleming.s left hand rule , `F_1` is towards the conductors X and `F_2` is towards the conductor Y i.e., X and Y are attracting each other . When the currents are in the opposite direction , X and Y repels each other. `F_1` and `F_2` are the mutual forces. From Newton.s third law `F_1=-F_2`
Therefore , the force of attraction or the force of repulsion is given by
`F=(mu_0I_1I_2I)/(2pid)`
Definition of Ampere : Ampere is the current which flows through two infinitely long straight parallel conductors separated by a distance 1 metre, placed in free space, causes a force of `2xx10^(-7) Nm^(-1)` between them.