Question

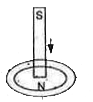
A small magnet $M$ is allowed to fall through a fixed horizontal conducting ring $R$. Let $g$ be the acceleration due to gravity. The acceleration of $M$ will be

Options

A. `lt` g when it is above R and moving towards R
B. `gt` g when it is above R and moving towards R
C. `lt` g when it is below R and moving away from R
D. `gt` g when it is below R and moving away from R

Answer

A::C

Explanation

To solve the problem of the small magnet $M$ falling through a fixed horizontal conducting ring $R$, we need to analyze the situation using the principles of electromagnetic induction and Newton's laws of motion.

1. Understanding the Setup:
- We have a small magnet `M` with a north pole and a south pole.
- The magnet is allowed to fall through a horizontal conducting ring `R`.
- The gravitational acceleration is denoted as `g`.

2. Initial Motion of the Magnet:
- When the magnet is released, it begins to fall under the influence of gravity, initially accelerating downwards with an acceleration of `g`.

3. Induction of EMF:
- As the magnet falls, it moves through the conducting ring, which causes a change in magnetic flux through the ring.
- According to Faraday's law of electromagnetic induction, a change in magnetic flux induces an electromotive force (EMF) in the ring.

4. Direction of Induced Current:
- The induced EMF generates a current in the ring. By Lenz's law, the direction of this induced current will be such that it opposes the change in magnetic flux that produced it.
- If the magnet is falling (moving towards the ring), the flux through the ring is increasing, and the induced current will create a magnetic field that opposes the magnet's motion (acting upwards).

5. Effect on the Magnet's Acceleration:
- The upward magnetic force due to the induced current opposes the downward force of gravity.
- Therefore, the net acceleration of the magnet `M` will be less than `g` because the upward force reduces the effective gravitational force acting on the magnet.

6. Case When the Magnet Moves Away:
- If the magnet moves away from the ring, the magnetic flux through the ring decreases.
- The induced current will again act to oppose this change, which means it will create a magnetic field that attracts the magnet back towards the ring.
- Thus, in this case as well, the acceleration of the magnet will still be less than `g` because the induced current is acting upwards.

7. Conclusion:
- In both scenarios (whether the magnet is moving towards or away from the ring), the acceleration of the magnet `M` will always be less than `g`.

Final Answer:
The acceleration of the magnet `M` will be less than `g`.

---