A wire of length l in the form of a square loop lies in a plane normal to a magnetic field B0. If this wire is converted into a circular loop in time, then find the average induced emf.
To find the average induced emf when a wire of length L in the form of a square loop is converted into a circular loop, we follow these steps:
Step 1: Determine the Area of the Square Loop
The wire forms a square loop, and since the length of the wire is L, each side of the square loop will be:
Side length of square=L4
The area A1 of the square loop is given by:
A1=(L4)2=L216
Step 2: Calculate the Magnetic Flux through the Square Loop
The magnetic flux Φ1 through the square loop in a magnetic field B0 is:
Φ1=B0⋅A1=B0⋅L216
Step 3: Determine the Radius of the Circular Loop
When the wire is reshaped into a circular loop, the circumference of the circular loop must equal the length of the wire:
2πR=L⟹R=L2π
Step 4: Calculate the Area of the Circular Loop
The area A2 of the circular loop is:
A2=πR2=π(L2π)2=π⋅L24π2=L24π
Step 5: Calculate the Magnetic Flux through the Circular Loop
The magnetic flux Φ2 through the circular loop is:
Φ2=B0⋅A2=B0⋅L24π
Step 6: Calculate the Change in Magnetic Flux
The change in magnetic flux ΔΦ as the loop transforms from a square to a circular shape is:
ΔΦ=Φ2−Φ1=(B0⋅L24π)−(B0⋅L216)
Factoring out B0:
ΔΦ=B0(L24π−L216)
Step 7: Simplify the Change in Flux
To simplify the change in flux:
ΔΦ=B0L2(416π−116)=B0L2(4−π16π)
Step 8: Calculate the Average Induced EMF
The average induced emf E is given by Faraday's law of electromagnetic induction:
E=−ΔΦΔt
Assuming the transformation occurs over a time t0:
E=−B0L2(4−π16π)t0
Thus, the average induced emf is:
E=B0L2(4−π)16πt0
Final Answer
The average induced emf when the wire is converted from a square loop to a circular loop is:
E=B0L2(4−π)16πt0
The wire forms a square loop, and since the length of the wire is L, each side of the square loop will be:
Side length of square=L4
The area A1 of the square loop is given by:
A1=(L4)2=L216
The magnetic flux Φ1 through the square loop in a magnetic field B0 is:
Φ1=B0⋅A1=B0⋅L216
When the wire is reshaped into a circular loop, the circumference of the circular loop must equal the length of the wire:
2πR=L⟹R=L2π
The area A2 of the circular loop is:
A2=πR2=π(L2π)2=π⋅L24π2=L24π
The magnetic flux Φ2 through the circular loop is:
Φ2=B0⋅A2=B0⋅L24π
The change in magnetic flux ΔΦ as the loop transforms from a square to a circular shape is:
ΔΦ=Φ2−Φ1=(B0⋅L24π)−(B0⋅L216)
Factoring out B0:
ΔΦ=B0(L24π−L216)
To simplify the change in flux:
ΔΦ=B0L2(416π−116)=B0L2(4−π16π)
The average induced emf E is given by Faraday's law of electromagnetic induction:
E=−ΔΦΔt
Assuming the transformation occurs over a time t0:
E=−B0L2(4−π16π)t0
Thus, the average induced emf is:
E=B0L2(4−π)16πt0
The average induced emf when the wire is converted from a square loop to a circular loop is:
E=B0L2(4−π)16πt0
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Check point
60 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Taking it together
119 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Level 2 Subjective
20 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
A wire in the form of a circular loop of radius 10cm lies in a plane normal to a magnetic field of 100T. If this wire is pulled to take a square shape in the same plane in 0.1s, find the average induced emf in the loop.
View SolutionA long wire carrying a steady current is bent into a circular loop of one turn. The magnetic field at the centre of the loop is B. it is then bent into a circular coil of n turns. The magnetic field at the centre of this coil of n turns will be
View SolutionA wire of length l is formed into a circular loop of one turn only and is suspended in a magnetic field B. When a current i is passed through the loop, the maximum torque experienced by it is
View SolutionOne of the two identical conducting wires of length L is bent in the form of a circular loop and the other one into a circular coil of N identical turns. If the same current passed in both, the ratio of the magnetic field at the central of the loop (BL) to that at the central of the coil (BC), i.e. BLBC will be :
View SolutionB0 is the magnetic field at point P for a given straight wire as shown in figure. If this wirre is bent into a circular loop, then magnetic field at the centre of loop is
_PHY_E06_130_Q01.png)
View SolutionA conducting square loop having edges of length2.0 cm is rotated through 180∘ about a diagonal in 0.20s. A magnetic field B exists in the region which si perpendicular to the loop in its initial position. If the average induced emf during the rotation is 20 mV, find the magnitude of the magnetic field.
View SolutionA circular loop is placed in magnetic field B=2t. Find the direction of induced current produced in the loop.
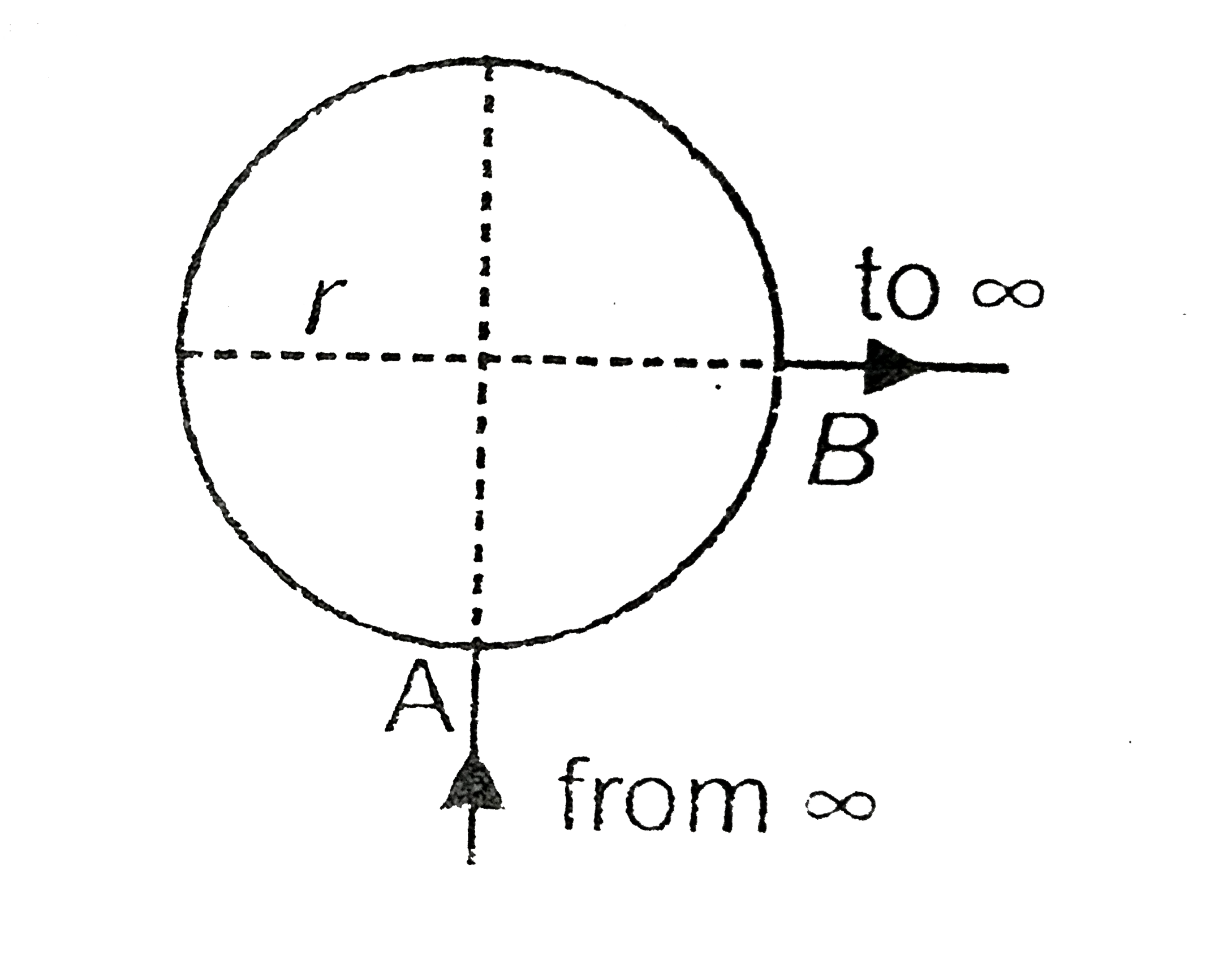
View SolutionIn the figure, the current l enters the circular loop of uniform wire of radius r at A and leaves it at B. The magnetic field of the centre of circular loop is
View SolutionA given wire is bent into a rectangular loop of size 15cm×5cm and placed perpendicular to a magnetic field of 1.0 Tesla. Within 0.5sec, the loop is changed into a 10cm square and the field increases to 1.4 Tesla.Calculate the value of e.m.f. induced in the loop?
View SolutionB0 is the magnetic field at point P fir s given straight wire as shown in figure. If this wire is bent into a circular loop,then magnetic field at the center of the loop is
_PHY_E06_051_Q01.png)
View Solution
