A horizontally placed spring mass system has time period T. The same system is now placed on a car moving with acceleration a in horizontal direction. Then,
time period will increase
time period will decrease
time period will remain constant
no conclusion can be drawn
The correct Answer is:C
To solve the problem, we need to analyze how the time period of a spring-mass system is affected when it is placed in a car that is accelerating horizontally.
1. Understand the Time Period of a Spring-Mass System:
The time period T of a simple harmonic oscillator (spring-mass system) is given by the formula:
T=2π√mk
where m is the mass of the block and k is the spring constant.
2. Identify the Forces Acting on the Mass:
In a spring-mass system, the restoring force acting on the mass when it is displaced from its equilibrium position is given by Hooke's Law:
F=−kx
where x is the displacement from the equilibrium position.
3. Consider the Effect of Acceleration:
When the spring-mass system is placed in a car that is accelerating with acceleration a, we need to consider how this affects the system. The acceleration of the car does not change the spring constant k or the mass m of the block.
4. Analyze the System in the Accelerating Frame:
In the accelerating frame of the car, the effective force acting on the mass can be thought of as being influenced by the pseudo force due to the acceleration of the car. However, this does not alter the restoring force provided by the spring.
5. Conclude on the Time Period:
Since the time period T depends only on the mass m and the spring constant k, and neither of these quantities changes when the system is placed in an accelerating car, we can conclude that the time period remains unchanged.
Therefore, the new time period T′ when the system is in the accelerating car is:
T′=T
Final Answer:
The time period remains constant when the spring-mass system is placed in a car moving with acceleration a.
The time period T of a simple harmonic oscillator (spring-mass system) is given by the formula:
T=2π√mk
where m is the mass of the block and k is the spring constant.
In a spring-mass system, the restoring force acting on the mass when it is displaced from its equilibrium position is given by Hooke's Law:
F=−kx
where x is the displacement from the equilibrium position.
When the spring-mass system is placed in a car that is accelerating with acceleration a, we need to consider how this affects the system. The acceleration of the car does not change the spring constant k or the mass m of the block.
In the accelerating frame of the car, the effective force acting on the mass can be thought of as being influenced by the pseudo force due to the acceleration of the car. However, this does not alter the restoring force provided by the spring.
Since the time period T depends only on the mass m and the spring constant k, and neither of these quantities changes when the system is placed in an accelerating car, we can conclude that the time period remains unchanged.
T′=T
The time period remains constant when the spring-mass system is placed in a car moving with acceleration a.
Similar Questions
Time period of a given spring-mass system is

View SolutionA block of mass m is placed inside a smooth hollow cylinder of radius R kept horizontally. Initially system was at rest. Now cylinder is given constant acceleration 2g in the horizontal direction by external agent. Find the maximum angular displacement of the block with the vertical.
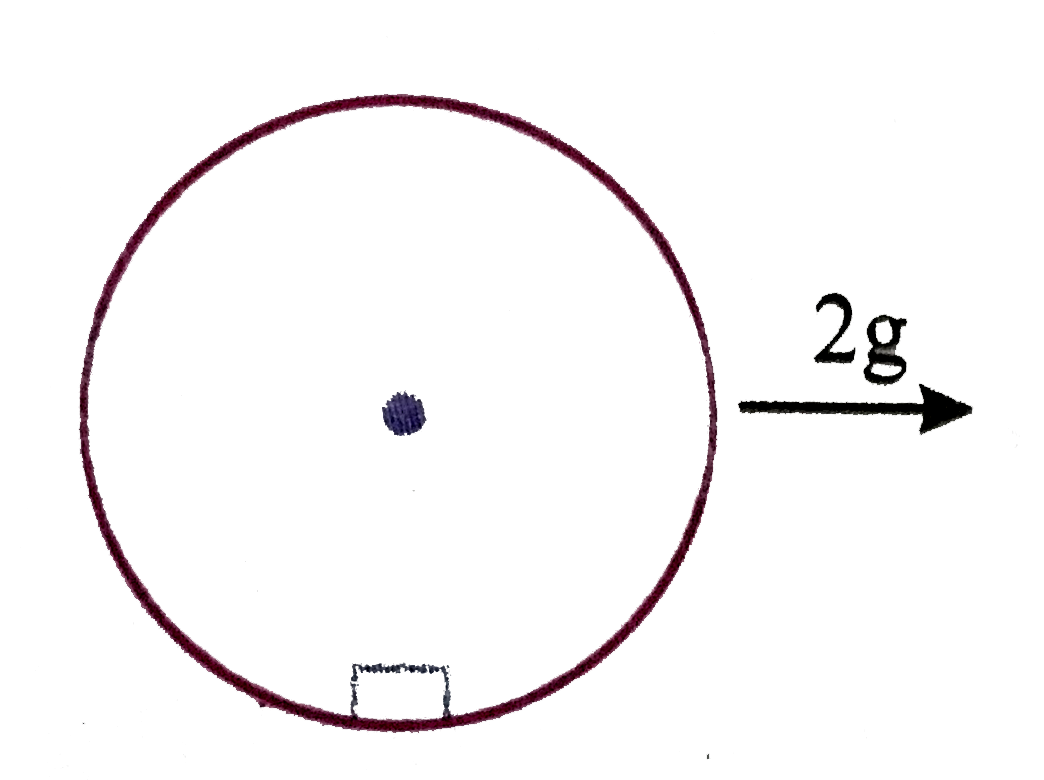 .
.
View SolutionA simple pendulum with a bob of mass m is suspended from the roof of a car moving with horizontal acceleration a
View SolutionA spring mass system oscillates in a car. If the car accelerates on a horizontal road, the frequency of oscillation will
View SolutionA spring mass system has time period of 2 second. What should be the spring constant of spring if the mass of the block is 10grams ?
View SolutionA horizontal spring mass system is executing SHM with time period of 4s. At time t=0, it is at mean position. Find the minimum time after which its potential energy becomes three times of kinetic energy.
View SolutionA force of 72 dyne is inclined to the horizontal at an angle of 60∘. Find the acceleration in a mass of 9 g, which moves in a horizontal direction.
View SolutionA dip needle vibrates in a vertical plane with time period of 3 second . The same needle is suspended horizontally and made to vibrate is a horizontal plane . The time period is again 3 sec. The angle of dip at that place is .
View SolutionTwo blocks each having mass m is attached to each other with a spring having force constant k. The system is placed on a rough horizontal surface having coefficient of friction μ. The maximum initial compression, that can be given to the spring so that when released the blocks do not move is
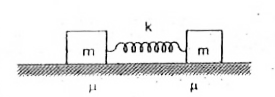
View SolutionToy cart tied to the end of an unstretched string of length a, when revolved moves in a horizontal circle of radius 2a with a time period T. Now the toy cart is speeded up until it moves in a horizontal circle of radius 3a with a period T. If Hooke's law (F=kx) holds, then
View Solution
