A force of 72 dyne is inclined to the horizontal at an angle of 60∘. Find the acceleration in a mass of 9 g, which moves in a horizontal direction.
5cms−1
4cms−1
2ms−1
3ms−1
The correct Answer is:B
To solve the problem step by step, we will follow these instructions:
Step 1: Identify the Given Values
- Force (F) = 72 dyne
- Angle (θ) = 60 degrees
- Mass (m) = 9 g
Step 2: Resolve the Force into Components
The force can be resolved into two components:
- Horizontal component (F_x) = F * cos(θ)
- Vertical component (F_y) = F * sin(θ)
For our case:
- F_x = 72 dyne * cos(60°)
- F_y = 72 dyne * sin(60°)
Step 3: Calculate the Horizontal Component of the Force
Using the cosine value:
- cos(60°) = 1/2
Thus,
- F_x = 72 dyne * (1/2) = 36 dyne
Step 4: Apply Newton's Second Law
According to Newton's second law, the net force acting on an object is equal to the mass of the object multiplied by its acceleration (F = ma). Since we are interested in the horizontal motion:
- F_x = m * a
Step 5: Convert Mass to Appropriate Units
We need to convert the mass from grams to kilograms for standard SI units. However, since we are using dyne (where 1 dyne = 1 g·cm/s²), we can keep it in grams.
- m = 9 g
Step 6: Rearrange the Equation to Solve for Acceleration (a)
From the equation F_x = m * a, we can rearrange it to find acceleration:
- a = F_x / m
Step 7: Substitute the Values
Now substitute the values we have:
- a = 36 dyne / 9 g
Step 8: Calculate the Acceleration
- a = 4 cm/s²
Final Answer
Thus, the acceleration of the mass is 4 cm/s².
---
- Force (F) = 72 dyne
- Angle (θ) = 60 degrees
- Mass (m) = 9 g
The force can be resolved into two components:
- Horizontal component (F_x) = F * cos(θ)
- Vertical component (F_y) = F * sin(θ)
- F_x = 72 dyne * cos(60°)
- F_y = 72 dyne * sin(60°)
Using the cosine value:
- cos(60°) = 1/2
- F_x = 72 dyne * (1/2) = 36 dyne
According to Newton's second law, the net force acting on an object is equal to the mass of the object multiplied by its acceleration (F = ma). Since we are interested in the horizontal motion:
- F_x = m * a
We need to convert the mass from grams to kilograms for standard SI units. However, since we are using dyne (where 1 dyne = 1 g·cm/s²), we can keep it in grams.
- m = 9 g
From the equation F_x = m * a, we can rearrange it to find acceleration:
- a = F_x / m
Now substitute the values we have:
- a = 36 dyne / 9 g
- a = 4 cm/s²
Thus, the acceleration of the mass is 4 cm/s².
Topper's Solved these Questions
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Check point 5.2
Explore 15 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Check point 5.3
Explore 20 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Subjective Question
Explore 27 Videos
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:INTEGER_TYPE
Explore 15 Videos
- LAWS OF THERMODYNAMICSBook:DC PANDEY ENGLISHChapter:LAWS OF THERMODYNAMICSExercise:Level 2 Subjective
Explore 18 Videos
Similar Questions
A force of 10 N inclined to the horizontal at an angle of 60∘ acts on a body of mass 2 kg. If the body can move in horizontal direction only find its acceleration.
View SolutionA force of 4N is inclined at an angle of 60∘ from the vertical. Find out its components along horizontal and vertical directions.

View SolutionA force of 4N is inclined at an angle of 60∘ from the vertical. Find out its components along horizontal and vertical directions.

View Solution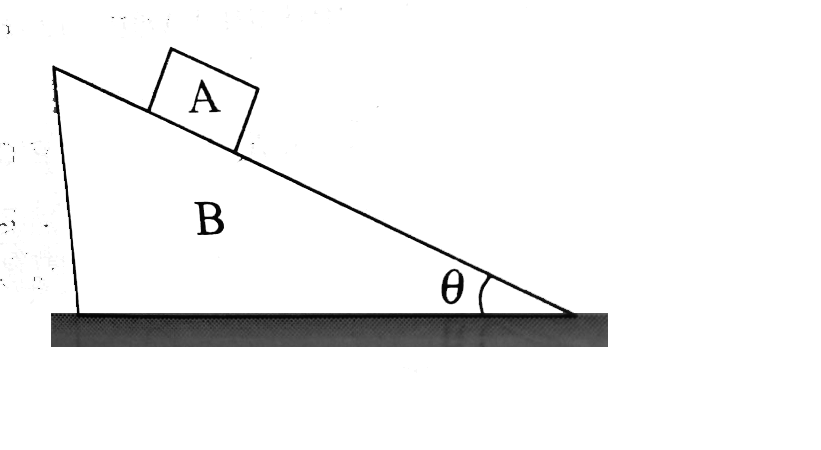
A block is placed on a smooth wedge of inclination angle θ with the horizontal. What minimum acceleration should be given to the wedge in the horizontal direction so that the block starts moving up along the incline?
View SolutionA body is projected with a velocity u at an angle of 60∘ to the horizontal. The time interval after which it will be moving in a direction of 30∘ to the horizontal is
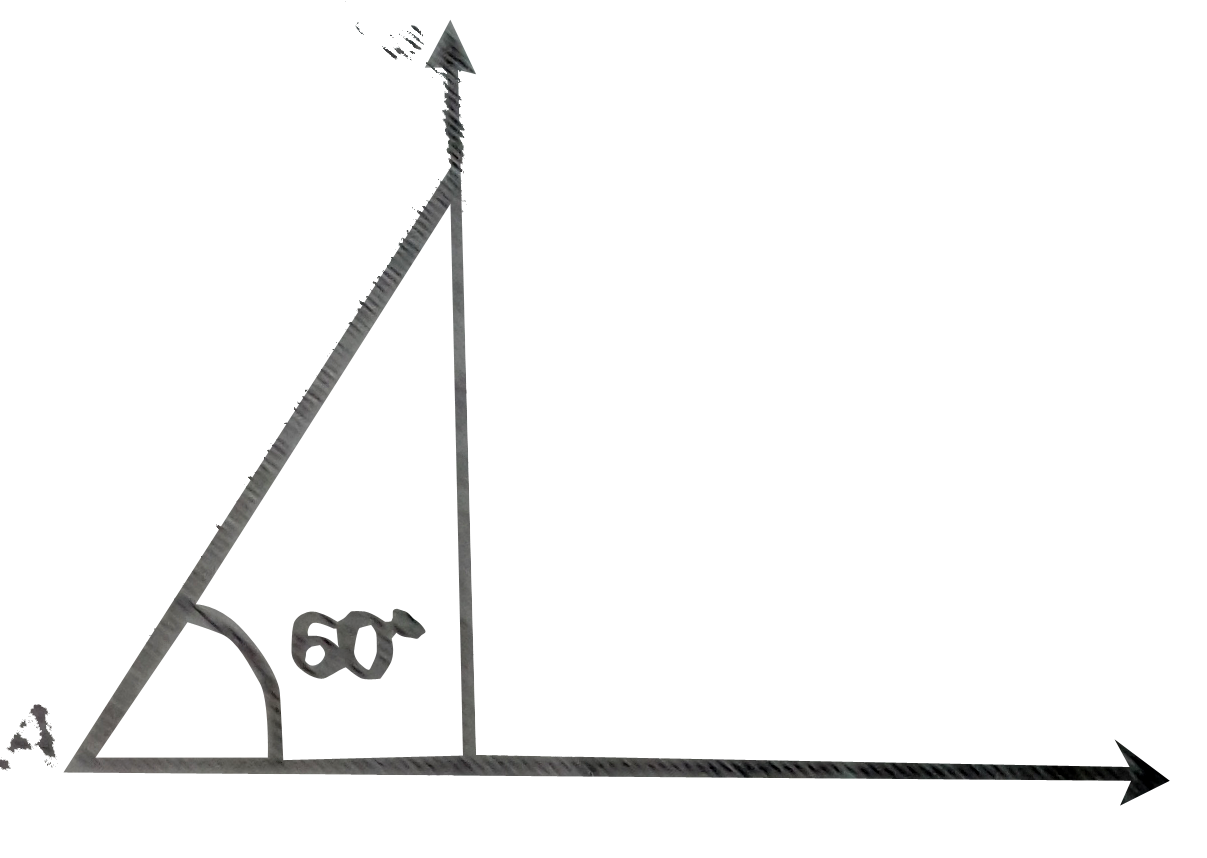
View SolutionA link AB is moving in a vertical plane. At a certain instant when the link is inclined 60∘ to the horizontal the point A is moving horizontaly at 3m/s, while B is moving in the vertical direction. What is the velocity of B?
View SolutionA force is inclined at 60∘ to the horizontal. If its rectangular component in the horizontal direction be 50N,find the magnitude of the force and its vertical component.
View Solution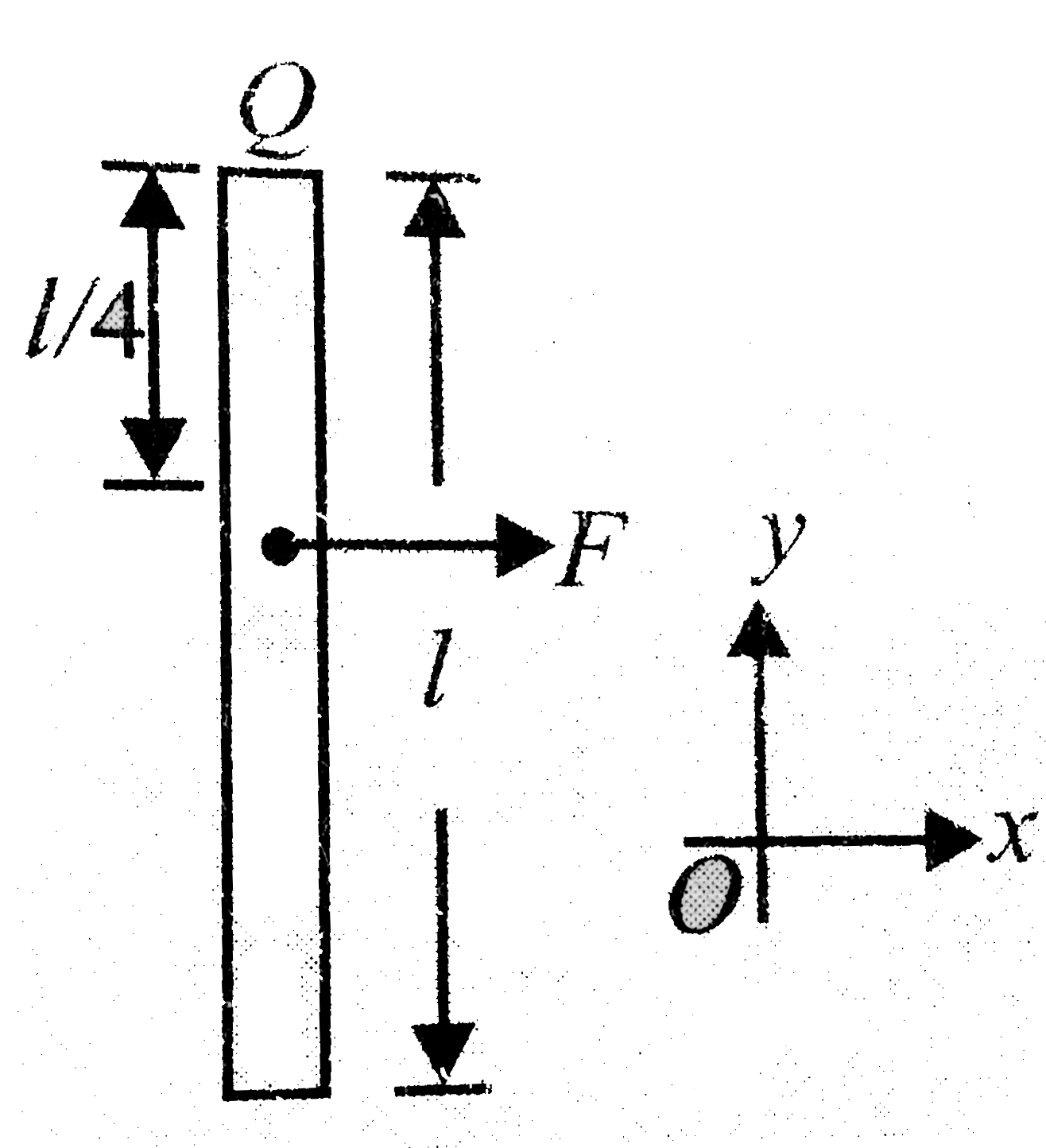 Find α,aQ and the point of zero acceleration when the horizontal force F acts on the smooth rod of mass m and length l which is kept on a horizontal surface.
Find α,aQ and the point of zero acceleration when the horizontal force F acts on the smooth rod of mass m and length l which is kept on a horizontal surface.
View SolutionA force is inclined at an angle of 60∘ from the horizontal. If the horizontal component of the force is 40N. Calculate the vertical component.
View SolutionA force is inclined at an angle of 60∘ from the horizontal. If the horizontal component of the force is 4N,calculate the vertical component.
View Solution
