A rectangular block of mass m and area of cross-section A floats in a liquid of density ρ. If it is given a small vertical displacement from equilibrium, it undergoes oscillation with a time period T. Then
T∝m
T∝ρ
T∝1A
T∝√1ρ
The correct Answer is:D
To solve the problem of a rectangular block floating in a liquid and undergoing oscillation when displaced, we can follow these steps:
Step 1: Understand the Forces Acting on the Block
When the block is floating in equilibrium, the weight of the block (mg) is balanced by the buoyant force (Fb). The buoyant force is given by the formula:
Fb=ρ⋅Vd⋅g
where:
- ρ = density of the liquid
- Vd = volume of the displaced liquid
- g = acceleration due to gravity
Step 2: Relate the Volume Displaced to the Block's Dimensions
For a rectangular block with an area of cross-section A and height submerged h:
Vd=A⋅h
Thus, the buoyant force can be expressed as:
Fb=ρ⋅(A⋅h)⋅g
Step 3: Set Up the Equilibrium Condition
At equilibrium, the weight of the block is equal to the buoyant force:
mg=ρ⋅(A⋅h)⋅g
From this, we can derive:
h=mρ⋅A
Step 4: Analyze the Displacement from Equilibrium
When the block is displaced by a small distance y downwards, the new height submerged becomes h+y. The new buoyant force is:
F′b=ρ⋅(A⋅(h+y))⋅g
Step 5: Calculate the Net Force
The net force acting on the block when displaced is:
Fnet=F′b−mg
Substituting the expressions for F′b and mg:
Fnet=ρ⋅(A⋅(h+y))⋅g−mg
Fnet=ρ⋅(A⋅h)⋅g+ρ⋅(A⋅y)⋅g−mg
Using the equilibrium condition mg=ρ⋅(A⋅h)⋅g:
Fnet=ρ⋅(A⋅y)⋅g
Step 6: Determine the Nature of the Motion
The net force can be expressed as:
Fnet=ρ⋅A⋅g⋅y
This indicates that the net force is proportional to the displacement y and acts in the opposite direction, satisfying the condition for simple harmonic motion (SHM).
Step 7: Find the Time Period of Oscillation
The time period T of oscillation for SHM is given by:
T∝√mk
where k is the effective spring constant. From our expression for Fnet, we can identify:
k=ρ⋅A⋅g
Thus, the time period can be expressed as:
T∝√mρ⋅A⋅g
Conclusion
The time period T is proportional to the square root of the mass m and inversely proportional to the square root of the product of the area A and the density ρ:
T∝√mρ⋅A
When the block is floating in equilibrium, the weight of the block (mg) is balanced by the buoyant force (Fb). The buoyant force is given by the formula:
Fb=ρ⋅Vd⋅g
where:
- ρ = density of the liquid
- Vd = volume of the displaced liquid
- g = acceleration due to gravity
For a rectangular block with an area of cross-section A and height submerged h:
Vd=A⋅h
Thus, the buoyant force can be expressed as:
Fb=ρ⋅(A⋅h)⋅g
At equilibrium, the weight of the block is equal to the buoyant force:
mg=ρ⋅(A⋅h)⋅g
From this, we can derive:
h=mρ⋅A
When the block is displaced by a small distance y downwards, the new height submerged becomes h+y. The new buoyant force is:
F′b=ρ⋅(A⋅(h+y))⋅g
The net force acting on the block when displaced is:
Fnet=F′b−mg
Substituting the expressions for F′b and mg:
Fnet=ρ⋅(A⋅(h+y))⋅g−mg
Fnet=ρ⋅(A⋅h)⋅g+ρ⋅(A⋅y)⋅g−mg
Using the equilibrium condition mg=ρ⋅(A⋅h)⋅g:
Fnet=ρ⋅(A⋅y)⋅g
The net force can be expressed as:
Fnet=ρ⋅A⋅g⋅y
This indicates that the net force is proportional to the displacement y and acts in the opposite direction, satisfying the condition for simple harmonic motion (SHM).
The time period T of oscillation for SHM is given by:
T∝√mk
where k is the effective spring constant. From our expression for Fnet, we can identify:
k=ρ⋅A⋅g
Thus, the time period can be expressed as:
T∝√mρ⋅A⋅g
The time period T is proportional to the square root of the mass m and inversely proportional to the square root of the product of the area A and the density ρ:
T∝√mρ⋅A
Similar Questions
A block of rectangular size of mass m and area of cross section A, float in a liquid of density ρ.If we give a small vertical displacement from equilibrium, It undergoes SHM with time period T, then
View SolutionA plank of mass 'm' and area of cross - section A is floating as shown in figure. When slightly displaced from mean position, plank starts oscillations. Find time period of these oscillations.

View SolutionA plank of mass 'm' and area of cross - section A is floating in a non - viscous liquid of desity ρ. When displaced slightly from the mean position, it starts oscillating. Prove that oscillations are simple harmonic and find its time period.
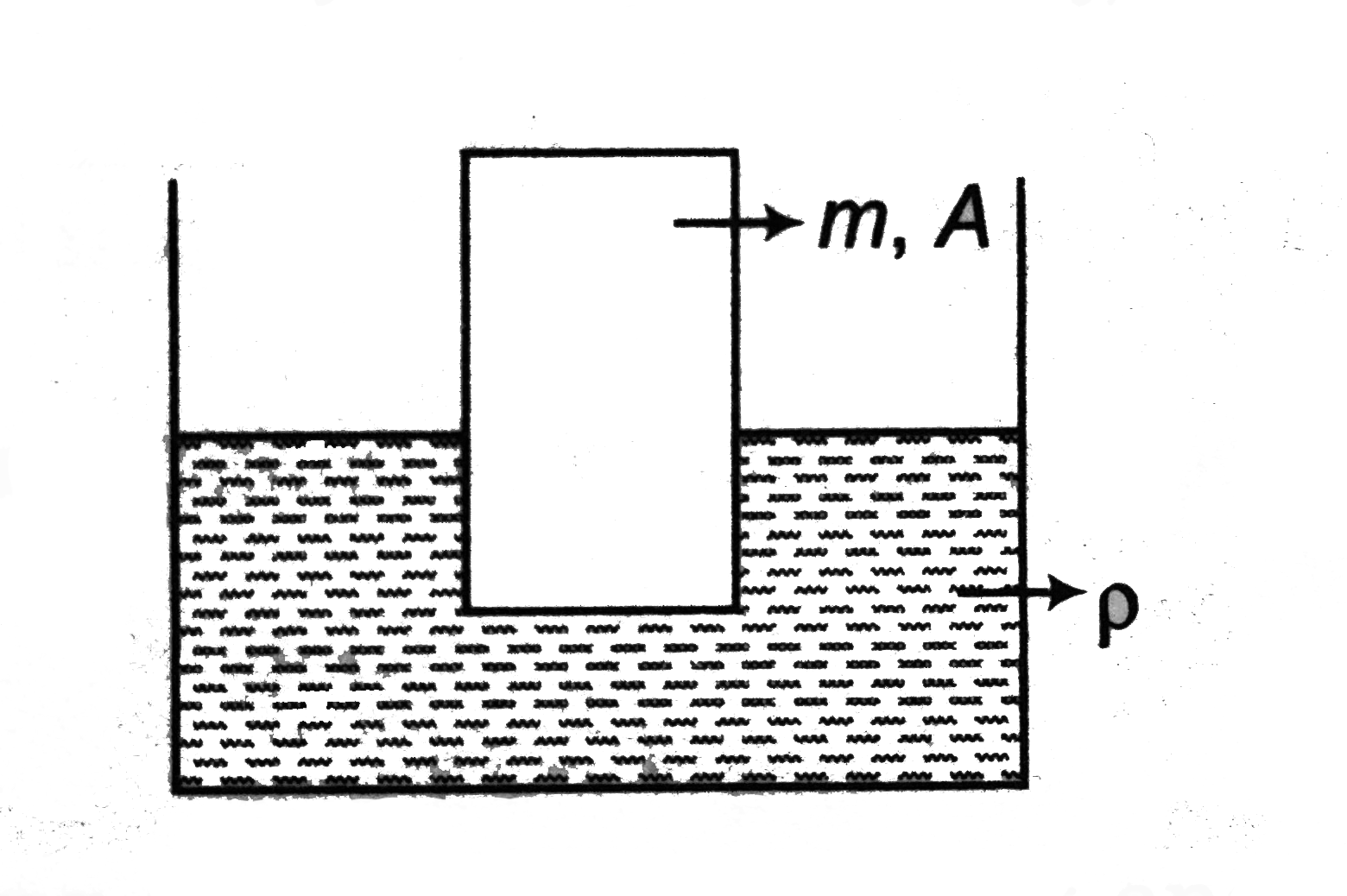
View SolutionA cylindrical block of wood of mass m and area cross-section A is floating in water (density = ρ) when its axis vertical. When pressed a little and the released the block starts oscillating. The period oscillations is
View SolutionA body of uniform cross-sectional area floats in a liquid of density thrice its value. The portion of exposed height will be :
View SolutionA small ring of mass m1 is connected by a string of length l to a small heavy bob of mass m2. The ring os free to move (slide) along a fixed smooth horizontal wire. The bob is given a small displacement from its equilibrium position at right angles to string. Find period of small oscillations.
_E01_106_Q01.png)
View Solution
A thin-walled tube of mass m and radius R has a rod of mass m and vry small cross section soldered on its inner surface. The side-view of the arrangement is as shown in the following figure. The entire arrangement is placed on a rough horizontal surface. The system is given a small angular displacement from its equilibrium position, as a result, the system performs oscillations. The time period of resulting oscillations if the tube rolls without slipping is
View SolutionPassage XIV) A uniform cylindrical block of mass 2M and cross-sectional area A remains partially submerged in a non viscous liquid of density ρ, density of the material of the cylinder is 3ρ. The cylinder is connected to lower end of the tank by means of a light spring of spring constant K. The other end of the cylinder is connected to anotehr block of mass M by means of a light inextensible sting as shown in the figure. The pulleys shown are massless and frictionless and assume that the cross-section of the cylinder is very small in comparison to that of the tank. Under equilibrium conditions, half of the cylinder is submerged. [given that cylinder always remains partially immersed)
Under equilibrium conditions
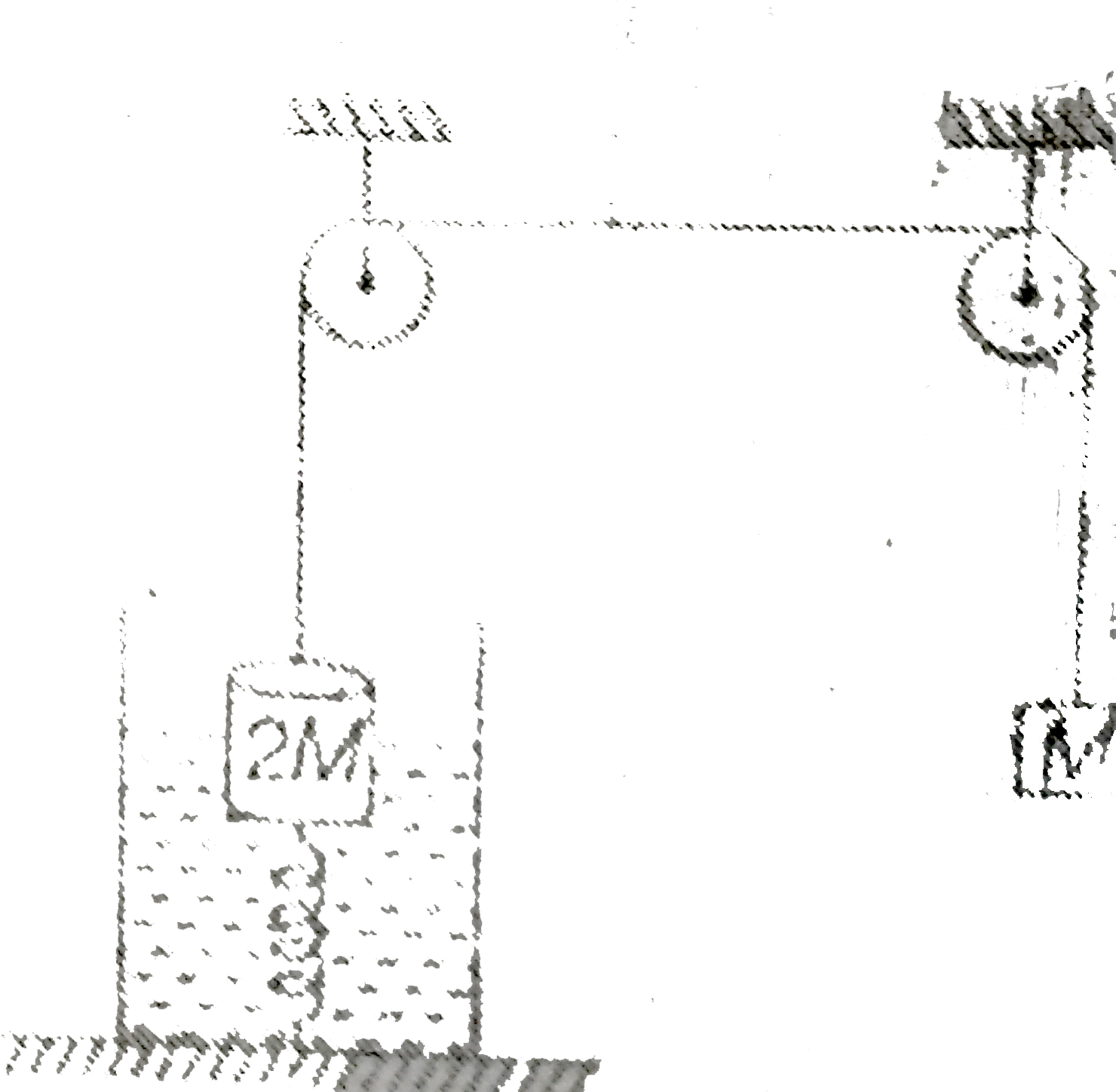
View SolutionPassage XIV) A uniform cylindrical block of mass 2M and cross-sectional area A remains partially submerged in a non viscous liquid of density ρ, density of the material of the cylinder is 3ρ. The cylinder is connected to lower end of the tank by means of a light spring of spring constant K. The other end of the cylinder is connected to anotehr block of mass M by means of a light inextensible sting as shown in the figure. The pulleys shown are massless and frictionless and assume that the cross-section of the cylinder is very small in comparison to that of the tank. Under equilibrium conditions, half of the cylinder is submerged. [given that cylinder always remains partially immersed)

By what maximum distance cylinder will be pushed downward into the liquid from equilibrium position so that when it is set free then tension in the string will not vanish [Assume at equilibrium position system was at rest]
View SolutionA vertical pole of length l , density ρ , area of cross section A floats in two immiscible liquids of densities ρ1and ρ2. In equuilibrium possition the bottom end is at the interface of the liquids. When the cylinder is displaced vertically, find the time period of oscillation.
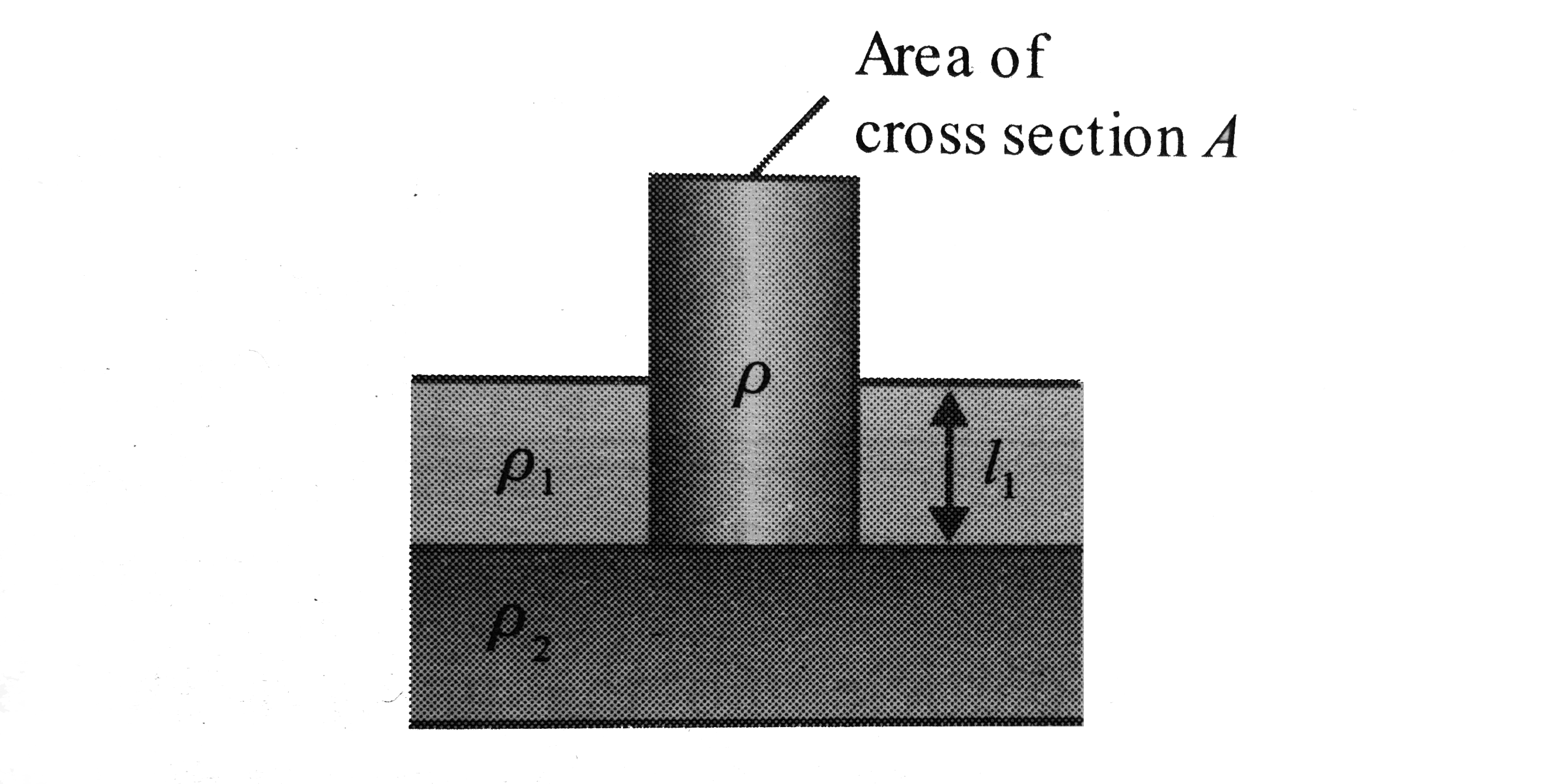
View Solution
