A train has a constant speed of 40 ms−1 on a level road against resistive force of magnitude 3×104N. Find the power of the engine.
To solve the problem, we need to find the power of the engine of the train moving at a constant speed against a resistive force. Here’s a step-by-step solution:
Step 1: Identify the given values
- Speed of the train (v) = 40 m/s
- Resistive force (R) = 3 × 10^4 N
Step 2: Understand the concept of power
Power (P) is defined as the rate at which work is done or energy is transferred. It can also be calculated using the formula:
P=F⋅v
where:
- P is the power,
- F is the force applied,
- v is the velocity of the object.
Step 3: Determine the force exerted by the engine
Since the train is moving at a constant speed, the force exerted by the engine (F) must equal the resistive force (R) acting against it. Therefore:
F=R=3×104N
Step 4: Calculate the power of the engine
Now, we can substitute the values into the power formula:
P=F⋅v
P=(3×104N)⋅(40m/s)
Step 5: Perform the multiplication
Calculating the power:
P=3×104×40
P=1.2×106W
Final Answer
The power of the engine is 1.2×106W or 1.2 MW.
---
- Speed of the train (v) = 40 m/s
- Resistive force (R) = 3 × 10^4 N
Power (P) is defined as the rate at which work is done or energy is transferred. It can also be calculated using the formula:
P=F⋅v
where:
- P is the power,
- F is the force applied,
- v is the velocity of the object.
Since the train is moving at a constant speed, the force exerted by the engine (F) must equal the resistive force (R) acting against it. Therefore:
F=R=3×104N
Now, we can substitute the values into the power formula:
P=F⋅v
P=(3×104N)⋅(40m/s)
Calculating the power:
P=3×104×40
P=1.2×106W
The power of the engine is 1.2×106W or 1.2 MW.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.1
20 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.2
20 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
A train travels from Agra to Delhi with a constant speed of 50kmh−1 and returns from Delhi to Agra with a constant speed of 40kmh−1 . Find the average speed of the train.
View SolutionAn elevator that can carry a maximum load of 1500 kg (elvator+ passengers) is moving up with a constant speed of 2 ms−1. The frictional force opposing the motion is 3000 N. Find the minmum power delvered by the motor to the elevator in watts as well as in horse power (g=10ms−2)
View SolutionA car travels with constant speed on a circular road level ground in figure Fair is the force of air resistence acts opposite to the motion of car which of the other forces shown best represents the horizontal force of the road on the car's tires?
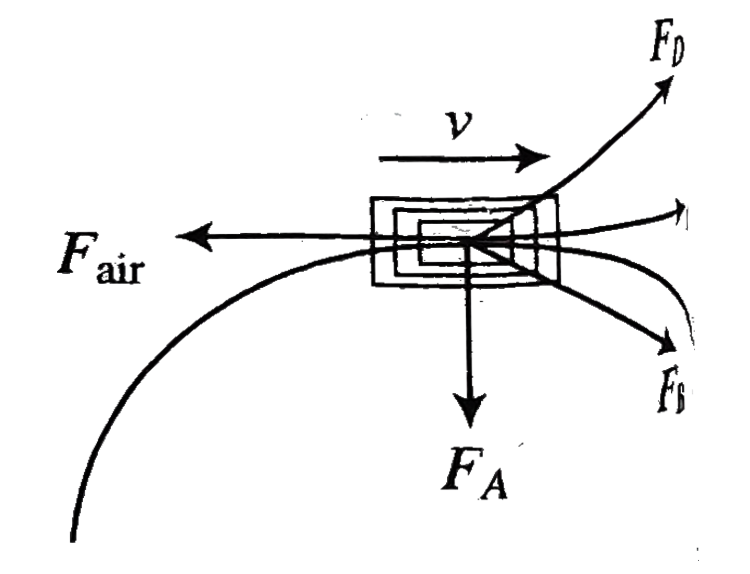
View SolutionA train of mass 2×105 kg has a constant speed of 20 ms−1 up a hill inclined at θ=sin−1(150) to the horizontal when the engine is working at 8×105 W. Find the resistance to motion of the trian. (Take ,g=9.8 ms−2).
View SolutionAn electron experiences a magnetic force of magnitude4.60×10−15N, when moving at an angle of 60∘ with respect to a magnetic field of magnitude 3.50×10−3T. Find the speed of the electron.
View SolutionA car travelling at a speed of 30ms−1 due north along the highway makes a left turn on to a sied road which heads towards due west. It takes 40s for the car to complere the turn. At the end of 40s, the caar has a speed of 20ms−1 along the side road. Derermime the magnitude of average acceleration over the 40second interval.
View SolutionAn ox can a maximum force of 1000 N.It is taking part in a cart race and is able to pull the cart at a constant speed of 30 ms−1 while making its best effort.Calculate the power developed by the ox.
View SolutionAn object of mass 10kg moves at a constant speed of 10 ms−1. A constant force, that acts for 4 sec on the object, gives it a speed of 2ms−1 in opposite direction. The force acting on the object is :
View SolutionAn automobile engine propels a 1000 kg car (A) along a levelled road at a speed of 36 km//h. Find the power if the opposing frictional force is 100 N Now, suppose after travelling a distance of 200 m, this car collides with another stationary car (B) of same mass and comes to rest. Let its engine also stop at the same time. Now car (B) starts moving on the same level road without getting its engine started. Find the speed of the car (B) just after the collision.
View SolutionA car travels with constant speed on a circular road on level ground. In the figure shown, Fair is the force of air resistance on the car. Which of the other forces best represent the horizontal force of the road on the car's tires ?

View Solution
