A train of mass 2×105 kg has a constant speed of 20 ms−1 up a hill inclined at θ=sin−1(150) to the horizontal when the engine is working at 8×105 W. Find the resistance to motion of the trian. (Take ,g=9.8 ms−2).
To solve the problem step-by-step, we will follow these steps:
Step 1: Identify the given data
- Mass of the train, m=2×105kg
- Speed of the train, v=20m/s
- Power of the engine, P=8×105W
- Angle of incline, θ=sin−1(150)
- Acceleration due to gravity, g=9.8m/s2
Step 2: Calculate the angle θ
Using the sine value:
sinθ=150
Step 3: Calculate the force exerted by the engine
Using the formula for power:
P=F⋅v
We can rearrange this to find the force F:
F=Pv=8×105W20m/s=4×104N
Step 4: Calculate the gravitational force components
The gravitational force acting on the train is mg:
mg=(2×105kg)×(9.8m/s2)=1.96×106N
Now, we need to calculate the component of this force acting down the incline:
mgsinθ=mg⋅150=1.96×10650=39200N
Step 5: Set up the equation for forces
Since the train is moving at a constant speed, the net force acting on it is zero. Therefore, the force exerted by the engine F must balance the gravitational force component and the resistance R:
F=mgsinθ+R
Substituting the values we have:
4×104=39200+R
Step 6: Solve for resistance R
Rearranging the equation to find R:
R=4×104−39200=800N
Final Answer
The resistance to motion of the train is R=800N.
---
- Mass of the train, m=2×105kg
- Speed of the train, v=20m/s
- Power of the engine, P=8×105W
- Angle of incline, θ=sin−1(150)
- Acceleration due to gravity, g=9.8m/s2
Using the sine value:
sinθ=150
Using the formula for power:
P=F⋅v
We can rearrange this to find the force F:
F=Pv=8×105W20m/s=4×104N
The gravitational force acting on the train is mg:
mg=(2×105kg)×(9.8m/s2)=1.96×106N
mgsinθ=mg⋅150=1.96×10650=39200N
Since the train is moving at a constant speed, the net force acting on it is zero. Therefore, the force exerted by the engine F must balance the gravitational force component and the resistance R:
F=mgsinθ+R
Substituting the values we have:
4×104=39200+R
Rearranging the equation to find R:
R=4×104−39200=800N
The resistance to motion of the train is R=800N.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.1
20 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - CHECK POINT 6.2
20 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, POWER AND ENERGYBOOK - DC PANDEY ENGLISHCHAPTER - WORK, POWER AND ENERGYEXERCISE - E Integer Type Questions
11 Videos
Similar Questions
A train has a constant speed of 40 ms−1 on a level road against resistive force of magnitude 3×104N. Find the power of the engine.
View SolutionA stone is thrown with a speed of 10ms−1 at an angle of projection 60∘. Find its height above the point of projection when it is at a horizontal distance of 3m from the thrower ? (Take g=10ms−2)
View SolutionA stone is thrown with a speed of 10ms−1 at an angle of projection 60∘. Find its height above the point of projection when it is at a horizontal distance of 3m from the thrower ? (Take g=10ms−2)
View SolutionA body of mass 10 kg moves with a constant speed v of 2 ms−1 along a circular path of radius 8 m. The power produced by the body will be
View SolutionA particle of mass 0.2 kg has an intial speed of 5ms−1 at the bottom of a rough inclined plane of inclination 30∘ and vertical height 0.5 m . What is the speed of the particle as it reaches the top of the inclined plan ? (Take μ=1/√3,g=10ms−2 ) .
View SolutionAn object of mass 10kg moves at a constant speed of 10 ms−1. A constant force, that acts for 4 sec on the object, gives it a speed of 2ms−1 in opposite direction. The force acting on the object is :
View SolutionA stone of mass 0.4 kg is thrown vertically up with a speed of 9.8 ms−1. Find the potential energy after half second.
View SolutionIf the force constant of spring is 50Nm−1, find mass of the block, if it an rests in the given situation (g=10ms−2).
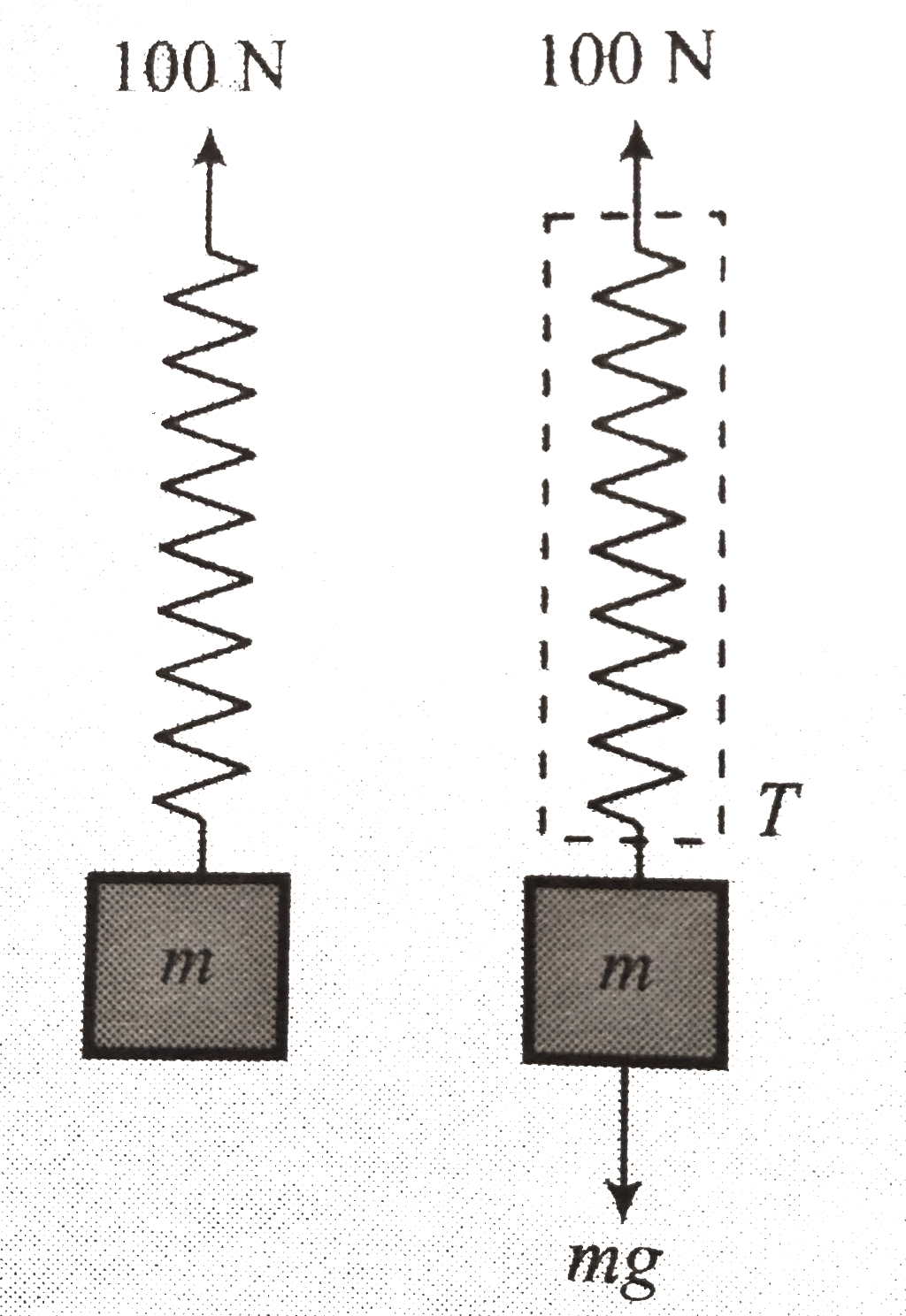
View SolutionA particle is projected at an angle of 45∘ with a velocity of 9.8ms−1. The horizontal range will be (Take, g=9.8ms−2)
View SolutionA cubical block (of side 2m) of mass 20kg slides on inclined plane lubricated with the oil of viscosity η=10−1 with constant velocity of 10ms−1. Find out the thickness of the layer of liquid (take g=10ms−2).

View Solution
