A block of mass 5 kg is kept on a horizontal floor having coefficient of friction 0.09. Two mutually perpendicular horizontal forces of 3 N and 4 N this block. The accelation of the block is ( take, g=10ms−2)
zero
0.1ms−2
0.2ms−2
0.3ms−2
The correct Answer is:B
To solve the problem step by step, we will follow these calculations:
Step 1: Identify the forces acting on the block.
- The block has two perpendicular forces acting on it: Fx=3N and Fy=4N.
Step 2: Calculate the resultant force.
- Since the forces are perpendicular, we can use the Pythagorean theorem to find the resultant force F′:
F′=√F2x+F2y=√32+42=√9+16=√25=5N
Step 3: Calculate the normal force (N).
- The normal force can be calculated using the formula:
N=m⋅g
where m=5kg and g=10m/s2:
N=5⋅10=50N
Step 4: Calculate the maximum force of friction (F_max).
- The maximum force of friction can be calculated using the coefficient of friction (μ):
Fmax=μ⋅N
Given μ=0.09:
Fmax=0.09⋅50=4.5N
Step 5: Calculate the net force (F).
- The net force acting on the block is the resultant force minus the maximum frictional force:
F=F′−Fmax=5−4.5=0.5N
Step 6: Calculate the acceleration (a) of the block.
- Using Newton's second law, F=m⋅a:
a=Fm=0.55=0.1m/s2
Thus, the acceleration of the block is 0.1m/s2.
- The block has two perpendicular forces acting on it: Fx=3N and Fy=4N.
- Since the forces are perpendicular, we can use the Pythagorean theorem to find the resultant force F′:
F′=√F2x+F2y=√32+42=√9+16=√25=5N
- The normal force can be calculated using the formula:
N=m⋅g
where m=5kg and g=10m/s2:
N=5⋅10=50N
- The maximum force of friction can be calculated using the coefficient of friction (μ):
Fmax=μ⋅N
Given μ=0.09:
Fmax=0.09⋅50=4.5N
- The net force acting on the block is the resultant force minus the maximum frictional force:
F=F′−Fmax=5−4.5=0.5N
- Using Newton's second law, F=m⋅a:
a=Fm=0.55=0.1m/s2
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Match the columns
7 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.4
20 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
A block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the block will be.
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View Solution
Knowledge Check
A block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the block will be.
A10ms−2B5ms−2C15ms−2D0.5ms−2Two blocks A and B of masses 10 kg and 15 kg are placed in contact with each other rest on a rough horizontal surface as shown in the figure. The coefficient of friction between the blocks and surface is 0.2. A horizontal force of 200 N is applied to block A. The acceleration of the system is (Take g=10ms−2)
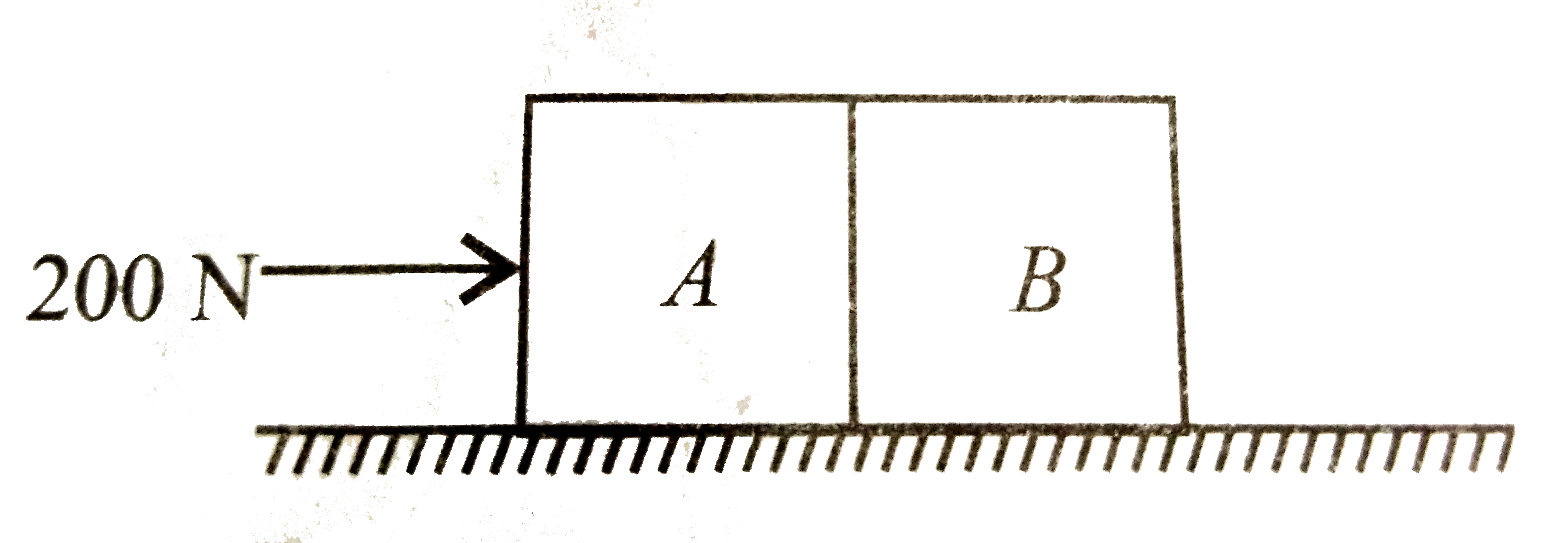 A4ms−2B6ms−2C8ms−2D10ms−2
A4ms−2B6ms−2C8ms−2D10ms−2
A block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View SolutionA block of mass m=5kg is resting on a rough horizontal surface for which the coefficient of friction is 0.2 . When a force F=40N is applied, the acceleration of the block will be (g=10m/s2) .

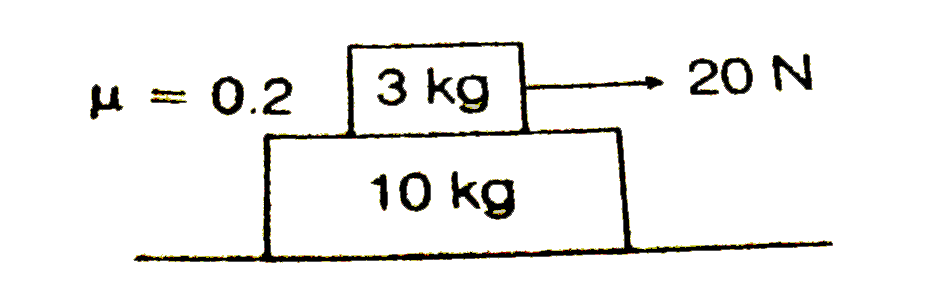
View SolutionA 3 kg block is placed over a 10 kg block and both are palced on a smooth horizontal surface. The coefficient of friction between the block is 0.2 . If a horizontal force of 20 N is applied to 3 kg block, accelerations of the two block in ms−2 are (take, g=ms−2)
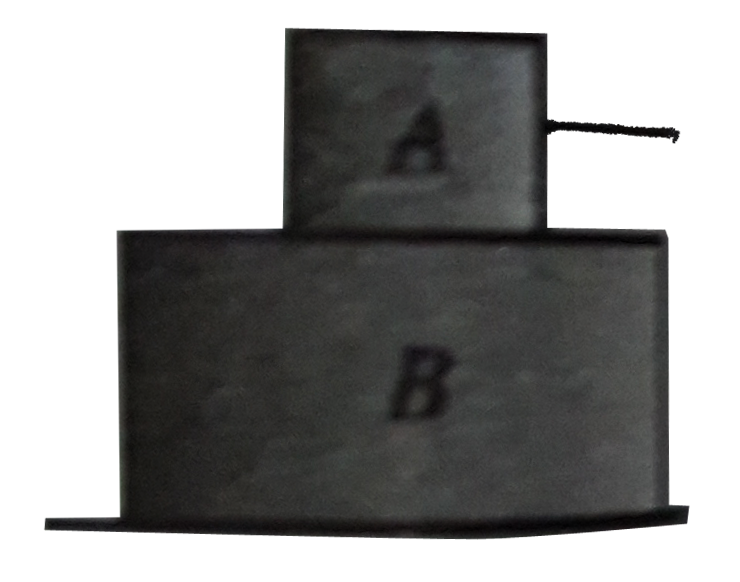
View SolutionA block A of mass 2kg is placed over smooth block B of mass 4kg which is placed over a smooth horizontal floor The coefficient of friction between A and B is 0.4 when a horizontal forces of magnitude 10N is applied on A the acceleration of block of A and B are
View SolutionA block of mass 2 kg is kept on a rough horizontal floor an pulled with a force F. If the coefficient of friction is 0.5. then the minimum force required to move the block is :-

View SolutionA block of mass m is kept on a horizontal table. If the static friction coefficient is μ., find the frictional force acting on the block.
View Solution
