A wooden box of mass 8kg slides down an inclined plane of inclination 30∘ to the horizontal with a constant acceleration of 0.4ms−2 What is the force of friction between the box and inclined plane ? (g=10m/s2) .
36.8N
76.8 N
65.6 N
none of these
The correct Answer is:A
To find the force of friction between the wooden box and the inclined plane, we can follow these steps:
Step 1: Identify the given data
- Mass of the box (m) = 8 kg
- Acceleration (a) = 0.4 m/s²
- Angle of inclination (θ) = 30°
- Acceleration due to gravity (g) = 10 m/s²
Step 2: Calculate the gravitational force acting on the box
The gravitational force (weight) acting on the box can be calculated using the formula:
Fg=m⋅g
Substituting the values:
Fg=8kg⋅10m/s2=80N
Step 3: Resolve the gravitational force into components
The gravitational force can be resolved into two components:
1. Perpendicular to the inclined plane:
F⊥=Fg⋅cos(θ)
2. Parallel to the inclined plane:
F∥=Fg⋅sin(θ)
Calculating F∥:
F∥=80N⋅sin(30∘)
Since sin(30∘)=12:
F∥=80N⋅12=40N
Step 4: Apply Newton's second law
According to Newton's second law, the net force acting on the box along the inclined plane is given by:
Fnet=m⋅a
The net force can also be expressed as:
Fnet=F∥−Ff
Where Ff is the force of friction.
Setting the two expressions for net force equal gives:
F∥−Ff=m⋅a
Step 5: Solve for the force of friction
Rearranging the equation to solve for Ff:
Ff=F∥−m⋅a
Substituting the known values:
Ff=40N−(8kg⋅0.4m/s2)
Ff=40N−3.2N
Ff=36.8N
Final Answer
The force of friction between the box and the inclined plane is 36.8 N.
---
- Mass of the box (m) = 8 kg
- Acceleration (a) = 0.4 m/s²
- Angle of inclination (θ) = 30°
- Acceleration due to gravity (g) = 10 m/s²
The gravitational force (weight) acting on the box can be calculated using the formula:
Fg=m⋅g
Substituting the values:
Fg=8kg⋅10m/s2=80N
The gravitational force can be resolved into two components:
1. Perpendicular to the inclined plane:
F⊥=Fg⋅cos(θ)
2. Parallel to the inclined plane:
F∥=Fg⋅sin(θ)
F∥=80N⋅sin(30∘)
Since sin(30∘)=12:
F∥=80N⋅12=40N
According to Newton's second law, the net force acting on the box along the inclined plane is given by:
Fnet=m⋅a
Fnet=F∥−Ff
Where Ff is the force of friction.
F∥−Ff=m⋅a
Rearranging the equation to solve for Ff:
Ff=F∥−m⋅a
Ff=40N−(8kg⋅0.4m/s2)
Ff=40N−3.2N
Ff=36.8N
The force of friction between the box and the inclined plane is 36.8 N.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Match the columns
7 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Medical entrances gallery
39 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Check point 5.4
20 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
A block of mass m slides down a rough inclined plane with an acceleration g2

View SolutionA block of mass 8 kg is sliding on a surface inclined at an angle of 45∘ with the horizontal. Calculate the acceleration of the block. The coefficient of friction between the block and surface is 0.6. (Take g=10m/s2)
View Solution
Knowledge Check
A body of mass 100 g is sliding from an inclined plane of inclination 30∘. What is the frictional force experienced if μ=1.7 ?
A1.7×√2×(1/√3)NB1.7×√3×(1/2)NC1.7×√3 ND1.7×√2×(1/3) N
A block of mass 8 kg is sliding on a surface inclined at an angle of 45∘ with the horizontal . Calculate the acceleration of the block . The coefficient of friction between the block and surface is 0⋅6 ( Take g = 10m/s2)
View SolutionA block slides down on inclined plane (angle of inclination 60∘ ) with an accelration g/2 Find the coefficient friction

View SolutionA block slides down a frictionless, inclined plane that makes a 30∘ angle with the horizontal. Find the acceleration of this block.
View SolutionA block of mass 5.0 kg slides down an incline of inclination 300 and length 10 m. find the work done by the force of gravity.
View SolutionA block of mass m slides down an inclined plane of inclination θ with uniform speed. The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is
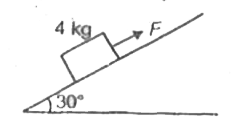
View SolutionA block of mass 4 kg is pulled along a smooth inclined plane of inclination 30∘ with constant velocity 3 m/s as shown, power delivered by the force is
View SolutionA box of mass 8kg placed on a rough inclined plane of inclination θ its downward motion can be prevented by applying an upward pull F and it can be made to slide upward appliying a force 2F .The coefficient of friction between the box and the inclined plane is
View Solution
