A particle is projected from horizontal making an angle of 53∘ with initial velocity 100ms−1. The time taken by the particle to make angle 45∘ from horizontal is
14s
2s
Both (a) and (b)
None of these
The correct Answer is:C
To solve the problem of finding the time taken by a particle projected at an angle of 53∘ with an initial velocity of 100m/s to make an angle of 45∘ with the horizontal, we can follow these steps:
Step 1: Resolve the initial velocity into horizontal and vertical components.
The initial velocity u can be resolved into horizontal (ux) and vertical (uy) components using trigonometric functions:
ux=u⋅cos(θ)=100⋅cos(53∘)
uy=u⋅sin(θ)=100⋅sin(53∘)
Using the values of cos(53∘)=35 and sin(53∘)=45:
ux=100⋅35=60m/s
uy=100⋅45=80m/s
Step 2: Determine the conditions for the angle of 45∘.
At the point where the particle makes an angle of 45∘ with the horizontal, the horizontal and vertical components of the velocity (vx and vy) will be equal:
vx=vy
Since the horizontal component of velocity remains constant:
vx=ux=60m/s
Thus, we have:
vy=60m/s
Step 3: Use the kinematic equation to find the time.
The vertical component of the velocity can be expressed using the kinematic equation:
vy=uy+a⋅t
Where:
- vy=60m/s
- uy=80m/s
- a=−g=−10m/s2 (acceleration due to gravity)
Substituting the values:
60=80−10t
Rearranging gives:
10t=80−60
10t=20
t=2s
Step 4: Find the second time when the angle is 45∘.
The second instance occurs when the particle is descending. At that point, the vertical velocity will be −60m/s:
−60=80−10t
Rearranging gives:
−60−80=−10t
−140=−10t
t=14s
Final Result:
The times at which the particle makes an angle of 45∘ with the horizontal are t1=2s and t2=14s.
The initial velocity u can be resolved into horizontal (ux) and vertical (uy) components using trigonometric functions:
uy=u⋅sin(θ)=100⋅sin(53∘)
uy=100⋅45=80m/s
At the point where the particle makes an angle of 45∘ with the horizontal, the horizontal and vertical components of the velocity (vx and vy) will be equal:
The vertical component of the velocity can be expressed using the kinematic equation:
- vy=60m/s
- uy=80m/s
- a=−g=−10m/s2 (acceleration due to gravity)
10t=20
t=2s
The second instance occurs when the particle is descending. At that point, the vertical velocity will be −60m/s:
−140=−10t
t=14s
The times at which the particle makes an angle of 45∘ with the horizontal are t1=2s and t2=14s.
Topper's Solved these Questions
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - B. Medical entrance
1 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - Medical entrance
12 Videos
- MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - MOTIONEXERCISE - A. Taking it together
1 Videos
- MEASUREMENT AND ERRORSBOOK - DC PANDEY ENGLISHCHAPTER - MEASUREMENT AND ERRORSEXERCISE - Subjective
19 Videos
- MOTION IN A PLANEBOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery
32 Videos
Similar Questions
A particle is projected at an angle θ=30∘ with the horizontal, with a velocity of 10ms−1. Then
View SolutionA particle is projected at an angle of 45∘ with a velocity of 9.8ms−1. The horizontal range will be (Take, g=9.8ms−2)
View SolutionA particle is projected with velocity 50 m/s at an angle 60∘ with the horizontal from the ground. The time after which its velocity will make an angle 45∘ with the horizontal is
View SolutionA projectile is projected from the ground by making an angle of 60∘ with the horizontal. After 1 s projectile makes an angle of 30∘ with the horizontal . The maximum height attained by the projectile is (Take g=10ms−2)
View SolutionA projectile is thrown in the upward direction making an angle of 60∘ with the horizontal direction with a velocity of 150ms−1. Then the time after which its inclination with the horizontal is 45∘ is
View SolutionA particle is projected from the inclined plane at angle 37∘ with the inclined plane in upward direction with speed 10m/s. The angle of inclined plane with horizontal is 53∘. Then the maximum height attained by the particle from the inclined plane will be-
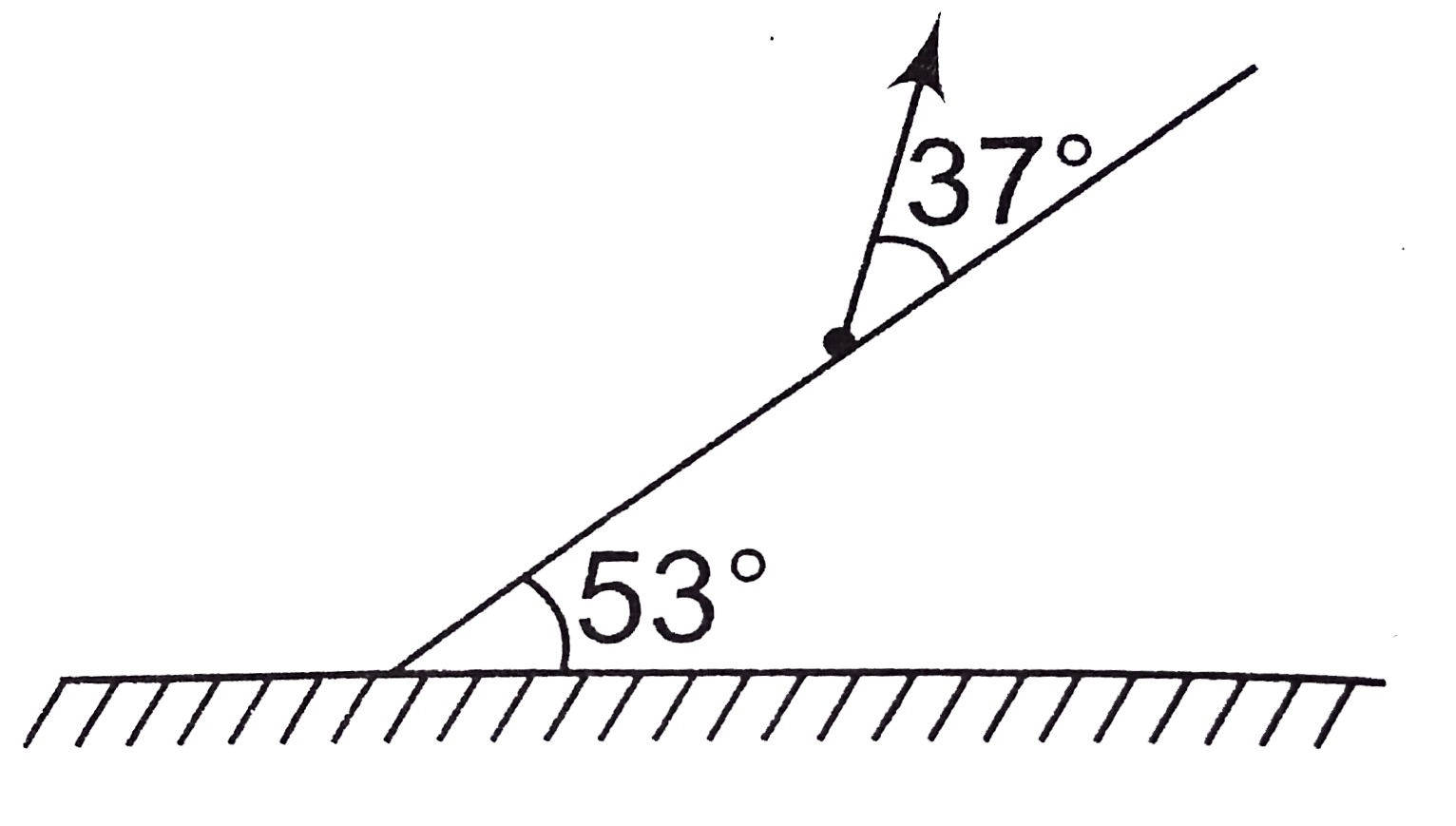
View SolutionA ball is projected at an angle 60∘ with the horizontal with speed 30 m/s. What will be the speed of the ball when it makes an angle 45∘ with the horizontal ?
View SolutionA particle is projected from the ground with velocity u making an angle θ with the horizontal. At half of its maximum heights,
View SolutionA particle is projected at an angle of 60∘ above the horizontal with a speed of 10m/s. After some time the direction of its velocity makes an angle of 30∘ above the horizontal. The speed of the particle at this instant is
View SolutionFind the ratio of de Broglie wavelengths of proton and α-particle of same energy
View Solution
