A dust particle is inside a sphere of refractive index 43. If the dust particle is 10.0cm from the wall of the 15.0cm radius bowl, where does it appear to an observer outside the bowl.
The correct Answer is:A, B, C, D
To solve the problem of where the dust particle appears to an observer outside the bowl, we will use the refraction formula for a spherical surface. Here are the steps to arrive at the solution:
1. Identify the Given Values:
- Refractive index of the medium (bowl) μ2=43
- Refractive index of air (outside the bowl) μ1=1
- Distance of the dust particle from the wall of the bowl d=10.0cm
- Radius of the bowl R=15.0cm
2. Calculate the Distance from the Center of the Bowl:
- The distance of the dust particle from the center of the bowl is given by:
U=R−d=15.0cm−10.0cm=5.0cm
- Since the object (dust particle) is inside the bowl, we take U as negative:
U=−5.0cm
3. Apply the Spherical Surface Refraction Formula:
- The formula for refraction at a spherical surface is given by:
μ2V−μ1U=μ2−μ1R
- Substituting the known values:
43V−1−5=43−1−15
4. Simplify the Equation:
- Calculate μ2−μ1:
43−1=43−33=13
- Substitute this back into the equation:
43V+15=13−15
- Simplifying the right side:
13÷−15=−145
- Now the equation becomes:
43V+15=−145
5. Solve for V:
- Rearranging gives:
43V=−145−15
- Finding a common denominator for the right side (which is 45):
−145−945=−1045=−29
- Thus:
43V=−29
- Cross-multiplying gives:
4⋅9=−2⋅3V⟹36=−6V⟹V=−6cm
6. Interpret the Result:
- The negative sign indicates that the image appears on the same side as the object, which means it appears to be located 6cm from the center of the bowl towards the outside.
Final Answer:
The dust particle appears to be located 6cm from the center of the bowl, which means it appears to be 9cm from the wall of the bowl.
- Refractive index of the medium (bowl) μ2=43
- Refractive index of air (outside the bowl) μ1=1
- Distance of the dust particle from the wall of the bowl d=10.0cm
- Radius of the bowl R=15.0cm
- The distance of the dust particle from the center of the bowl is given by:
U=R−d=15.0cm−10.0cm=5.0cm
- Since the object (dust particle) is inside the bowl, we take U as negative:
U=−5.0cm
- The formula for refraction at a spherical surface is given by:
μ2V−μ1U=μ2−μ1R
- Substituting the known values:
43V−1−5=43−1−15
- Calculate μ2−μ1:
43−1=43−33=13
- Substitute this back into the equation:
43V+15=13−15
- Simplifying the right side:
13÷−15=−145
- Now the equation becomes:
43V+15=−145
- Rearranging gives:
43V=−145−15
- Finding a common denominator for the right side (which is 45):
−145−945=−1045=−29
- Thus:
43V=−29
- Cross-multiplying gives:
4⋅9=−2⋅3V⟹36=−6V⟹V=−6cm
- The negative sign indicates that the image appears on the same side as the object, which means it appears to be located 6cm from the center of the bowl towards the outside.
The dust particle appears to be located 6cm from the center of the bowl, which means it appears to be 9cm from the wall of the bowl.
Topper's Solved these Questions
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Exercise 31.6
12 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Exercise 31.7
2 Videos
- REFRACTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFRACTION OF LIGHTEXERCISE - Exercise 31.4
1 Videos
- REFLECTION OF LIGHTBOOK - DC PANDEY ENGLISHCHAPTER - REFLECTION OF LIGHTEXERCISE - Subjective
9 Videos
- SEMICONDUCTORSBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORSEXERCISE - Subjective
12 Videos
Similar Questions
An equiconvex lens is made from glass of refractive index 1.5. If the radius of each surface is changed from 5cm to 6cm, then the power
View SolutionA plano-concave lens is made of glass of refractive index 1.5 and the radius of curvature of its curved face is 100 cm. What is the power of the lens?
View SolutionIn a thin spherical fish bowl of radius 10 cm filled with water of refractive index 4/3 there is a small fish at a distance of 4 cm from the centre C as shown in the figure. Where will the image of fish appears, if seen from E.

View SolutionAn air bubble is seen inside a solid sphere of glass (n=1.5) of 4.0cm diameter at a distance of 1.0cm from the surface of the sphere (on seeing along the diameter). Determine the real position of the bubble inside the sphere.
View SolutionA sphere made of transparent material of refractive index (μ=32 )and of radius 50 cm has a small air bubble 10 cm below the surface. The apparent depth of the bubble if viewed from outside normally is
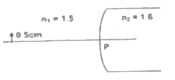
View SolutionAn object of height 0.5 cm is placed laterally in the medium of refractive index m = 1.5 at a distance 100 cm away form the pole. The radius of curvature of the surface is 20 cm. The height of the image formed after refraction is
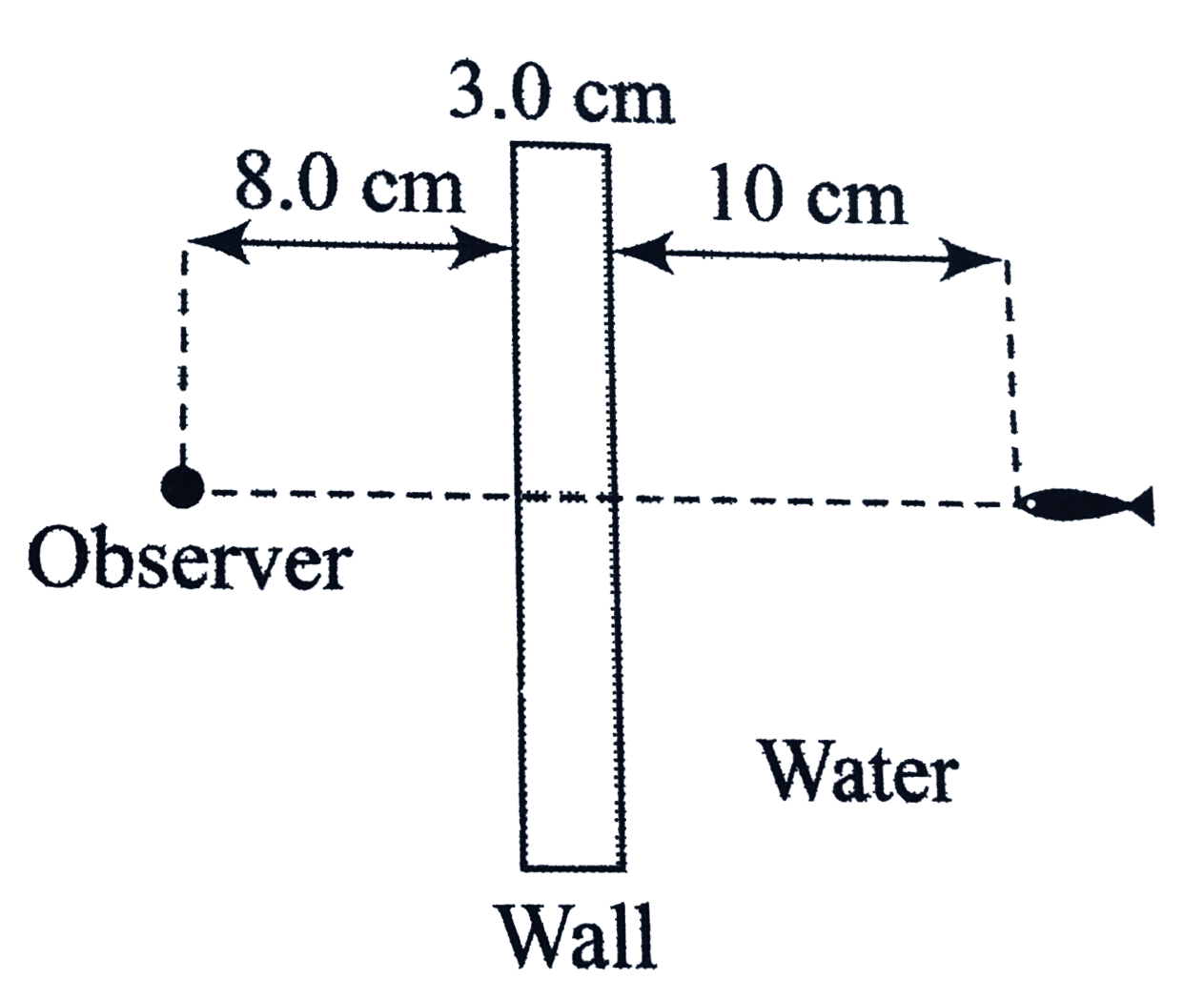
View SolutionIn Figure, a fish watcher watches a fish through a 3.0 cm thick glass wall of a fish tank. The watcher is in level with the fish, the index of refraction of the glass is 8/5 and that of the water 4/3 .
a. To the fish, how far away does the watcher appear to be ?
b. To the watcher, how far away does the fish appear to be ?
View SolutionA spherical convex surface separates object and image space of refractive index 1.0and43. If radius of curvature of the surface is 10cm, find its power.
View SolutionA spherical convex surface separates object and image space of refractive index 1.0and43. If radius of curvature of the surface is 10cm, find its power.
View SolutionA particle moves in a circle of radius 5 cm with constant speed and time period 0.2πs. The acceleration of the particle is
View Solution
