A conducting circular loop of radius a and resistance per unit length R is moving with a constant velocity v0 , parallel to an infinite conducting wire carrying current i0. A conducting rod of length 2a is approaching the centre of the loop with a constant velocity v02 along the direction 2 of the current. At the instant t=0, the rod comes in contact with the loop at A and starts sliding on the loop with the constant velocity. Neglecting the resistance of the rod and the self-inductance of the circuit, find the following when the rod slides on the loop.
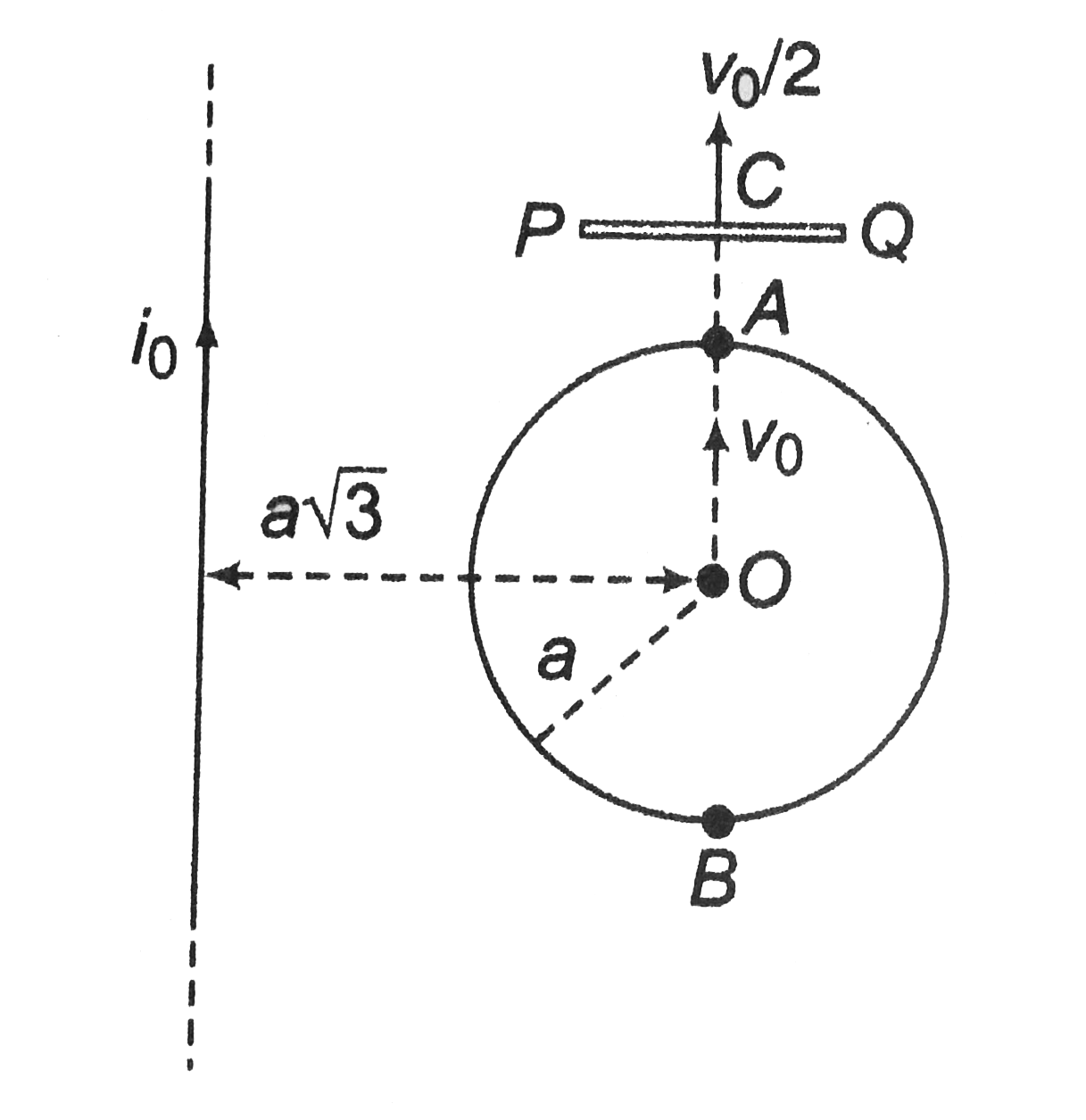
(a) The current through the rod when it is at a distance of (a2) from the point A of the loop.
(b) Force required to maintain the velocity of the rod at that instant.
The correct Answer is:B, C
a. At the given instant
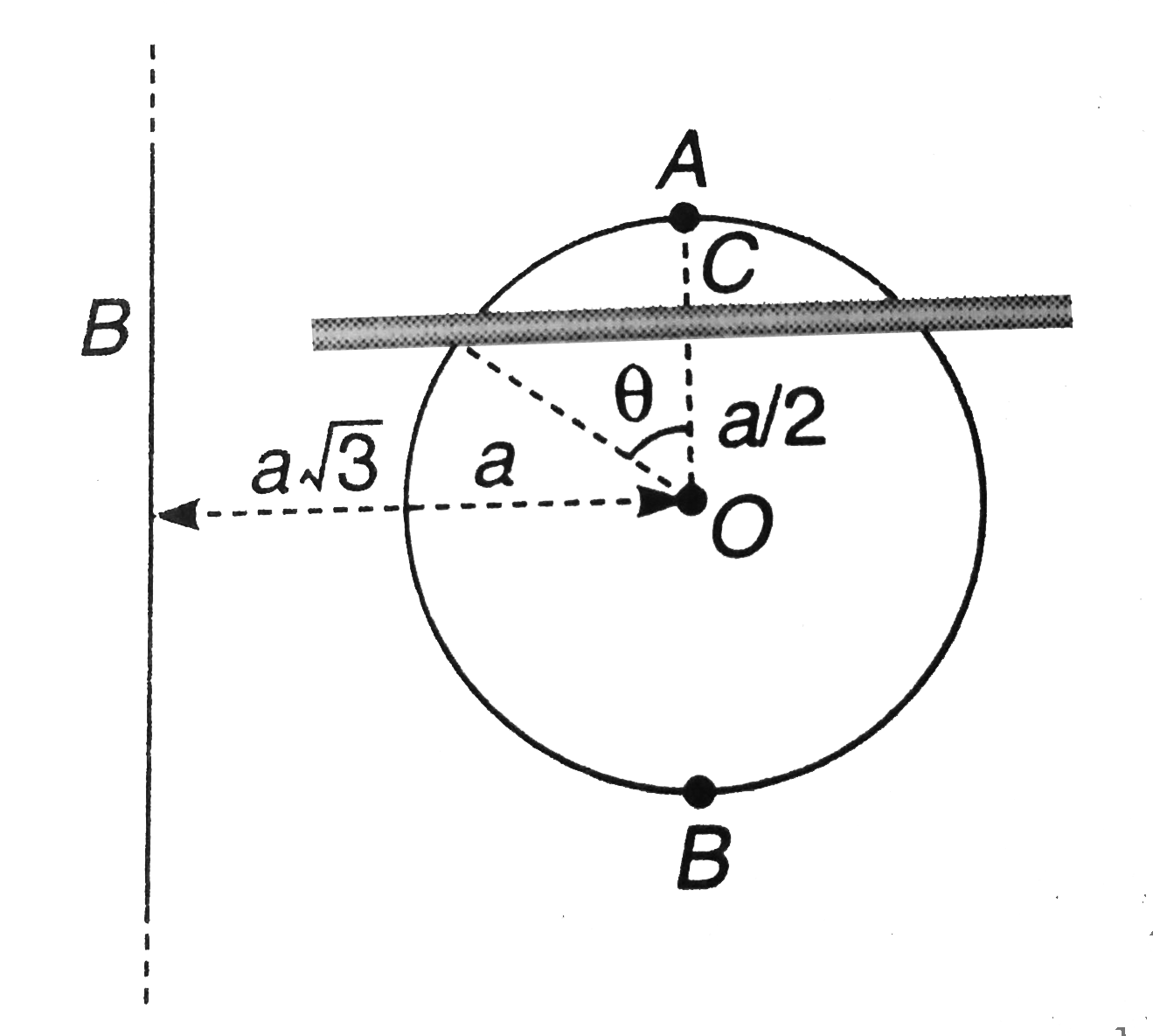
AC=a2,OC=a2
and cosθ=a2a=12,θ=π3
∴ Velocity of rod
=(+v02) along the direction of current.
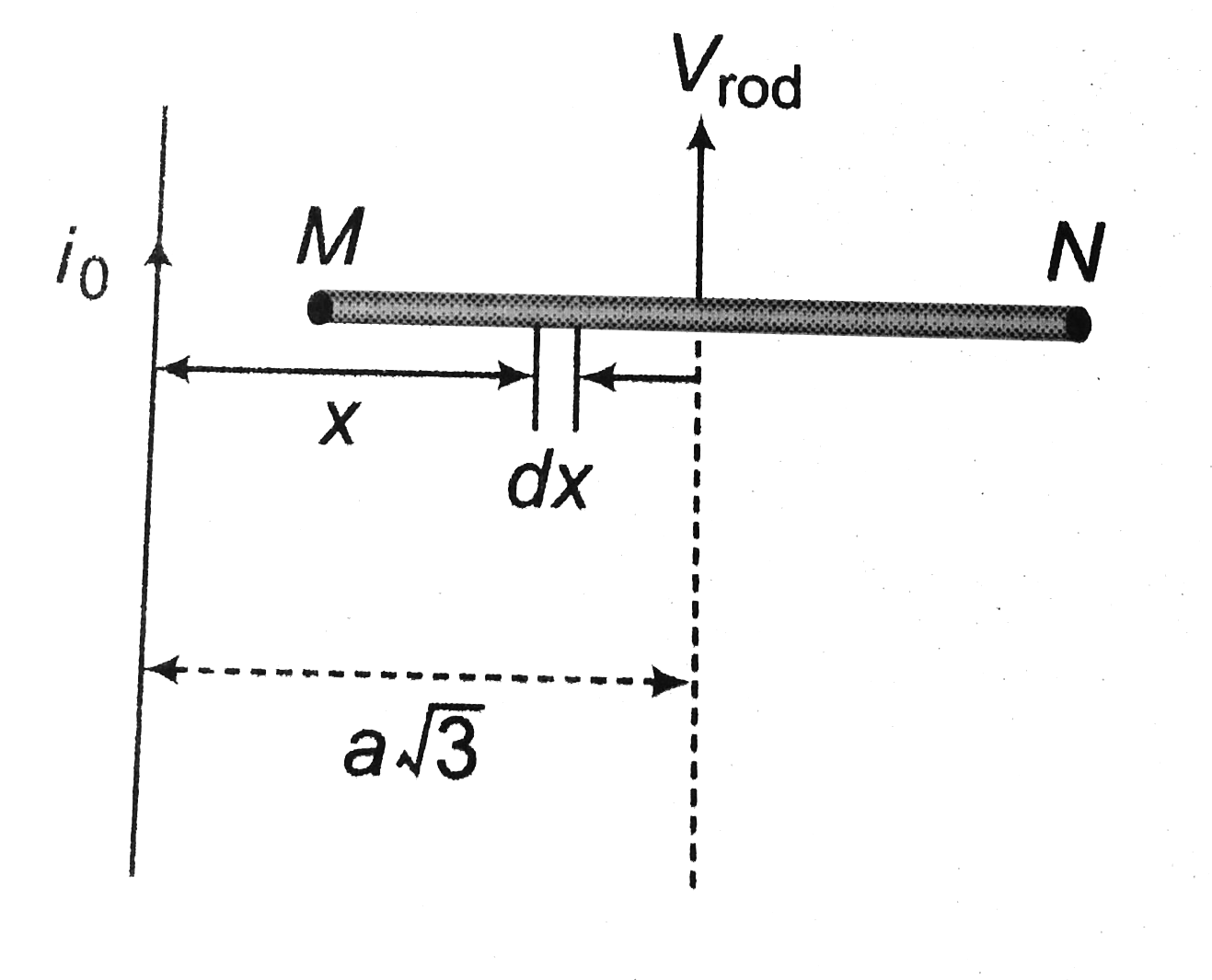
Emf induced across the ends M and N
Erod=∫a√3+a√32a√3−a√32vrodBdx
=vrod∫3a√32a√32μ0i02πxdx
(v02)μ0i02πln(31)
with end M at higher potential.
Since, the effective length of both the arcs MAN and MBN is MN.
∴EMAN=EMBN+Vloopμ0i02πln3
=v0μ0i02πlne
with point M at higher potential.
Resistance of arc MAN
⇒R1=(R)2(aθ)=2aRπ3
⇒ Resistance of arc MBN
⇒R2=(R)a(2π−2θ)=4aRπ3
Equivalent circuit at the given instant is shown in figure.

Current through the rod MN,
i=(i1+i2)=(EMAN−ErodR1)+(EMBN−RrodR2)
i=(EMAN−Erod)[1R1+1R1]
=(v0μ0i0)(ln304π)[912+14][3aRπ]
9v0i0μ016aRπ2ln(3)
b. Force on the rod

Frod=∫3a√32a√32B
=iμ0i02πln3=9μ20i20v032aRπ3(ln3)2
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Example
71 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Check point
60 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - SUBJECTIVE TYPE
1 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Medical entrances gallery
97 Videos
- ELECTROMAGNETIC WAVESBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Sec C
22 Videos
Similar Questions
A conducting rod of length l is moving in a transverse magnetic field of strength B with veocity v. The resistance of the rod is R. The current in the rod is
View SolutionA conducting rod AB of length l is projected on a frictionless frame PSRQ with velocity v0 at any instant. The velocity of the rod after time t is
View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is
View SolutionA small square loop of edge a and resistance R is moved with velocity v0 away from an infinitely long current carrying conductor carrying current I so that the conductor and side of square are always in same plane. Find induced current in loop at a separation of r
View SolutionA conducting loop is pulled with a constant velocity towards a region of unifrom maganetic field of induction B as shown in the figure. Then the current involved in the loop is (d>r)
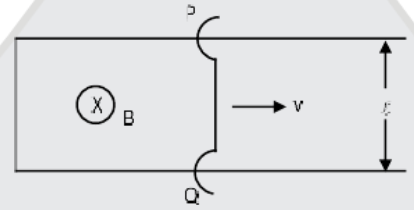
View SolutionA rod having length l and resistance R is moving with velocity v on a π shape conductor. Find the current in the rod.
View SolutionShows a long current carrying wire and two rectangular loops moving with of velocity v. Find the direction of current in each loop.
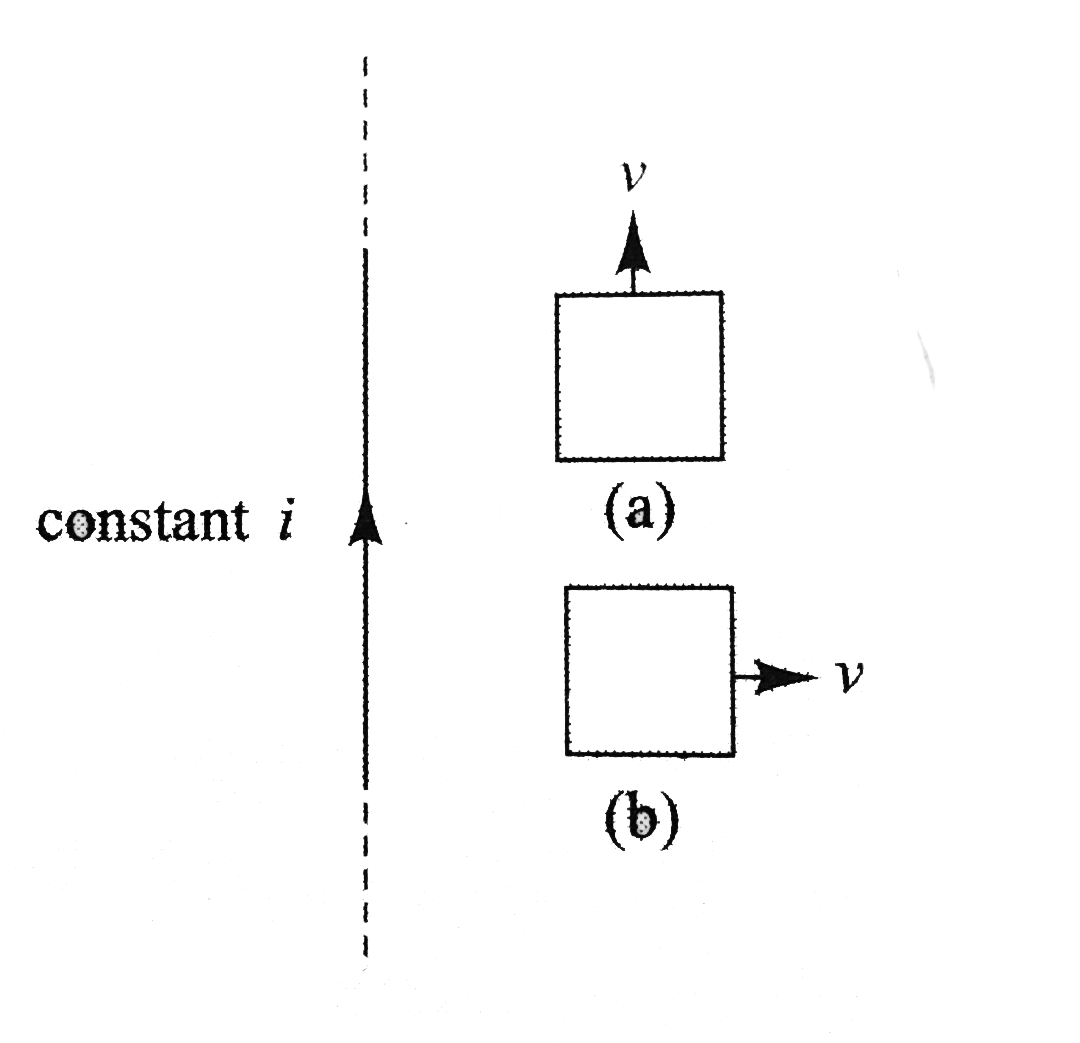
View SolutionShows a long current carrying wire and two rectangular loops moving with of velocity v. Find the direction of current in each loop.

View SolutionShows a long current carrying wire and two rectangular loops moving with of velocity v. Find the direction of current in each loop.

View SolutionA square conducting loop of side length L carries a current I.The magnetic field at the centre of the loop is (dependence on L)
View Solution
