A current of 50A is placed through a straight wire of length 6cm then the magnetic induction at a point 5cm from the either end of the wire is (1gauss=10−4T)
2.5gauss
1.25gauss
1.5gauss
3.0gauss
The correct Answer is:C
To solve the problem of finding the magnetic induction at a point 5 cm from either end of a straight wire carrying a current of 50 A, we can follow these steps:
1. Identify the Given Data:
- Current (I) = 50 A
- Length of the wire (L) = 6 cm = 0.06 m
- Distance from either end to the point (r) = 5 cm = 0.05 m
- Conversion factor: 1 Gauss = 10−4 T
2. Understand the Geometry:
- The wire is of finite length, and we need to find the magnetic field at a point P which is 5 cm from either end of the wire.
- The wire can be considered as extending from point A to point B, where A and B are the ends of the wire.
3. Determine the Angles:
- The distance from the midpoint of the wire to point P is 3 cm (half of the wire length) vertically and 5 cm horizontally.
- Using the Pythagorean theorem, we can find the distances:
- OP2=OA2+AP2
- OP2=32+52
- OP=√9+25=√34≈5.83 cm
4. Calculate Sine of Angles:
- For the angles θ1 and θ2 at points A and B:
- sin(θ1)=35 and sin(θ2)=35
5. Use the Formula for Magnetic Field:
- The formula for the magnetic field (B) due to a finite straight wire is given by:
B=μ0I4πr(sinθ1+sinθ2)
- Where:
- μ0=4π×10−7 T m/A
6. Substituting Values:
- Substitute the known values into the formula:
B=(4π×10−7)×504π×0.05(35+35)
- Simplifying:
B=(10−7×50)0.05×65
7. Calculating the Magnetic Field:
- Calculate:
B=10−6×6=6×10−6 T
8. Convert to Gauss:
- Since 1 Gauss=10−4 T:
B=6×10−610−4=60 Gauss
Final Answer:
The magnetic induction at the point 5 cm from either end of the wire is 60 Gauss.
---
- Current (I) = 50 A
- Length of the wire (L) = 6 cm = 0.06 m
- Distance from either end to the point (r) = 5 cm = 0.05 m
- Conversion factor: 1 Gauss = 10−4 T
- The wire is of finite length, and we need to find the magnetic field at a point P which is 5 cm from either end of the wire.
- The wire can be considered as extending from point A to point B, where A and B are the ends of the wire.
- The distance from the midpoint of the wire to point P is 3 cm (half of the wire length) vertically and 5 cm horizontally.
- Using the Pythagorean theorem, we can find the distances:
- OP2=OA2+AP2
- OP2=32+52
- OP=√9+25=√34≈5.83 cm
- For the angles θ1 and θ2 at points A and B:
- sin(θ1)=35 and sin(θ2)=35
- The formula for the magnetic field (B) due to a finite straight wire is given by:
B=μ0I4πr(sinθ1+sinθ2)
- Where:
- μ0=4π×10−7 T m/A
- Substitute the known values into the formula:
B=(4π×10−7)×504π×0.05(35+35)
- Simplifying:
B=(10−7×50)0.05×65
- Calculate:
B=10−6×6=6×10−6 T
- Since 1 Gauss=10−4 T:
B=6×10−610−4=60 Gauss
The magnetic induction at the point 5 cm from either end of the wire is 60 Gauss.
Topper's Solved these Questions
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - SCQ_TYPE
5 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Videos
- MAGNETICSBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETICSEXERCISE - SUBJECTIVE_TYPE
1 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
- MAGNETISM AND MATTERBOOK - DC PANDEY ENGLISHCHAPTER - MAGNETISM AND MATTEREXERCISE - Medical gallery
1 Videos
Similar Questions
Knowledge Check
If a long straight wire carries a current of 40 A, then the magnitude of the field B at a point 15 cm away from the wire is
A5.34×10−5TB8.34×10−5TC9.6×10−5TD10.2×10−5T
A current i is flowing in a straight conductor of length L. The magnetic induction at a point distant L4 from its centre will be-
View SolutionA current i is flowing in a straight conductor of length L. The magnetic induction at a point distant L4 from its centre will be-
View SolutionA long cylindrical wire carrying current of 10A has radius of 5mm find its its magnetic field induction at a point 2mm from the centre of the wire
View SolutionA long straight wire carries a current of 35A. What is the magnitude of the field →B at a point 20cm from the wire?
View SolutionA wire of length 3 cm has current 1 amp. Find magnetic field at a perpendicular distance a cm from centre of wire
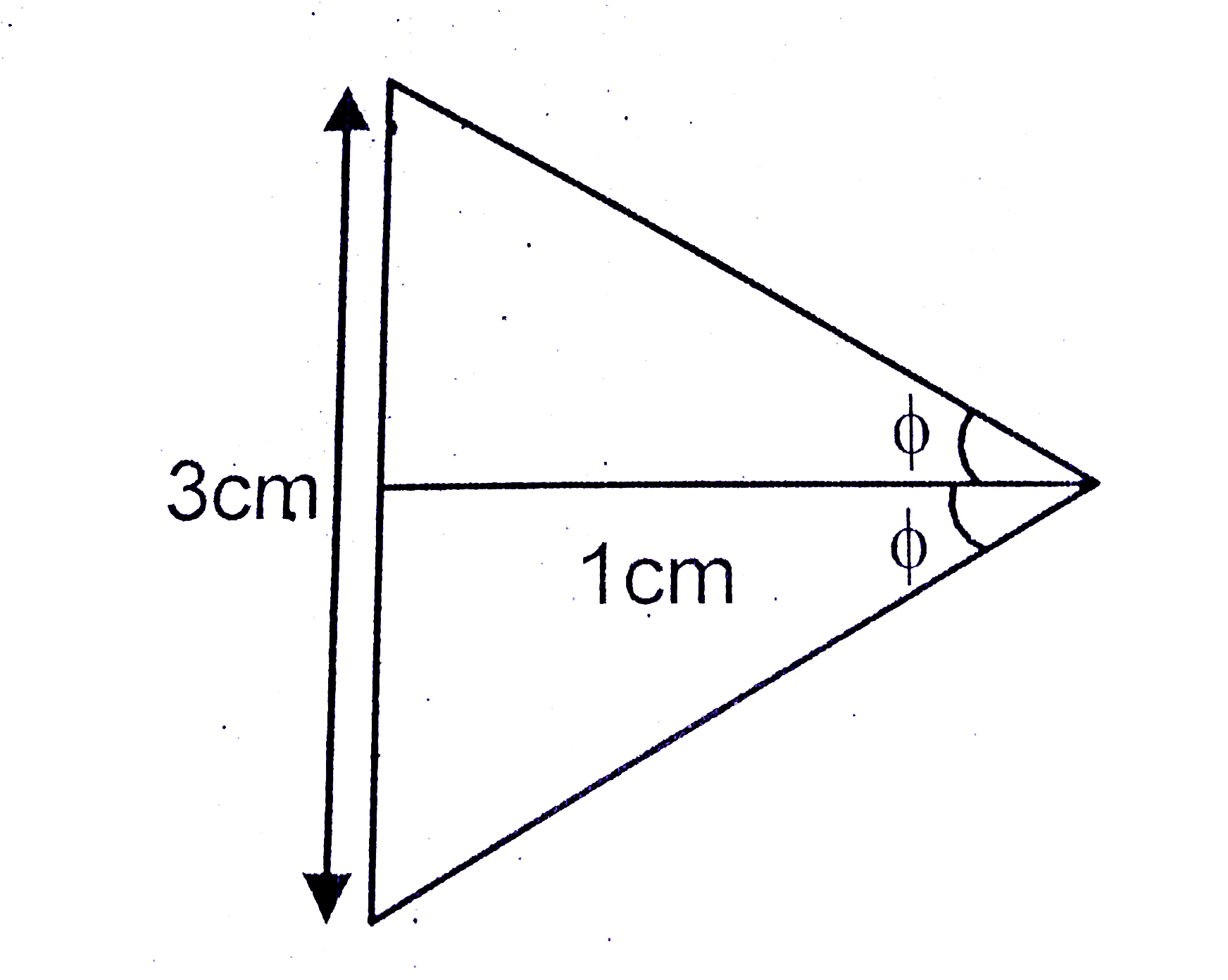
View SolutionA long straight wire carries an electric current of 2A. The magnetic induction at a perpendicular distance of 5m from the wire is (μ04π×107Hm−1)
View SolutionA very long wire carrying a current I=5.0A is bent at right angles. Find the magnetic induction at a point lying on a perpendicular normal to the plane of the wire drawn through the Point of bending at a distance l=35cm from it.
View Solution