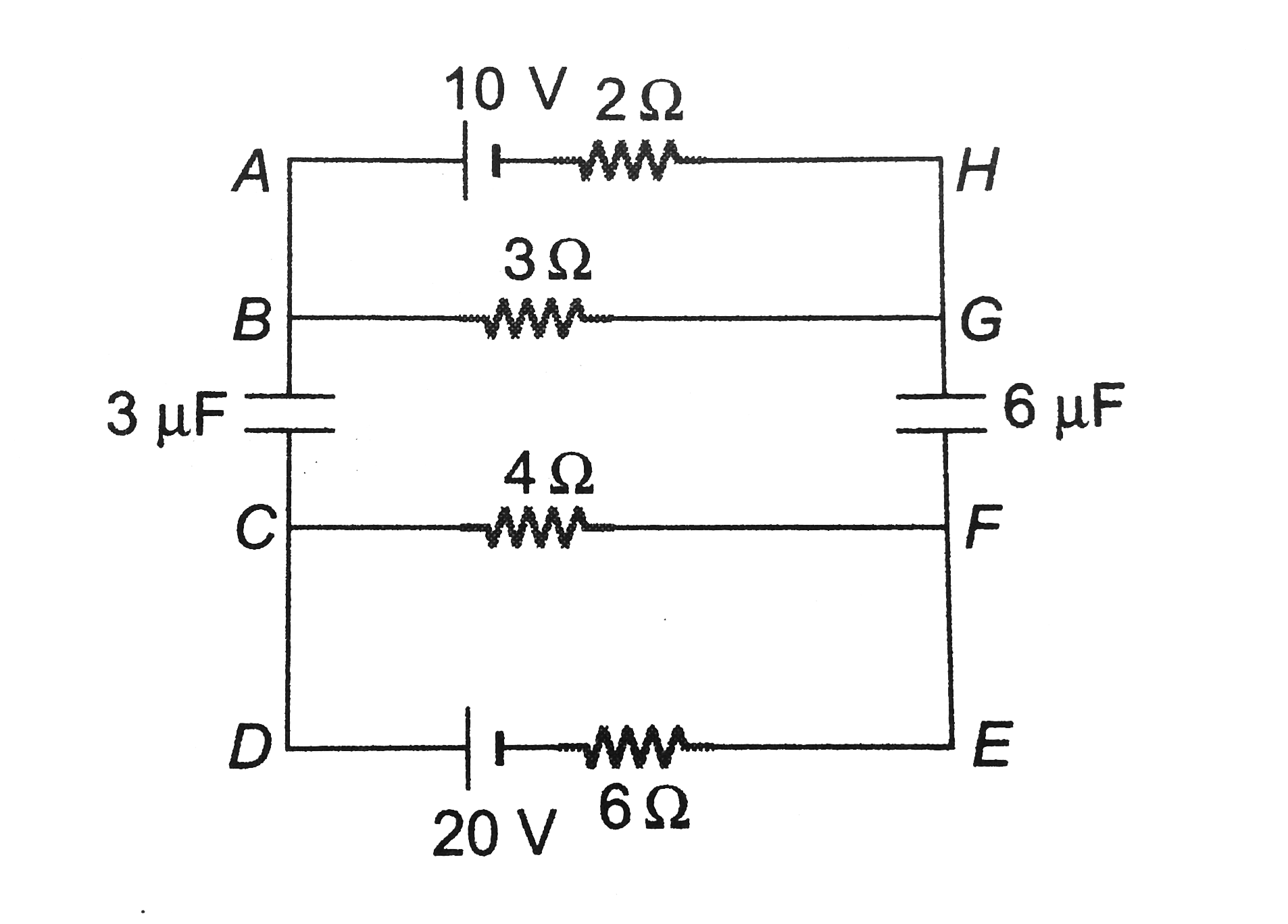
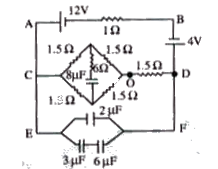
Analyse the given circuit in the steady state condition. Charge on the capacitor is q0=16μC. If the e.m.f. of the battery is 6k then find the value of k.
The correct Answer is:A
 a. Current inlower branch =E8=3A
a. Current inlower branch =E8=3A
Current in uper branch =E9=249=2.67A
b. Pd across the capacitor =E2−E3=E6
From q=CV, we have 16=(4)E6
∴E=24V
c. After short circuitting the bttery, we will have to find net resistance across capacitor to calculate equivalent value of τC in dischargein. 3Ω and 6Ω are in parallel. Similarly 4Ω and 4Ω are in parallel. They are then in series
∴Rnet=4Ω
τC=CRnet=(4×4)μs=16μs
During discharging q=q0e−tτC
or 8=16e−t16
Solving this equation we get t=11.1μs
Topper's Solved these Questions
- CAPACITORSBook:DC PANDEY ENGLISHChapter:CAPACITORSExercise:OBJECTIVE_TYPE
Explore 1 Video
- CAPACITORSBook:DC PANDEY ENGLISHChapter:CAPACITORSExercise:OBJECTIVE_TYPE
Explore 1 Video
- ATOMSBook:DC PANDEY ENGLISHChapter:ATOMSExercise:MEDICAL ENTRANCES GALLERY
Explore 42 Videos
- COMMUNICATION SYSTEMBook:DC PANDEY ENGLISHChapter:COMMUNICATION SYSTEMExercise:Subjective
Explore 11 Videos
Similar Questions
Knowledge Check
In the circuit given, the charge on capacitor C3 at steady state is
 A6μCB12μCC18μCD24μC
A6μCB12μCC18μCD24μC
For the circuit arrangement shown in figure, in the steady state condition charge on the capacitor is
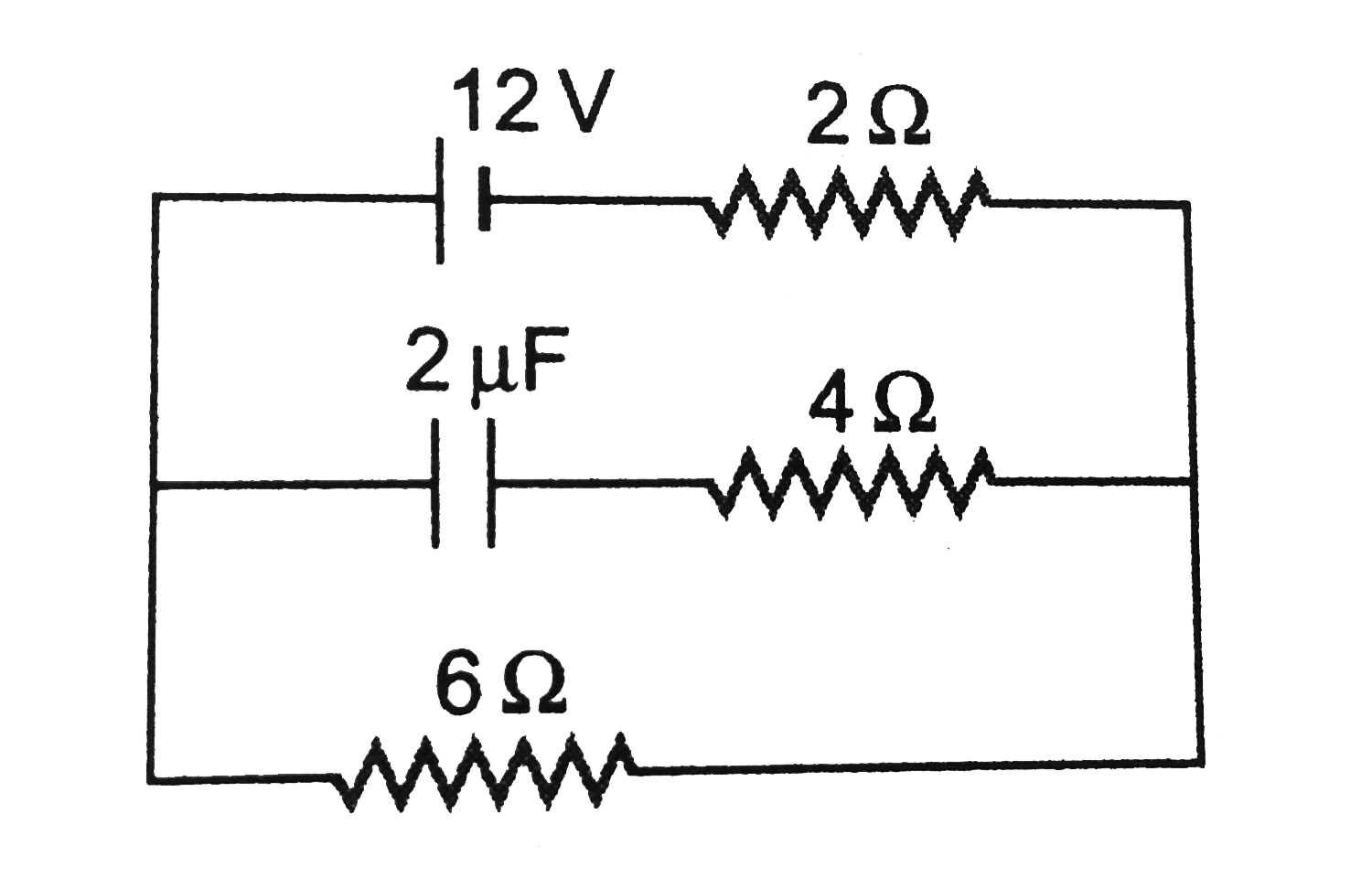
View SolutionIn the given circuit, find the potential differece across the 6μf capacitor in steady state.
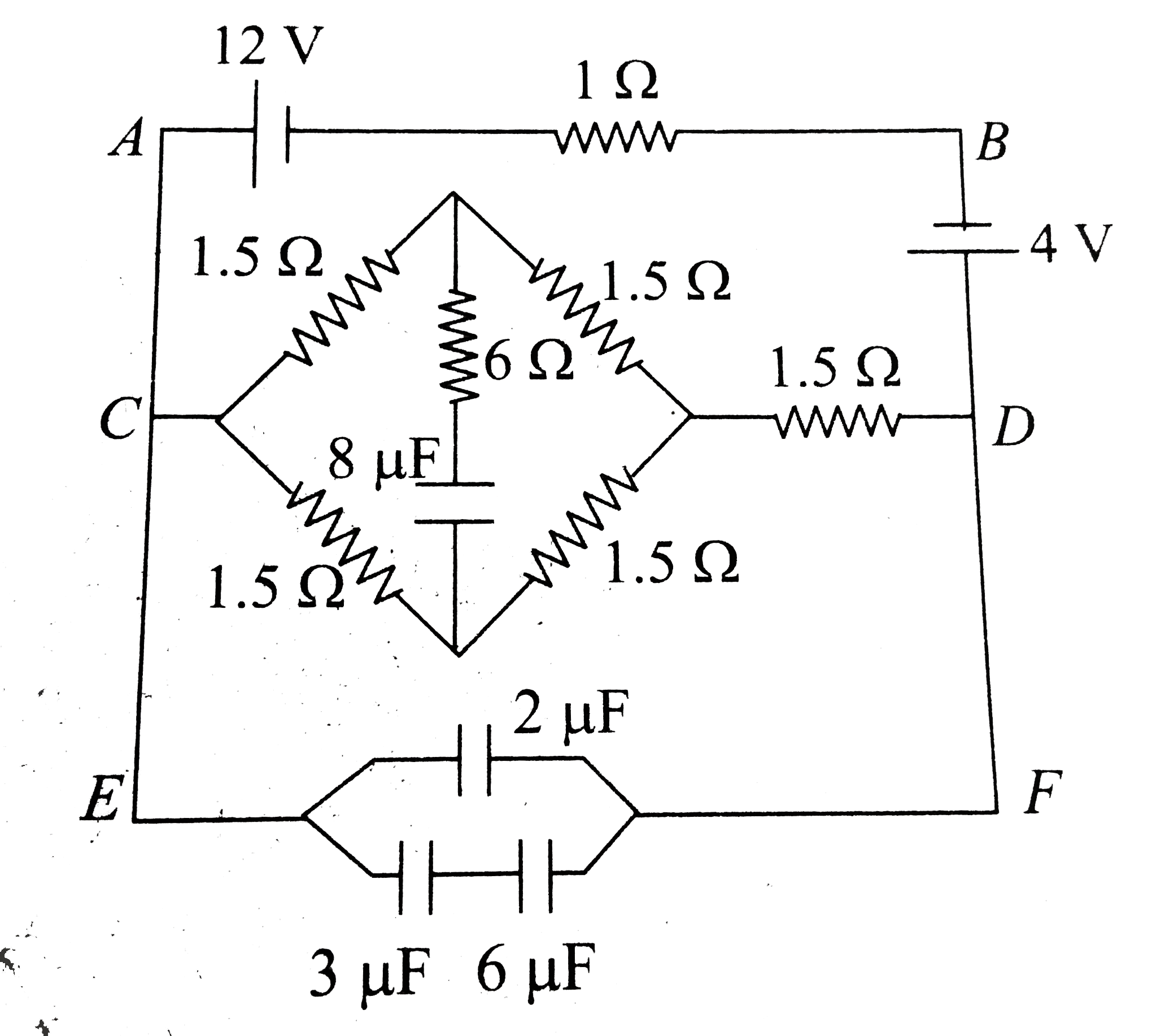
View SolutionFind the current in each branch f the given circuit in steady state. Find the charge on each capacitor.
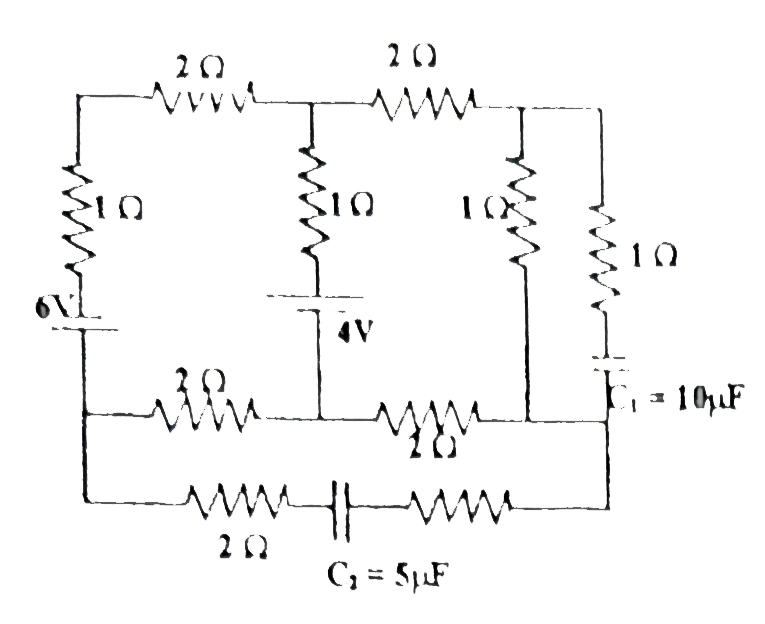
View SolutionIn the given circuit, the potential difference across the 6μF capacitor in steady state is
View SolutionThe circuit shown in fig 5.223 is in steady state.
find the charges on the capacitors C1andC2 respectively.

View SolutionIn the given circuit find out charge on 6μF and 1μF capacitor.

View SolutionIn the given circuit switch K is open. The charge on the capacitor is C is steady-state is q1 Now the key is closed and steady-state charge on C is q2 The ratio of charges q1/q2 is -
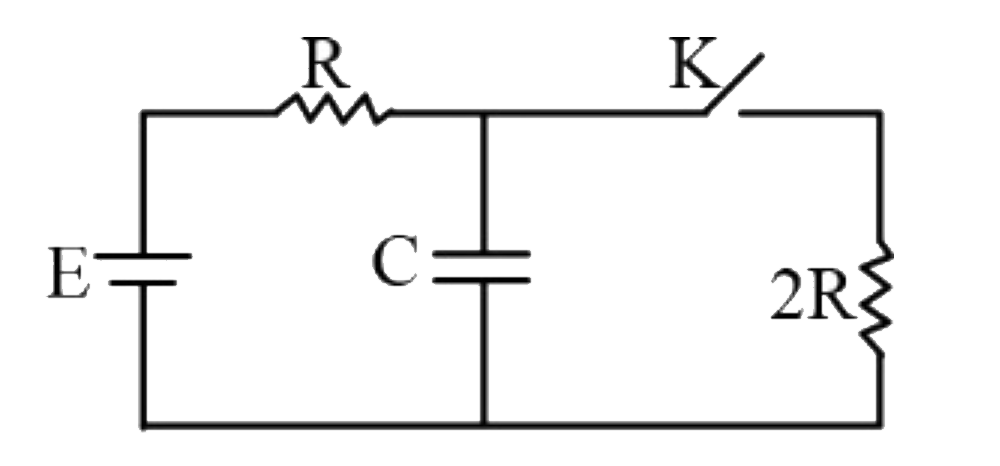
View Solution