A tube 1.0m long is closed at one end. A stretched wire is placed near the open end. The wire is 0.3m long and a mass of 0.01kg . It is held fixed at both ends and vibrates in its fundamental mode. It sets the air column in the tube into vibration at its fundamental frequency by resonance. Find
(a) the frequency of oscillation of the air column and
(b) the tension in the wire.
Speed of sound in air = 330m/s .
The correct Answer is:A, B
To solve the problem step by step, we will first find the frequency of oscillation of the air column and then calculate the tension in the wire.
Step 1: Understand the relationship between the frequencies of the air column and the wire
In this problem, we have a tube closed at one end (the air column) and a stretched wire vibrating in its fundamental mode. The frequencies of both the air column and the wire will be the same due to resonance.
Step 2: Calculate the frequency of the air column
The fundamental frequency F2 of the air column can be calculated using the formula:
F2=V24L1
where:
- V2 is the speed of sound in air (given as 330m/s),
- L1 is the length of the air column (which is 1.0m).
Substituting the values:
F2=3304×1.0=3304=82.5Hz
Step 3: Calculate the frequency of the wire
The fundamental frequency F1 of the wire can be calculated using the formula:
F1=V12L2
where:
- V1 is the speed of the wave on the wire,
- L2 is the length of the wire (which is 0.3m).
Since the frequencies are equal (F1=F2), we can set up the equation:
F1=F2=82.5Hz
Step 4: Calculate the speed of the wave on the wire
From the frequency formula for the wire, we can express the speed V1:
V1=2L2⋅F1
Substituting the known values:
V1=2×0.3×82.5=49.5m/s
Step 5: Calculate the tension in the wire
The speed of the wave on the wire is related to the tension T and the linear mass density μ of the wire:
V1=√Tμ
Rearranging gives:
T=μV21
First, we need to calculate the linear mass density μ:
μ=mL2=0.01kg0.3m=0.010.3≈0.0333kg/m
Now substituting V1 and μ into the tension formula:
T=0.0333×(49.5)2≈0.0333×2450.25≈81.67N
Final Results
(a) The frequency of oscillation of the air column is 82.5Hz.
(b) The tension in the wire is approximately 81.67N.
In this problem, we have a tube closed at one end (the air column) and a stretched wire vibrating in its fundamental mode. The frequencies of both the air column and the wire will be the same due to resonance.
The fundamental frequency F2 of the air column can be calculated using the formula:
F2=V24L1
where:
- V2 is the speed of sound in air (given as 330m/s),
- L1 is the length of the air column (which is 1.0m).
F2=3304×1.0=3304=82.5Hz
The fundamental frequency F1 of the wire can be calculated using the formula:
F1=V12L2
where:
- V1 is the speed of the wave on the wire,
- L2 is the length of the wire (which is 0.3m).
F1=F2=82.5Hz
From the frequency formula for the wire, we can express the speed V1:
V1=2L2⋅F1
Substituting the known values:
V1=2×0.3×82.5=49.5m/s
The speed of the wave on the wire is related to the tension T and the linear mass density μ of the wire:
V1=√Tμ
Rearranging gives:
T=μV21
First, we need to calculate the linear mass density μ:
μ=mL2=0.01kg0.3m=0.010.3≈0.0333kg/m
T=0.0333×(49.5)2≈0.0333×2450.25≈81.67N
(a) The frequency of oscillation of the air column is 82.5Hz.
Topper's Solved these Questions
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Level 1 Assertion And Reason
10 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Level 1 Objective
34 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - TYPE 5.
2 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Level 2 Subjective
8 Videos
Similar Questions
A stretched wire is kept near the open end of a closed pipe 0.75 m long. The wire is 0.3 m long and has a mass of 0.01 kg. The wire is made to vibrate in its fundamental node, after Ixing at both ends. It sets the air column in the tube hito vibration at its fundamental frequency by resonance. Find (a) the frequency of oscillation of the air column and 6) the tension in the wire. Velocity of sound =330 m/s.
View SolutionA 30.0-cm-long wire having a mass of 10.0 g is fixed at the two ends and is vibrated in its fundamental mode.A 50.0-cm-long closed organ pipe, placed with its open end near the wire, is set up into resonance in its fundamental mode by the vibrating wire. Find the tension in the wire. Speed of sound in air =340ms−1.
View SolutionA wire of length 40 cm which has a mass of 4 gms oscillates in its second harmonic and sets the air column in the tube to vibrations in its fundamental mode as shown in figure. Assuming speed of sound in air as 340 m/s, find the tension in the wire.

View SolutionA string fixed at both ends first oscillates in its fundamental mode then in second harmonic mode.Then match the following.

View SolutionA wire of length 40cm which has a mass of 4 g oscillates in its second harmonic and sets the air column in the tube to vibrations in its funrations in its fundamental mode as shows in figure. Assuming the speed of sound in air as 340m/s . Find the tension in the wire.

View SolutionConsider the situation shown in figure. The wire which has a mass of 4.00 g oscillates in its second harmonic and sets the air column in the tube into vibrations in its fundamental mode. Assuming that the speed of sound in air is 40ms−1, find the tension in the wire.
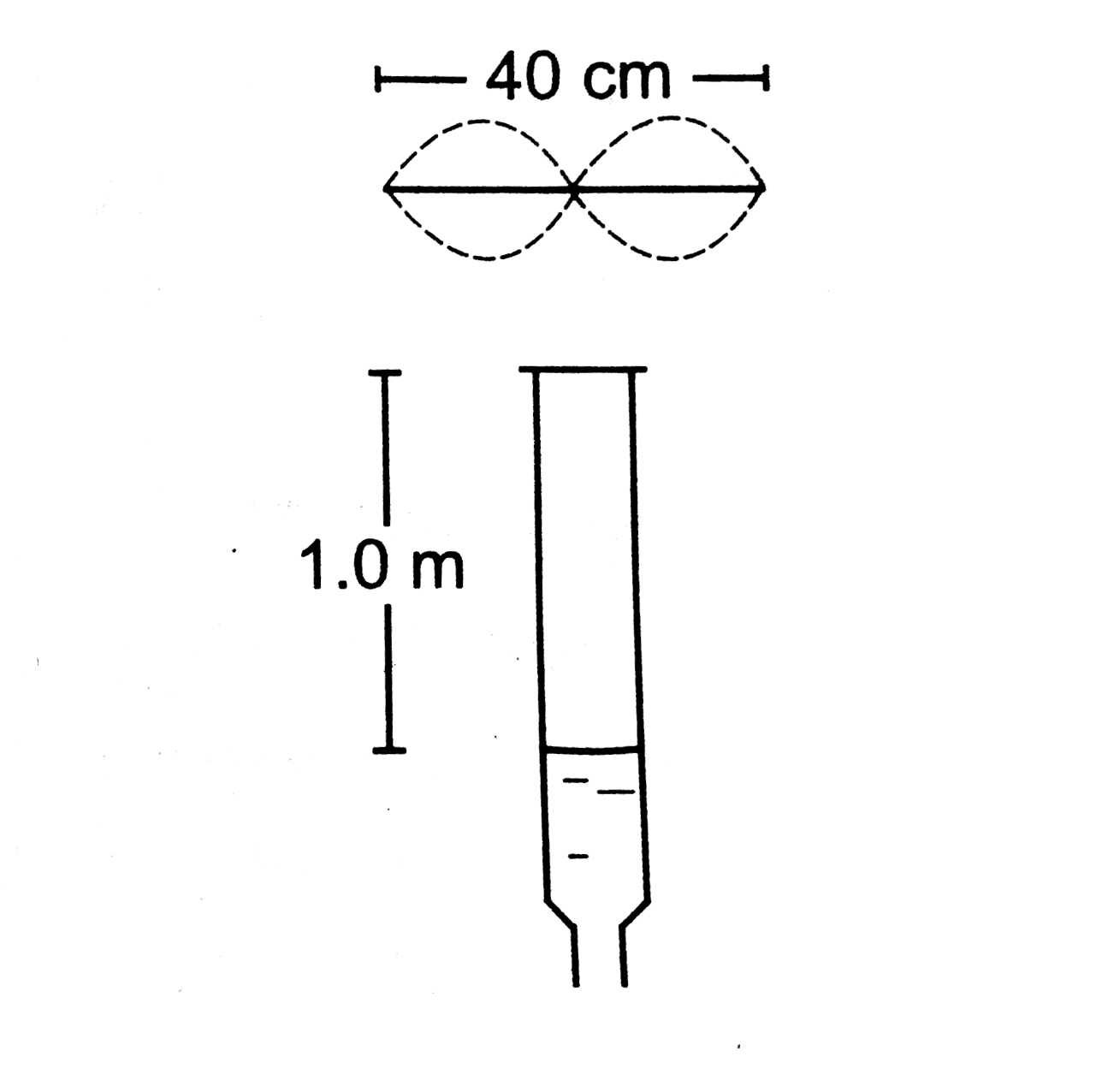 .
.
View SolutionIf the fundamental frequency of a pipe closed at one is 512HZ . The frequency of a pipe of the same dimension but open at both ends will be
View SolutionFind the fundamental frequency and the frequency of the third overtone of a pipe 45 cm long. If the pipe is open at both ends (use V=344m/s)
View SolutionTo decrease the fundamental frequency of a stretched string fixed at both ends one might
View SolutionA string 2.0 m long and fixed at its ends is driven by a 240 Hz vibrator. The string vibrates in its third harmonic mode. The speed of the wave and its fundamental frequency is
View Solution
