When equal volumes of two substance are mixed, the specific gravity of the mixurie is 4. When equal weights of the same substance are mixed, the specific gravity of the mixture is 3. The soecufuc gravities of the two substance could be
6 and 2
3and 4
2.5 and 3.5
5 and 3
The correct Answer is:A
To solve the problem, we need to find the specific gravities (or densities) of two substances based on the information given about their mixtures.
1. Understanding Specific Gravity:
- Specific gravity (SG) is defined as the ratio of the density of a substance to the density of water.
- If we denote the specific gravities of the two substances as SG1 and SG2, we can relate them to their densities as follows:
SG1=D1Dw,SG2=D2Dw
where Dw is the density of water.
2. Mixing Equal Volumes:
- When equal volumes of the two substances are mixed, the specific gravity of the mixture is given as 4.
- The formula for the specific gravity of the mixture when equal volumes are mixed is:
SGmix1=D1+D22Dw=4
- Rearranging gives:
D1+D2=8Dw(Equation 1)
3. Mixing Equal Weights:
- When equal weights of the two substances are mixed, the specific gravity of the mixture is given as 3.
- The formula for the specific gravity of the mixture when equal weights are mixed is:
SGmix2=21D1+1D2=3
- Rearranging gives:
2D1D2D1+D2=3Dw(Equation 2)
4. Substituting Equation 1 into Equation 2:
- From Equation 1, we have D1+D2=8Dw.
- Substitute this into Equation 2:
2D1D28Dw=3Dw
- Cross-multiplying gives:
2D1D2=24D2w
- Simplifying gives:
D1D2=12D2w(Equation 3)
5. Solving the Equations:
- Now we have two equations:
- D1+D2=8Dw (Equation 1)
- D1D2=12D2w (Equation 3)
- Let D1 and D2 be the roots of the quadratic equation:
x2−(D1+D2)x+D1D2=0
- Substituting from Equations 1 and 3:
x2−8Dwx+12D2w=0
- Solving this quadratic equation using the quadratic formula:
x=8Dw±√(8Dw)2−4⋅12D2w2
- Simplifying gives:
x=8Dw±√64D2w−48D2w2=8Dw±√16D2w2=8Dw±4Dw2
- This results in:
x=6Dworx=2Dw
- Thus, the specific gravities are:
SG1=6,SG2=2
Conclusion:
The specific gravities of the two substances are 6 and 2.
- Specific gravity (SG) is defined as the ratio of the density of a substance to the density of water.
- If we denote the specific gravities of the two substances as SG1 and SG2, we can relate them to their densities as follows:
SG1=D1Dw,SG2=D2Dw
where Dw is the density of water.
- When equal volumes of the two substances are mixed, the specific gravity of the mixture is given as 4.
- The formula for the specific gravity of the mixture when equal volumes are mixed is:
SGmix1=D1+D22Dw=4
- Rearranging gives:
D1+D2=8Dw(Equation 1)
- When equal weights of the two substances are mixed, the specific gravity of the mixture is given as 3.
- The formula for the specific gravity of the mixture when equal weights are mixed is:
SGmix2=21D1+1D2=3
- Rearranging gives:
2D1D2D1+D2=3Dw(Equation 2)
- From Equation 1, we have D1+D2=8Dw.
- Substitute this into Equation 2:
2D1D28Dw=3Dw
- Cross-multiplying gives:
2D1D2=24D2w
- Simplifying gives:
D1D2=12D2w(Equation 3)
- Now we have two equations:
- D1+D2=8Dw (Equation 1)
- D1D2=12D2w (Equation 3)
- Let D1 and D2 be the roots of the quadratic equation:
x2−(D1+D2)x+D1D2=0
- Substituting from Equations 1 and 3:
x2−8Dwx+12D2w=0
- Solving this quadratic equation using the quadratic formula:
x=8Dw±√(8Dw)2−4⋅12D2w2
- Simplifying gives:
x=8Dw±√64D2w−48D2w2=8Dw±√16D2w2=8Dw±4Dw2
- This results in:
x=6Dworx=2Dw
- Thus, the specific gravities are:
SG1=6,SG2=2
The specific gravities of the two substances are 6 and 2.
Topper's Solved these Questions
- FLUID MECHANICSBook:DC PANDEY ENGLISHChapter:FLUID MECHANICSExercise:Level 1 Subjective
Explore 36 Videos
- FLUID MECHANICSBook:DC PANDEY ENGLISHChapter:FLUID MECHANICSExercise:Subjective Question
Explore 2 Videos
- FLUID MECHANICSBook:DC PANDEY ENGLISHChapter:FLUID MECHANICSExercise:Assertion and Reason
Explore 1 Video
- EXPERIMENTSBook:DC PANDEY ENGLISHChapter:EXPERIMENTSExercise:Subjective
Explore 15 Videos
- GENERAL PHYSICSBook:DC PANDEY ENGLISHChapter:GENERAL PHYSICSExercise:INTEGER_TYPE
Explore 2 Videos
Similar Questions
Ten litres of a liquid of specific gravity 1.3 is mixed with 6 litres of a liquid of specific gravity 0.8 the specific gravity of mixture is
View SolutionWhen two substances of specific gravities d1 and d2 are mixed in equal volumes the specific garvity of mixture is found to be ρ1. When they are mixed in equal masses, the specific gravity of the mixture is found to be ρ2.(d1≠d2)
View Solution
Knowledge Check
Two moles of oxygen are mixed with eight moles of helium. The effective specific heat of the mixture at constant volume is
A1.3RB1.4RC1.7RD1.9R
If equal masses of two liquids of densities d1andd2 are mixed together, the density of the mixture is
View SolutionWhen equal volumes of pH =4 and pH=6 are mixed together then th ph of the resulting solution will be [log 5 =0.7]
View SolutionEqual masses of two substance of densities ρ1andρ2 are mixed together. What is the density of the mixture?
View SolutionTwo liquids are at temperatures 20∘Cand40∘C. When same mass of both of them is mixed, the temperature of the mixture is 32∘C. What is the ratio of their specific heats?
View SolutionEqual volumes of two HCl solutions of pH=3 and pH=5 were mixed. What is the pH of the resulting solution ?
View SolutionEqual volumes of two HCl solutions of pH=3 and pH=5 were mixed. What is the Ph of the resulting solution ?
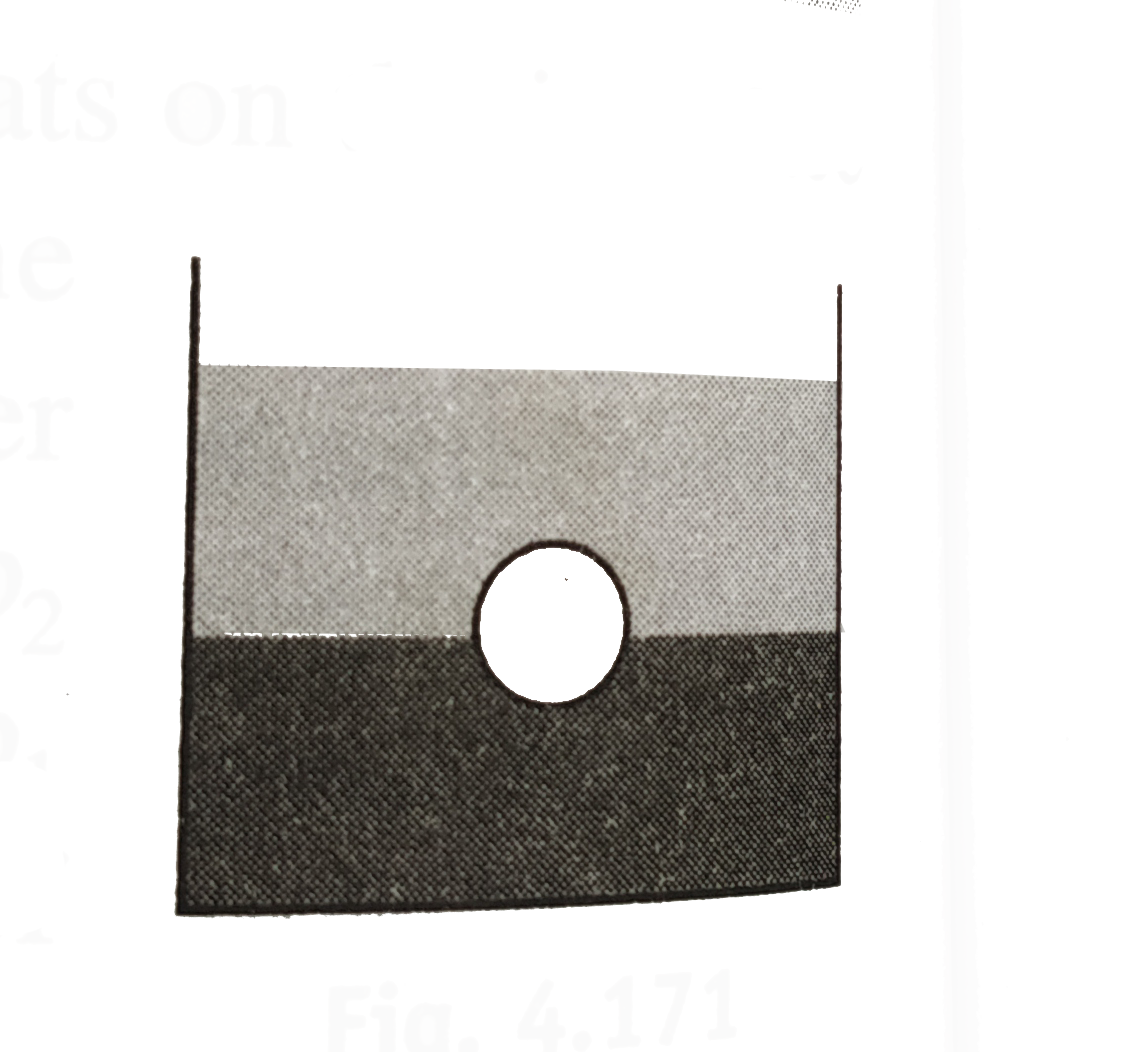
View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids (see the figure). The specific gravity of the upper liquid is ρ1 and that of lower one is ρ2 and the specific gravity of ball is ρ(ρ1>ρ>ρ2) The fraction of the volume the ball in the u of upper liquid is
View Solution