Three point masses 'm' each are placed at the three vertices of an equilateral traingle of side 'a'. Find net gravitational force on any point mass.
The correct Answer is:A, B, C, D
To find the net gravitational force on any point mass placed at one vertex of an equilateral triangle formed by three point masses, we can follow these steps:
Step 1: Identify the Configuration
We have three point masses, each of mass 'm', placed at the vertices of an equilateral triangle with side length 'a'. Let's label the vertices as A, B, and C.
Step 2: Calculate the Gravitational Force Between the Masses
The gravitational force between any two point masses is given by Newton's law of gravitation:
F=Gm1m2r2
In our case, the force between any two masses (e.g., mass at A and mass at C) is:
FAC=Gm2a2
Similarly, the force between mass at B and mass at C is:
FBC=Gm2a2
Step 3: Determine the Directions of the Forces
The forces FAC and FBC act along the lines connecting the masses. The angle between these two forces is 60 degrees because the triangle is equilateral.
Step 4: Resolve the Forces into Components
To find the net gravitational force acting on the mass at vertex C, we can resolve the forces FAC and FBC into their x and y components.
- The x-component of FAC (acting towards A) is:
FACx=FAC⋅cos(60∘)=Gm2a2⋅12=Gm22a2
- The y-component of FAC is:
FACy=FAC⋅sin(60∘)=Gm2a2⋅√32=√3Gm22a2
- The x-component of FBC (acting towards B) is:
FBCx=FBC⋅cos(60∘)=Gm2a2⋅12=Gm22a2
- The y-component of FBC is:
FBCy=FBC⋅sin(60∘)=Gm2a2⋅√32=√3Gm22a2
Step 5: Sum the Components
Now, we sum the x and y components to find the net force on mass C.
- The total x-component of the net force Fnet,x:
Fnet,x=FACx+FBCx=Gm22a2+Gm22a2=Gm2a2
- The total y-component of the net force Fnet,y:
Fnet,y=FACy+FBCy=√3Gm22a2+√3Gm22a2=√3Gm2a2
Step 6: Calculate the Magnitude of the Net Force
The magnitude of the net gravitational force Fnet can be calculated using the Pythagorean theorem:
Fnet=√(Fnet,x)2+(Fnet,y)2
Substituting the values we found:
Fnet=√(Gm2a2)2+(√3Gm2a2)2
=√G2m4a4+3G2m4a4=√4G2m4a4=2Gm2a2
Final Answer
Thus, the net gravitational force on any point mass placed at one vertex of the triangle is:
Fnet=2Gm2a2
We have three point masses, each of mass 'm', placed at the vertices of an equilateral triangle with side length 'a'. Let's label the vertices as A, B, and C.
The gravitational force between any two point masses is given by Newton's law of gravitation:
The forces FAC and FBC act along the lines connecting the masses. The angle between these two forces is 60 degrees because the triangle is equilateral.
To find the net gravitational force acting on the mass at vertex C, we can resolve the forces FAC and FBC into their x and y components.
FACx=FAC⋅cos(60∘)=Gm2a2⋅12=Gm22a2
FACy=FAC⋅sin(60∘)=Gm2a2⋅√32=√3Gm22a2
FBCx=FBC⋅cos(60∘)=Gm2a2⋅12=Gm22a2
FBCy=FBC⋅sin(60∘)=Gm2a2⋅√32=√3Gm22a2
Now, we sum the x and y components to find the net force on mass C.
Fnet,x=FACx+FBCx=Gm22a2+Gm22a2=Gm2a2
Fnet,y=FACy+FBCy=√3Gm22a2+√3Gm22a2=√3Gm2a2
The magnitude of the net gravitational force Fnet can be calculated using the Pythagorean theorem:
Thus, the net gravitational force on any point mass placed at one vertex of the triangle is:
Topper's Solved these Questions
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Solved Examples
16 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - Miscellaneous Examples
8 Videos
- GENERAL PHYSICSBOOK - DC PANDEY ENGLISHCHAPTER - GENERAL PHYSICSEXERCISE - INTEGER_TYPE
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - INTEGER_TYPE
10 Videos
Similar Questions
Three equal masses of 1 kg each are placed at the vertices of an equilateral triangle of side 1 m, then the gravitational force on one of the masses due to other masses is (approx.)
View SolutionTwo points masses m each are kept at the two verticle of an equilateral triangle of side 'a' as shows in figure.
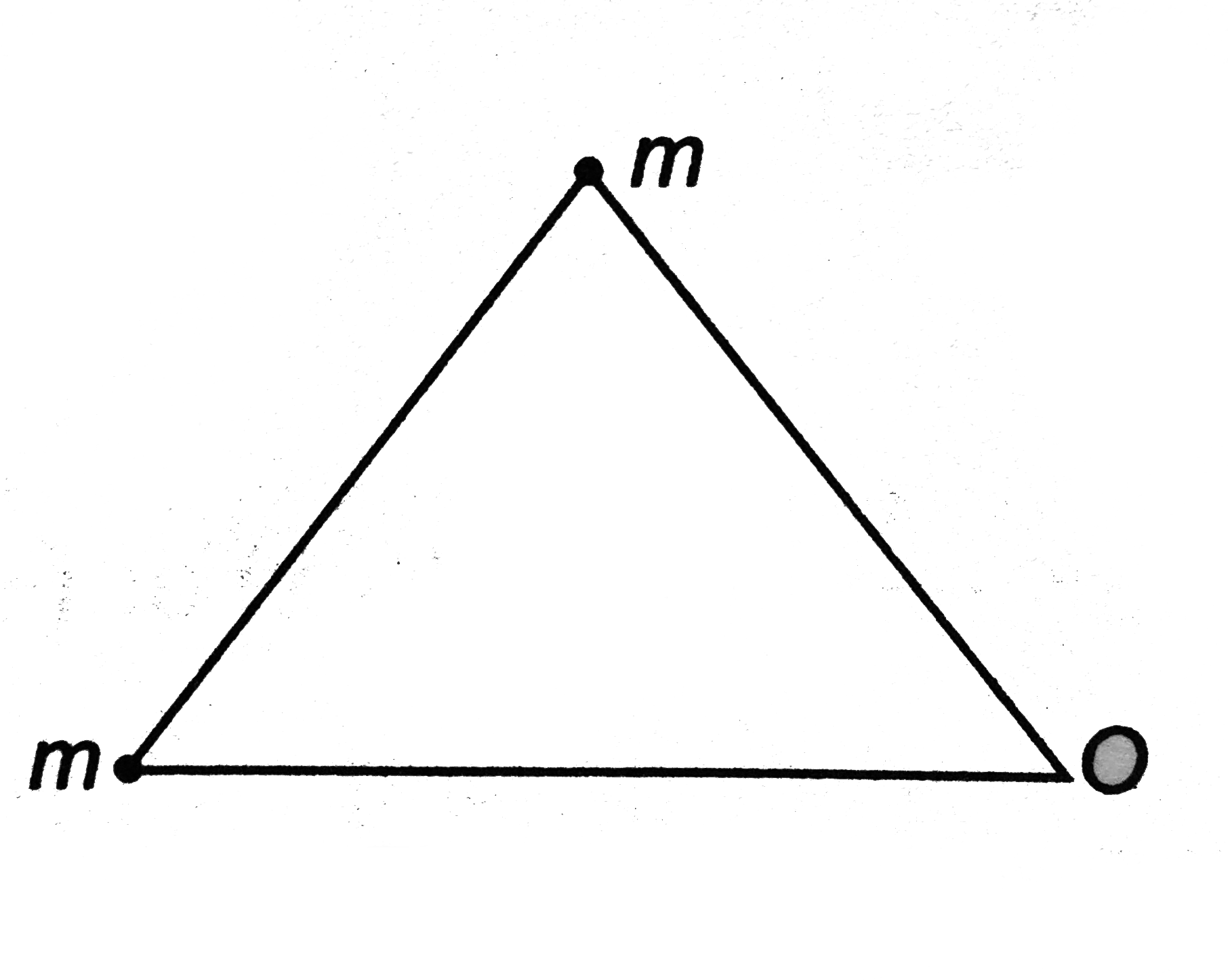
Find gravitational potential and magnitude of field strength at O.
View SolutionThree mass points each of mass m are placed at the vertices of an equilateral triangle of side 1. What is the gravitational field and potential due to the three masses at the centroid of the triangle ?
View SolutionThree mass points each of mass m are placed at the vertices of an equilateral tringale of side l. What is the gravitational field and potential due to three masses at the centroid of the triangle ?
View SolutionThree point charges q are placed at three vertices of an equilateral triangle of side a. Find magnitude of electric force on any charge due to the other two.
View SolutionThree particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionThree particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionThree particle of mass m each are placed at the three corners of an equilateral triangle of side a. Find the work which should be done on this system to increase the sides of the triangle to 2a.
View SolutionThree masses of 1kg, 2kg, and 3kg, are placed at the vertices of an equilateral triangle of side 1m. Find the gravitational potential energy of this system.
Take G=6.67×10−11N−m2/kg2.
View SolutionThree masses of 1kg, 2kg, and 3kg, are placed at the vertices of an equilateral triangle of side 1m. Find the gravitational potential energy of this system.
Take G=6.67×10−11N−m2/kg2.
View Solution
