A cylinder having radius 0.4 m initially rotating (at r=0) with ω0=54rad/s is placed on a rough inclinded plane with θ=37∘ having friction coefficient μ=0.5 the time taken by the cylinder to start pure rolling is (g=10m/s2)

5.4 s
2.4 s
1.4 s
none of these
a=(μcosθ)+(gsinθ)
=0.5×10×0.8+10×0.6=10m/s2
α=(μmgcosθ)R)12mR2=2μgcosθR
=2×0.5×10×0.80.4=20rad/s2
Pure rolling will start when
v=Rω or at =R(ω0−αt)
∴10t=0.4(54−20t)
Solving this equation we get
t=1.2s
Topper's Solved these Questions
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Level 2 Multiple Correct
Explore 36 Videos
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Level 2
Explore 1 Video
- ROTATIONAL MECHANICSBook:DC PANDEY ENGLISHChapter:ROTATIONAL MECHANICSExercise:Level 1 Subjective
Explore 32 Videos
- ROTATIONBook:DC PANDEY ENGLISHChapter:ROTATIONExercise:(C) Chapter Exercises
Explore 39 Videos
- ROTATIONAL MOTIONBook:DC PANDEY ENGLISHChapter:ROTATIONAL MOTIONExercise:Integer Type Questions
Explore 17 Videos
Similar Questions
A uniform solid sphere of mass m and radius 'R' is imparted an initial velocity v0 and angular ω0=2v0R and then placed on a rough inclined plane of inclination 'theta' and coefficient of friction μ=2tanθ as shown. The time after which the sphere will start rolling without slipping is

View SolutionA disc of mass m and radius R rotating with angular speed ω0 is placed on a rough surface (co-officient of friction =μ). Then

View Solution
Knowledge Check
A ring of radius R is rotating with an angular speed ω0 about a horizontal axis. It is placed on a rough horizontal table. The coefficient of kinetic friction is μk. The time after it starts rolling is.
AωoμkR2gBωog2μkRC2ωoRμkgDωoR2μkg
A cylinder of mass m radius R is spined to a clockwise angular velocity ωo and then gently placed on an inclined plane for which coefficient of friction μ=tanθ,θ is the angle of inclined plane with the horizontal. The centre of mass of cylinder will remain stationary for time

View SolutionIf a solid cylinder rolls without slipping on an inclined plane of inclination '0' then the minimum coefficient of friction required to support pure rolling is
View SolutionA hollow sphere of mass m and radius R is rolling downdard on a rough inclined plane of inclination θ. If the coefficient of friction between the hollow sphere and incline is μ, then
View SolutionA sphere of radius R is projected with a reverse spin ω0 down a rough inclined plane with a speed v0 for which coefficient of friction is μ>tanθ, where θ is angle of the inclination. Show that it will turn back if
ω>5v0μ2R(μ−tanθ)

View SolutionA block of mass 10 kg is released on rough incline plane. Block start descending with acceleration 2m/s2. Kinetic friction force acting on block is (take g=10m/s2)
View SolutionA solid sphere of radius 2.45m is rotating with an angular speed of 10rad/s. When this rotating sphere is placed on a rough horizontal surface then after sometime it starts pure rolling. Find the linear speed of the sphere after it starts pure rolling.
View SolutionA thin but very large plank of mass 2 m is placed on a horziontal smooth surface. A solid cylinder of mass m and radius r is given only translational velocity v0 and gently placed on the plank as shown in the figure. The coefficient of kinetic friciton between the plank and the cylinder is μ.
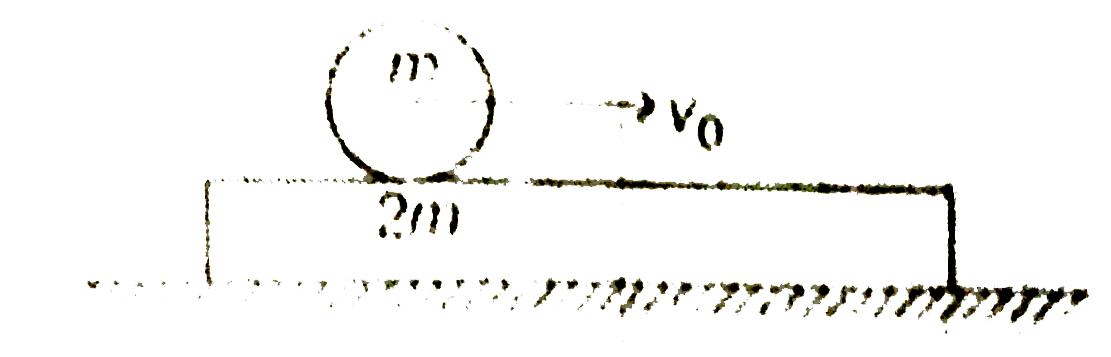
the cylinder given impluse t=0, than at what time pure rolling starts
View Solution