Question

Two identical discs are positioned on a vertical axis as shown in the figure. The bottom disc is rotating at angular velocity ${\omega }_0$ and has rotational kinetic energy $K_0$.The top disc is initially at rest. It then falls and sticks to the bottom disc. The change in the rotational kinetic energy of the system is

Options

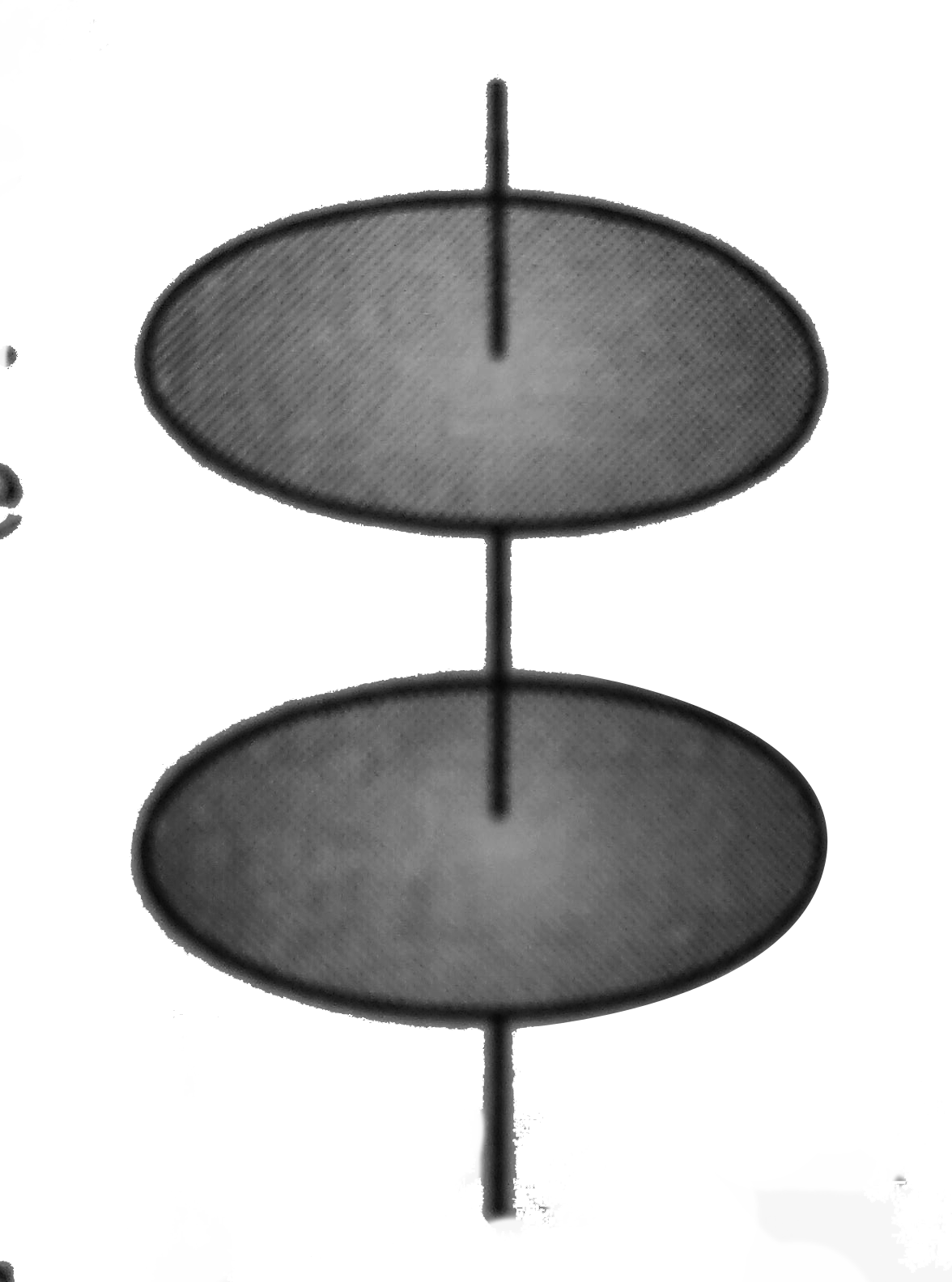
A. `K_(0)//2`
B. `-K_(0)//2`
C. `-K_(0)//4`
D. `K_(0)//4`

Explanation

`K=(L^(2))/(2I)` or `Kprop(1)/(I)` As `I` has doubled, so `K` will become half.