Three particles, each of the mass m are situated at the vertices of an equilateral triangle of side a. The only forces acting on the particles are their mutual gravitational forces. It is desired that each particle moves in a circle while maintaining the original mutual separation a. Find the initial velocity that should be given to each particle and also the time period of the circular motion. (F=Gm1m2r2)
The correct Answer is:A, B, C
To solve the problem of finding the initial velocity and time period of the circular motion of three particles situated at the vertices of an equilateral triangle, we will follow these steps:
Step 1: Understand the Forces Acting on the Particles
Each particle experiences gravitational forces due to the other two particles. The gravitational force between any two particles is given by:
F=Gm2a2
where G is the gravitational constant, m is the mass of each particle, and a is the distance between the particles.
Step 2: Calculate the Resultant Gravitational Force
Since the particles are at the vertices of an equilateral triangle, the angle between the forces acting on one particle due to the other two is 60∘. Therefore, we can calculate the resultant force Fr acting on one particle using the law of cosines:
Fr=√F2+F2+2F2cos(60∘)=√2F2+2F2⋅12=√3F2=F√3
Step 3: Relate the Resultant Force to Centripetal Force
The resultant gravitational force Fr provides the necessary centripetal force for circular motion. The centripetal force Fc required for circular motion is given by:
Fc=mv2R
where v is the velocity of the particle and R is the radius of the circular path.
Step 4: Set the Forces Equal
Since the gravitational force provides the centripetal force, we can set them equal:
F√3=mv2R
Substituting F=Gm2a2:
Gm2a2√3=mv2R
Step 5: Simplify the Equation
We can cancel one m from both sides:
Gm√3a2=v2R
Rearranging gives:
v2=Gm√3Ra2
Step 6: Find the Relation Between R and a
To find R, we can drop a perpendicular from the center of the triangle to one side. The height of the triangle is given by:
h=√32a
The radius R of the circular path is:
R=a√3
Step 7: Substitute R into the Velocity Equation
Substituting R into the velocity equation:
v2=Gm√3(a√3)a2=Gma
Thus, the initial velocity v is:
v=√Gma
Step 8: Calculate the Time Period of Circular Motion
The time period T of circular motion is given by:
T=2πRv
Substituting R and v:
T=2π(a√3)√Gma
Simplifying gives:
T=2πa3/2√3Gm
Final Results
- The initial velocity v that should be given to each particle is:
v=√Gma
- The time period T of the circular motion is:
T=2πa3/2√3Gm
Each particle experiences gravitational forces due to the other two particles. The gravitational force between any two particles is given by:
Since the particles are at the vertices of an equilateral triangle, the angle between the forces acting on one particle due to the other two is 60∘. Therefore, we can calculate the resultant force Fr acting on one particle using the law of cosines:
The resultant gravitational force Fr provides the necessary centripetal force for circular motion. The centripetal force Fc required for circular motion is given by:
Since the gravitational force provides the centripetal force, we can set them equal:
We can cancel one m from both sides:
To find R, we can drop a perpendicular from the center of the triangle to one side. The height of the triangle is given by:
Substituting R into the velocity equation:
The time period T of circular motion is given by:
- The initial velocity v that should be given to each particle is:
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Solved Examples2videos
CIRCULAR MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Miscellaneous Examples5videos
CIRCULAR MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Subjective21videos
CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- BOOK - DC PANDEY ENGLISHCHAPTER - COMMUNICATION SYSTEMEXERCISE - Only One Option is Correct27videos
COMMUNICATION SYSTEM
Similar Questions
Three particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
The gravitational potential at O isView SolutionThree particles each of mass m are kept at vertices of an equilateral triangle of side L. The gravitational field at centre due to these particle is
View Solution
Knowledge Check
- Question 1 - Select One
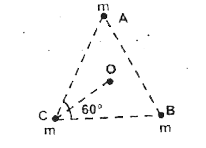
Three masses each of mass m are palced at the vertices of an equilateral triangles ABC of side l as shown in figure. The force acting on a mass 2m placed at the centroid O of the triangle is
 AzeroB3Gm2l2C5Gm2l2D7Gm2l2
AzeroB3Gm2l2C5Gm2l2D7Gm2l2
Three particles, each of mass m are fixed at the vertices of an equilateral triangle of side length a. The only forces acting on the particles are their mutual gravitational forces. Then answer the following questions.
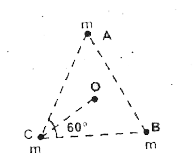
Force acting on particle C, due to particle A and BView SolutionThree particles of equal mass 'm' are situated at the vertices of an equilateral triangle of side L. The work done in increasing the side of the triangle to 2L is
View SolutionThree particle each of mass m are placed at the corners of equilateral triangle of side l

Which of the following is/are correct ?View SolutionThree point masses 'm' each are placed at the three vertices of an equilateral traingle of side 'a'. Find net gravitational force on any point mass.
View SolutionThree equal masses of 1.5 kg each are fixed at the vertices of an equilateral triangle of side 1 m. What is the force acting on a particle of mass 1 kg placed at its centroid ?
View SolutionThree particles, each of mass m are situated at the vertices of an equilateral triangle ABC of side L as shown in the figure. Find the moment of inertia of the system about the line AX perpendicular to AB in the plane of ABC
 View Solution
View SolutionThree particles, each of mass m are situated at the vertices of an equilateral triangle ABC of side l (as shown in the figure).
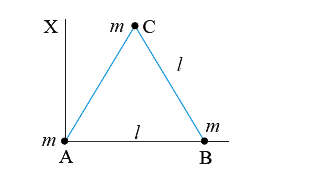
The moment of inertia of the system about a line AX perpendicular to AB and in the plane of ABC, will be:View Solution
