Find the speed of both the blocks at the moment the block m2 hits the wall AB, after the blocks are released from rest. Given that m1=0.5kg and m2=2kg,(g=10m/s2)
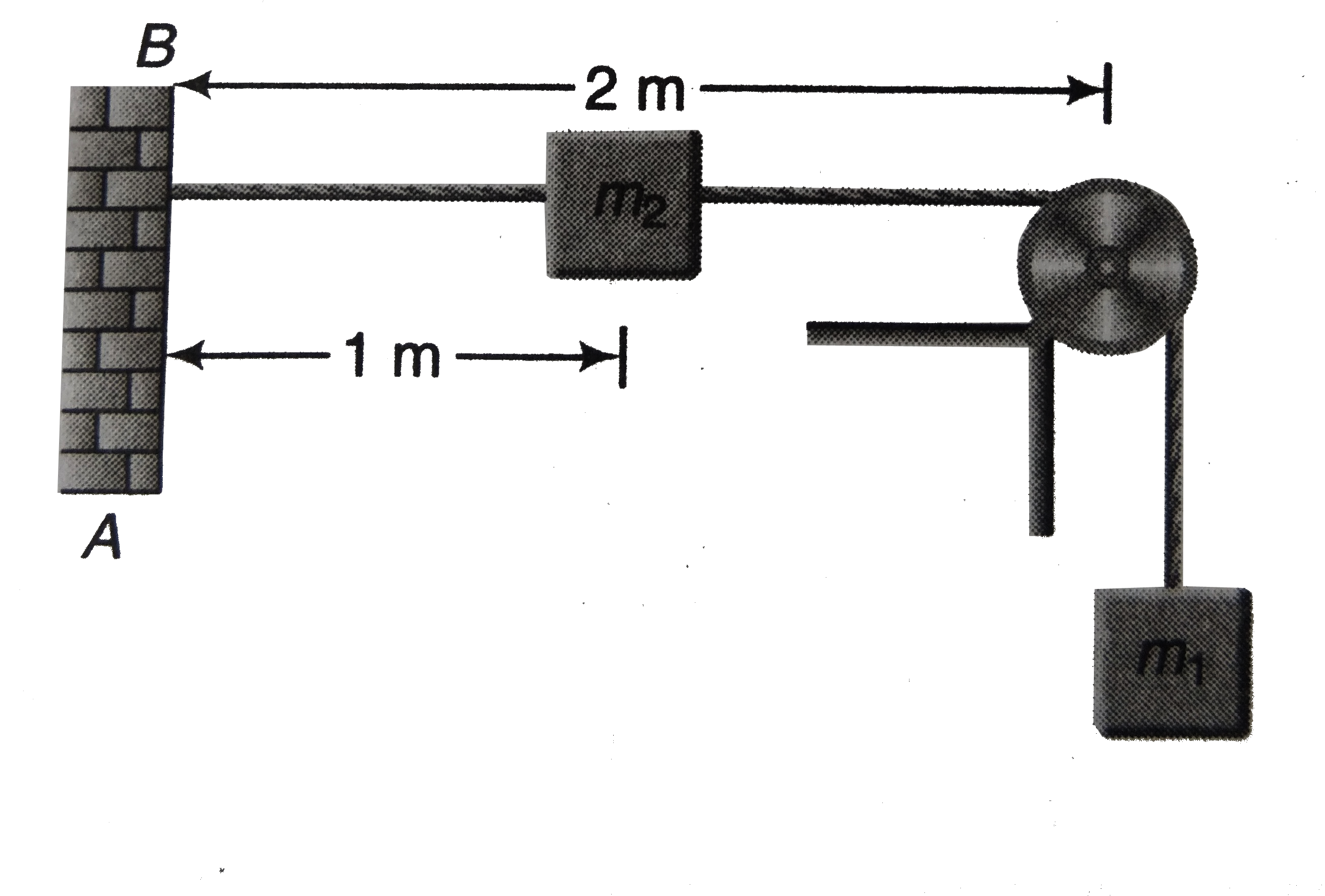 .
.
The correct Answer is:A, B, C
From conservation of energy ,
m2gh2=m1gh+(1)+12m1v1_(2)+12m2v(1)2
Here, v1=v2cosθ
:. 2×10×1=(0.5)(10)(√5−1)+12×0.5
xv22×(2√5)2+12×2×v22
∴20=6.18+0.2v22+v22
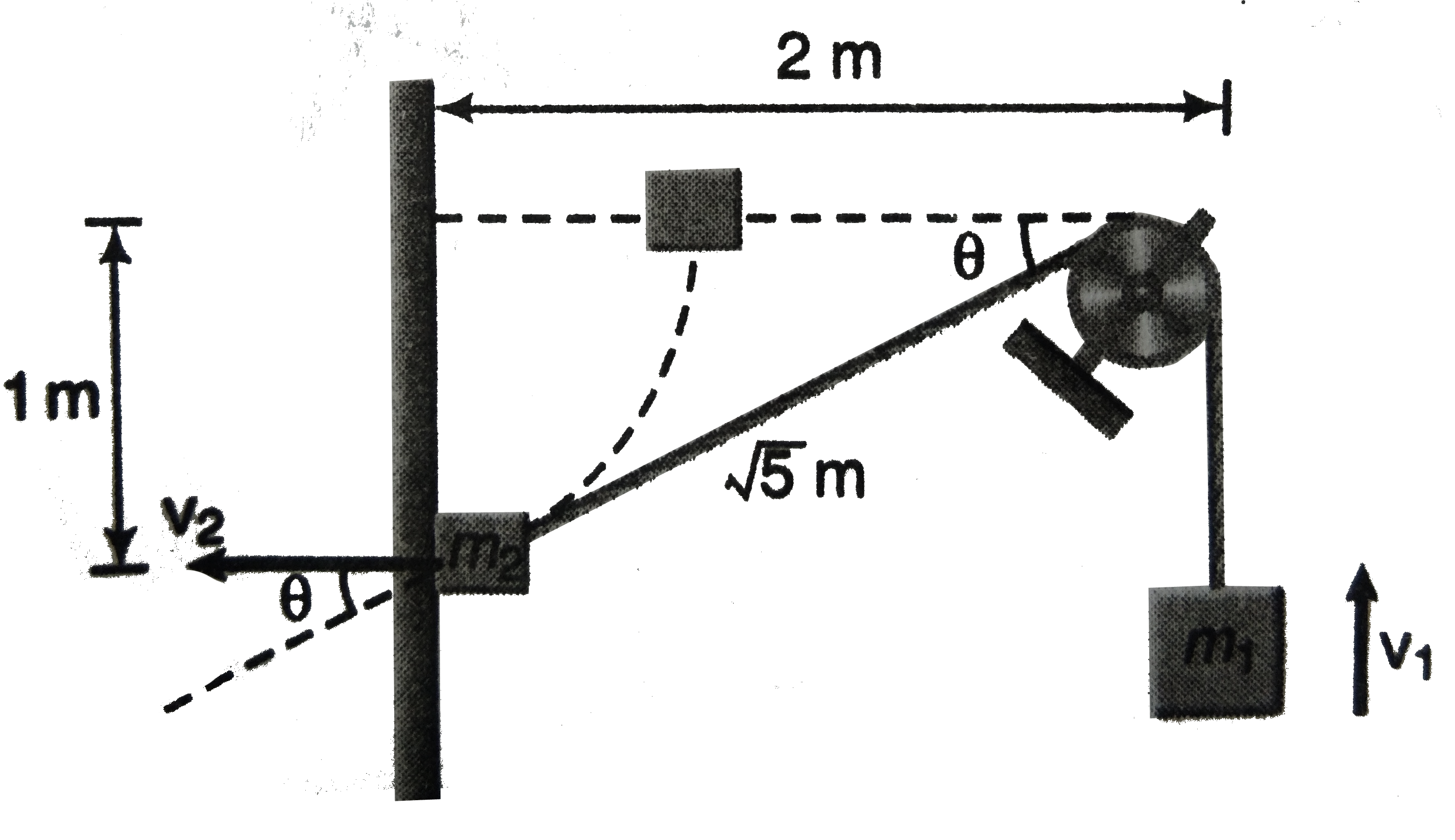
v2=3.39m/s
and v1=v2cosθ=2√5×3.39
or v1=3.03m/s,
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based
2 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 More Than One Correct
9 Videos
- WAVE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
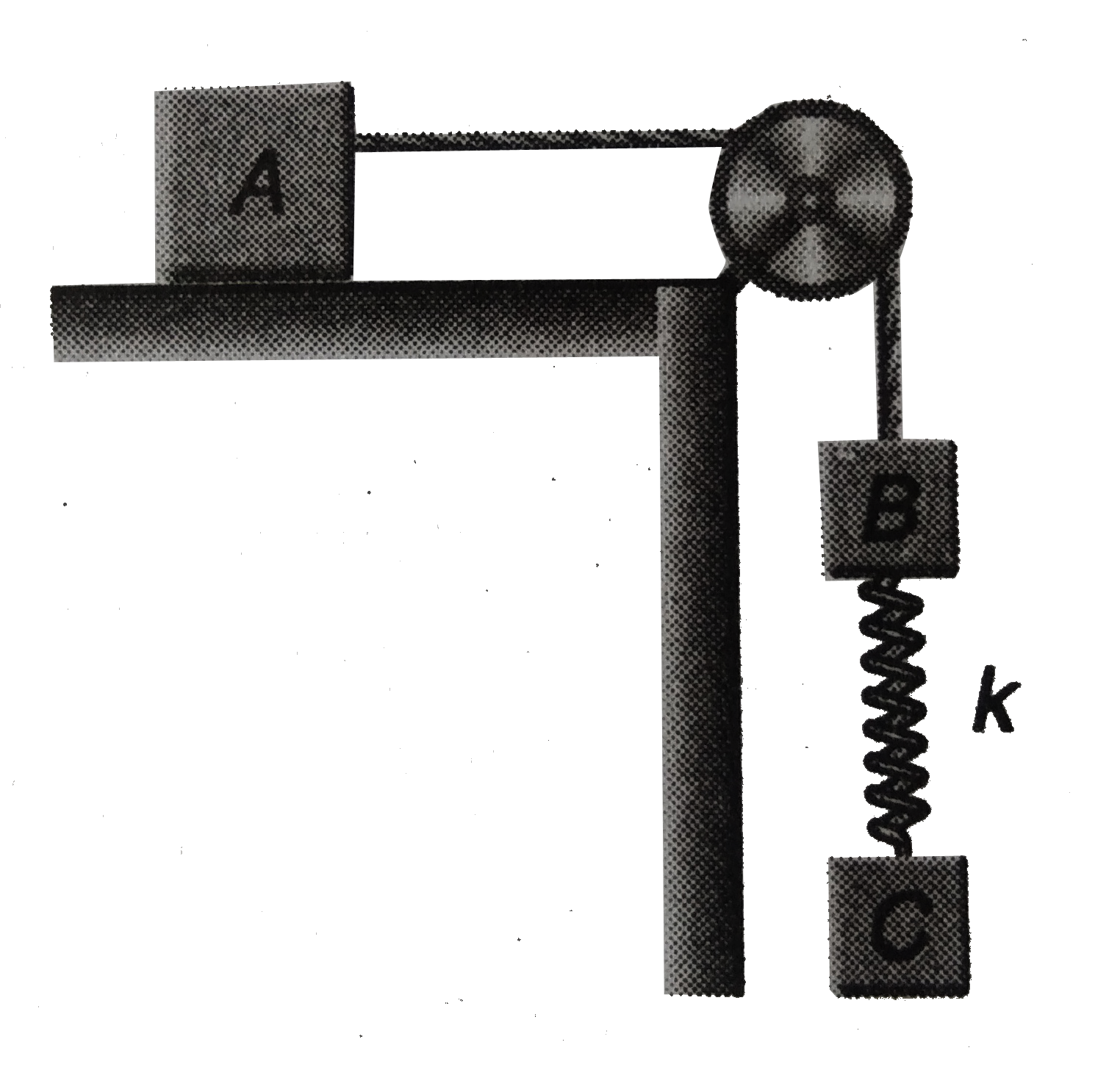
In the figure shown masses of the blocks A, B and C are 6kg, 2kg and 1kg respectively. Mass of the spring is negligibly small and its stiffness is 1000 N//m. The coefficient of friction between the block A and the table is μ=0.8. Initially block C is held such that spring is in relaxed position. The block is released from rest. Find (g=10m/s2).
View SolutionThe block of mass m1 is placed on a wedge of an angle θ, as shown. The block is moving over the inclined surface of the wedge. Friction coefficient between the block and the wedge is μ1, whereas it is μ2 between the wedge and the horizontal surface. if μ1=12,θ=45∘,m1=4kg,m2=5kg and g=10m/s2, find minimum value of μ2 so that the wedge remains stationary on the surface. express your answer in multiple of 10−3
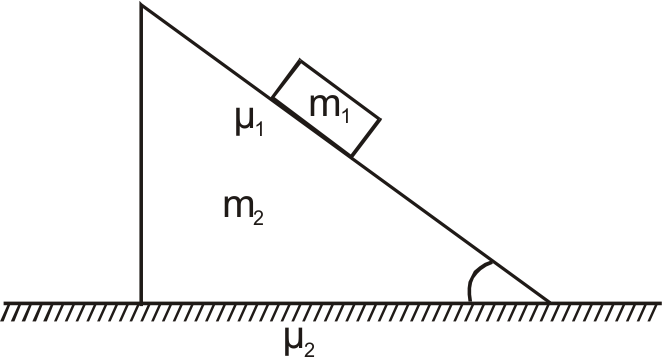
View SolutionThe block of mass m1 is placed on a wedge of an angle θ, as shown. The block is moving over the inclined surface of the wedge. Friction coefficient between the block and the wedge is μ1, whereas it is μ2 between the wedge and the horizontal surface. if μ1=12,θ=45∘,m1=4kg,m2=5kg and g=10m/s2, find minimum value of μ2 so that the wedge remains stationary on the surface. express your answer in multiple of 10−3

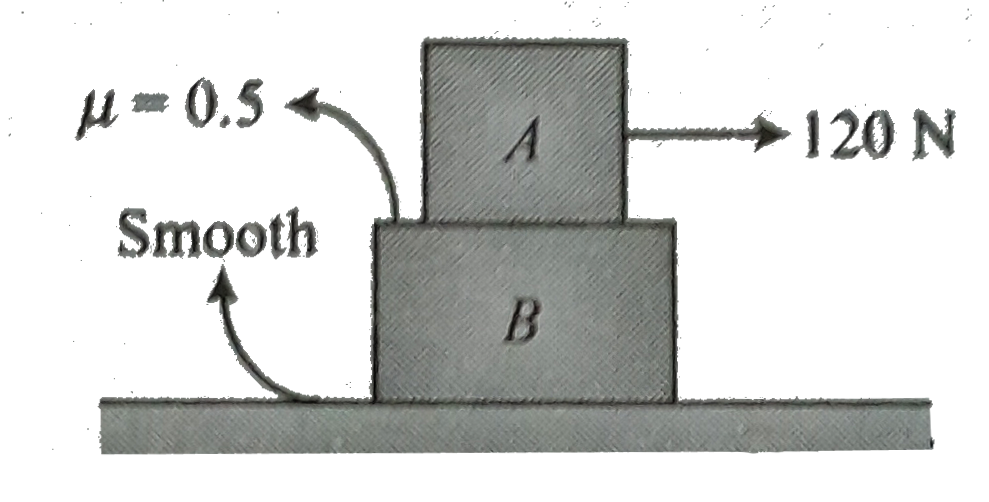
View SolutionFind the acceleration of the two blocks . The system is initialy at rest and the friction are as shown in fig .Given mA=mB=10kg
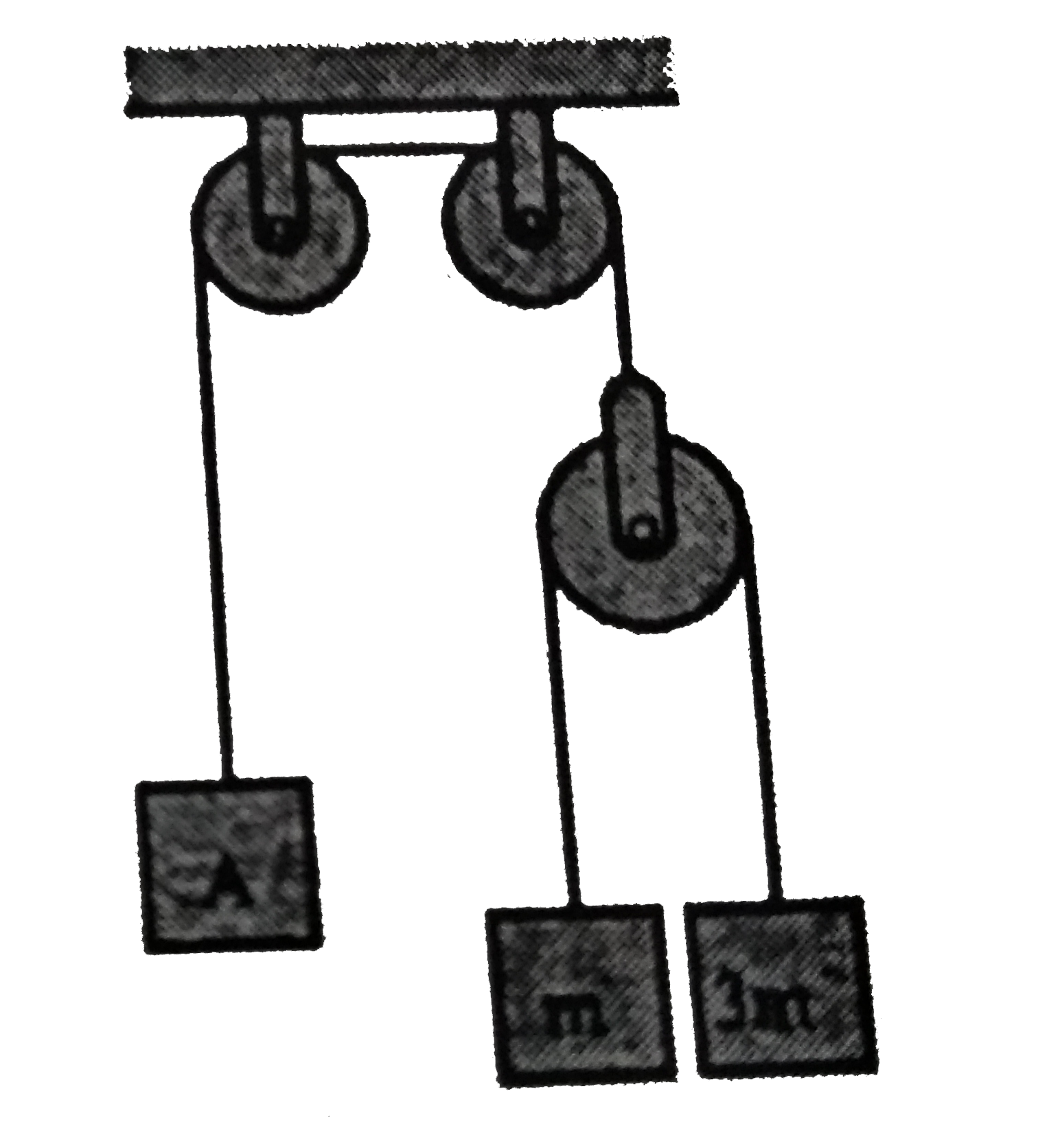
View SolutionIn the given figure, Find mass of the block A, if it remains at rest, when the system is released from rest. Pulleys and strings are massless. [g=10m/s2]
View SolutionA block B of mass M is suspended from a cord of length l attached to a cart A of mass M as shown in the figure. The horizontal surface on which the cart moves is smooth. Initially both the cart and the block are at rest in the position shown. Now B is released. Take M=m=2kg,θ=45∘andg=10m/s2.
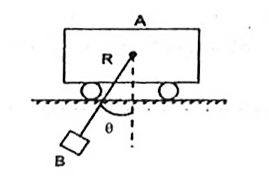
The magnitude of acceleration of B (with respect to cart) immediately after the system is released from rest is
View SolutionA block B of mass M is suspended from a cord of length l attached to a cart A of mass M as shown in the figure. The horizontal surface on which the cart moves is smooth. Initially both the cart and the block are at rest in the position shown. Now B is released. Take M=m=2kg,θ=45∘andg=10m/s2.
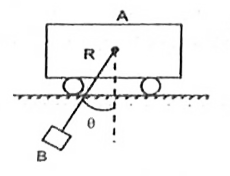
The acceleration of the cart immediately after the system is released from rest is
View SolutionA block B of mass M is suspended from a cord of length l attached to a cart A of mass M as shown in the figure. The horizontal surface on which the cart moves is smooth. Initially both the cart and the block are at rest in the position shown. Now B is released. Take M=m=2kg,θ=45∘andg=10m/s2.
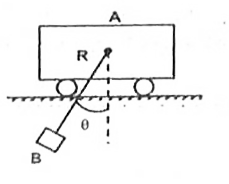
The tension in the cord immediately after the system is released from rest is
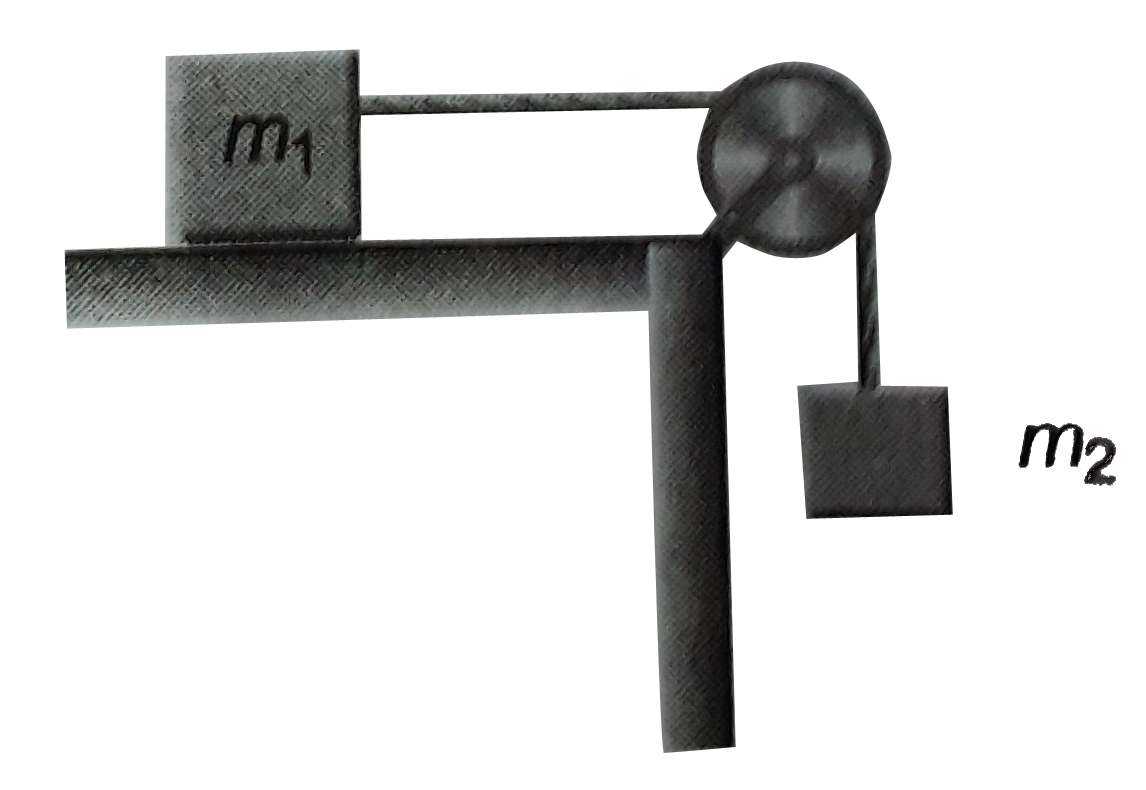
View SolutionTwo masses m1=10Kg and m2=5kg are connected by an ideal string as shown in the figure. The coefficient of friction between m1 and the surface is μ=0.2 Assuming that the system is released from rest calculate the velocity of blocks when m2 has descended by 4m. (g=10m/s2)
 .
.
View SolutionIn the figure shown co-efficient of friction between the block B and C is 0.4. There is no friction between the block C and hte surface on which it is placed. The system of blocks is released from rest in the shown situation. Find the distance moved by the block C when block A descends through a distance 2m. Given masses of the blocks are mA=3kg,mB=5kgandmC=10kg.

View Solution
