Question

Two persons of equal heights are carrying a long uniform wooden plank of length $l$ . They are at distance $\frac{l}{4}$ and $\frac{l}{6}$ from nearest end of the rod. The ratio of normal reation at their heads is

Options

A. `2:3`
B. `1:3`
C. `4:3`
D. `1:2`

Answer

C

Explanation

`x = (1)/(2) - (1)/(4) = (1)/(4)`

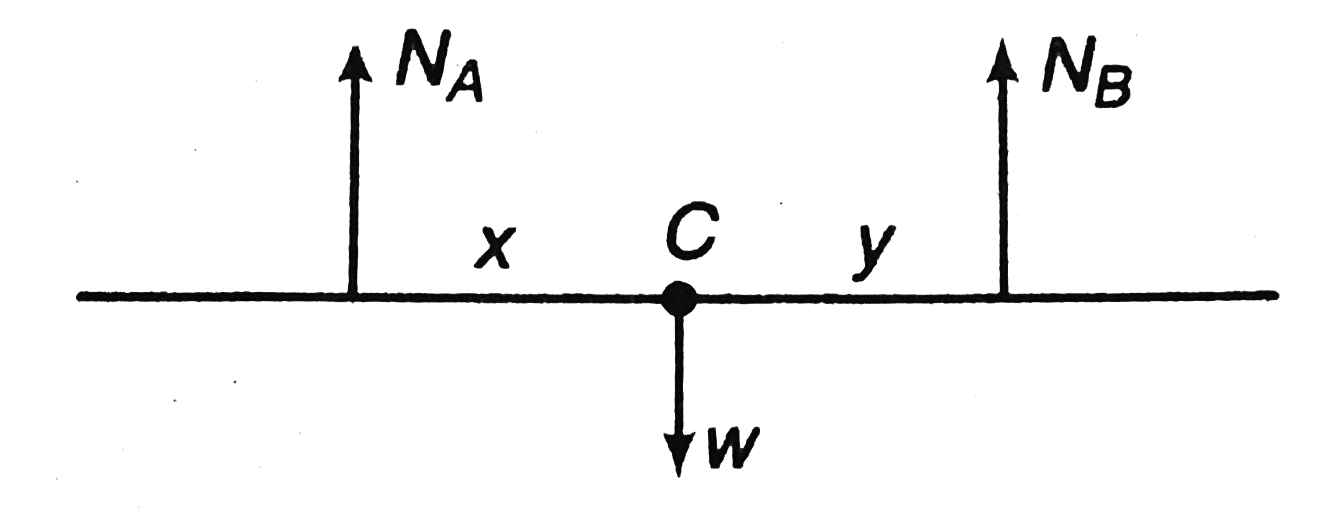
`y = (1)/(2) - (1)/(6) = (1)/(3)`
`sum` (moments of all forces about point` C) = 0`
`:. N_(A)x = N_(B)y`
or `(N_(A))/(N_(B)) = (y)/(x) = (4)/(3)`