In a wire V = 5.0V, I = 2.00A, L=10.0cm and diameter d=5.00mm. Evaluate
3.9
0.019
0.029
0.03
The correct Answer is:A
To solve the problem, we need to evaluate the maximum percentage error in resistivity, represented as (Δρ×100)/ρ. We will follow these steps:
Step 1: Understand the relationship between voltage, current, and resistance
According to Ohm's law, the resistance R can be expressed as:
R=VI
where V is the voltage and I is the current.
Step 2: Determine the resistivity formula
The resistivity ρ can be expressed in terms of resistance R and the physical dimensions of the wire:
ρ=R⋅AL
where A is the cross-sectional area and L is the length of the wire.
Step 3: Calculate the cross-sectional area A
The area A of the wire can be calculated using the diameter d:
A=πd24
Given that d=5.00mm=0.005m, we can calculate A:
A=π(0.005)24=π×0.0000254≈1.9635×10−5m2
Step 4: Substitute values into the resistivity formula
Now we can substitute the values of V, I, A, and L into the resistivity formula:
ρ=VI⋅AL
Given V=5.0V, I=2.0A, and L=10.0cm=0.1m:
ρ=5.02.0⋅1.9635×10−50.1=2.5⋅1.9635×10−4≈4.90875×10−4Ω⋅m
Step 5: Calculate the maximum percentage error in resistivity
The maximum percentage error in resistivity can be calculated using the formula:
Δρρ×100=ΔVV+ΔII+ΔLL+2ΔDD
Step 6: Determine the least counts
- For voltage V=5.0V, the least count ΔV=0.1V:
ΔVV=0.15.0=0.02
- For current I=2.0A, the least count ΔI=0.01A:
ΔII=0.012.0=0.005
- For length L=10.0cm, the least count ΔL=0.1cm=0.001m:
ΔLL=0.0010.1=0.01
- For diameter d=5.0mm, the least count ΔD=0.01mm=0.00001m:
ΔDD=0.000010.005=0.002
Step 7: Substitute the errors into the formula
Now substituting these values into the error formula:
Δρρ×100=0.02+0.005+0.01+2×0.002
Calculating this gives:
Δρρ×100=0.02+0.005+0.01+0.004=0.039 or 3.9%
Final Answer
The maximum percentage error in resistivity is approximately 3.9%.
---
According to Ohm's law, the resistance R can be expressed as:
R=VI
where V is the voltage and I is the current.
The resistivity ρ can be expressed in terms of resistance R and the physical dimensions of the wire:
ρ=R⋅AL
where A is the cross-sectional area and L is the length of the wire.
The area A of the wire can be calculated using the diameter d:
A=πd24
Given that d=5.00mm=0.005m, we can calculate A:
A=π(0.005)24=π×0.0000254≈1.9635×10−5m2
Now we can substitute the values of V, I, A, and L into the resistivity formula:
ρ=VI⋅AL
Given V=5.0V, I=2.0A, and L=10.0cm=0.1m:
ρ=5.02.0⋅1.9635×10−50.1=2.5⋅1.9635×10−4≈4.90875×10−4Ω⋅m
The maximum percentage error in resistivity can be calculated using the formula:
Δρρ×100=ΔVV+ΔII+ΔLL+2ΔDD
- For voltage V=5.0V, the least count ΔV=0.1V:
ΔVV=0.15.0=0.02
ΔII=0.012.0=0.005
ΔLL=0.0010.1=0.01
ΔDD=0.000010.005=0.002
Now substituting these values into the error formula:
Δρρ×100=0.02+0.005+0.01+2×0.002
Calculating this gives:
Δρρ×100=0.02+0.005+0.01+0.004=0.039 or 3.9%
The maximum percentage error in resistivity is approximately 3.9%.
Topper's Solved these Questions
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-A
Explore 80 Videos
- JEE MAIN 2021Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2021Exercise:SECTION-B
Explore 40 Videos
- JEE MAINBook:JEE MAINS PREVIOUS YEARChapter:JEE MAINExercise:All Questions
Explore 473 Videos
- JEE MAIN 2022Book:JEE MAINS PREVIOUS YEARChapter:JEE MAIN 2022Exercise:Question
Explore 492 Videos
Similar Questions
In the experiment of Ohm's law, when potential difference of 10.0 V is applied, current measured is 1.00 A. If length of wire is found to be 10.0cm and diameter of wire 2.50 mm, then find maximum permissible percentage error in resistivity.
View SolutionIn the experiment of Ohm's law, a potential difference of 5.0 V is applied across the end of a conductor of length 10.0 cm and diameter of 5.00 mm. The measured current in the conductor is 2.00 A. The maximum permissible percentage error in the resistivity of the conductor is :
View Solution
Knowledge Check
In the determination of Young's modulus (Y=4MLgπ/d2) by using Searle's method, a wire of length L= 2 m and diameter d = 0.5 mm is used. For a load M = 2.5 kg, an extension l = 0.25 mm in the length of the wire is observed. Quantities d and l are measured using a screw gauge and a micrometer, respectively. The have the same pitch of 0.5 mm. The number of divisions on their circular scale is 100. The contributions to the maximum probable error of the Y measurement
Adue to the errors in the measurements of d and l are the sameBdue to the error in the measurement of d is twice that due to the error in the measurement of l.Cdue to the error in the measurement of l is twice that due to the error in the measurement of d.Ddue to the error in the measurement of d is four times that due to the error in the measurement of l.From ideal instruments, current measured is I=10.0 amp, potential difference measured is V=100.0 volt, length of wire is 31.4cm, and diameter of wire is 2.00mm, the resistivity of wire will be (in correct significant figure) (π=3.14)
A1.00×10−4Ω−mB1.0×10−4Ω−mC1×10−4Ω−mD1.000×10−4Ω−mFrom some instruments current measured is I=10.0amp, potential different measured is V=100.0V, length of wire is 31.4cm, and diameter of wire is 2.00mm (all in correct significant figure). The resistivity of wire (in correct significant figures)will be (use π=3.14)
A1.00×10−4Ω−mB1.0×10−4Ω−mC1×10−4Ω−mD1.000×10−4Ω−m
Wire C and wire D are made from different materials and have length LC=LD=1.0m. The resistivity and radius of wire C are 2.0×10−6.Ωm and 1.00 mm, and those of wire D are 1.0×1.0−6Ω.m. and 0.50 mm. The wires are joined as shown in Fig. 26-25, and a current of 2.0 A is set up in them. What is the electric potential difference between a) points 1 and 2 and (b) points 2 and 3? What is the rate at which energy is dissipated between c) points 1 and 2 and (d) points 2 and 3?

View SolutionIn an experiment, current measured is, 1=10.0A, potential difference measured is V=100.0C, length of the wire is 31.4cm and the diameter of the wire 2.00mm (all in correct significant figures). Find resistivity of the wire in correct significant figures. [Take π=3.14, exact].
(a)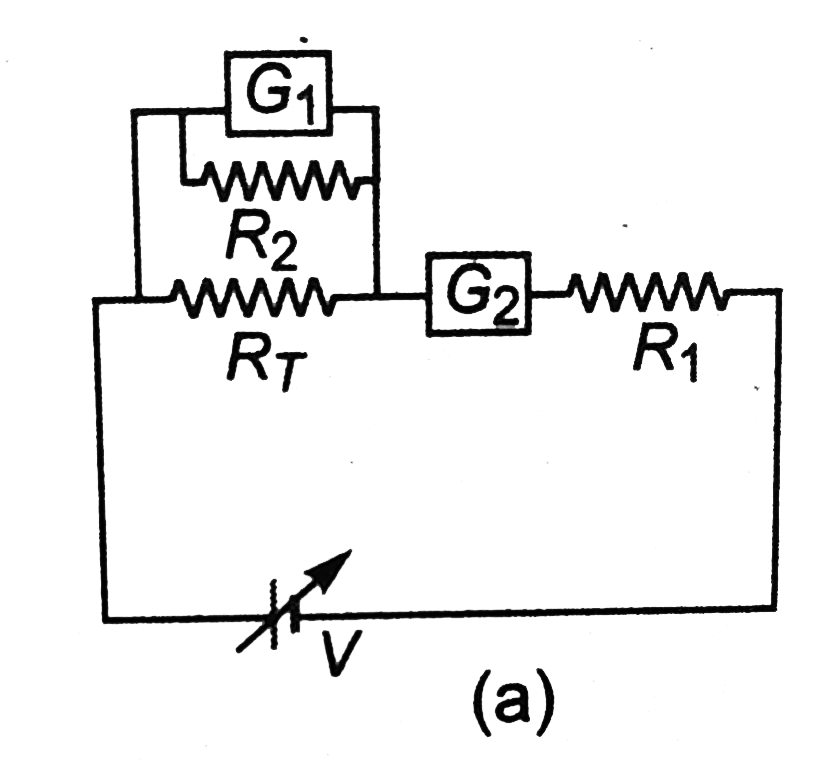
(b)
(c)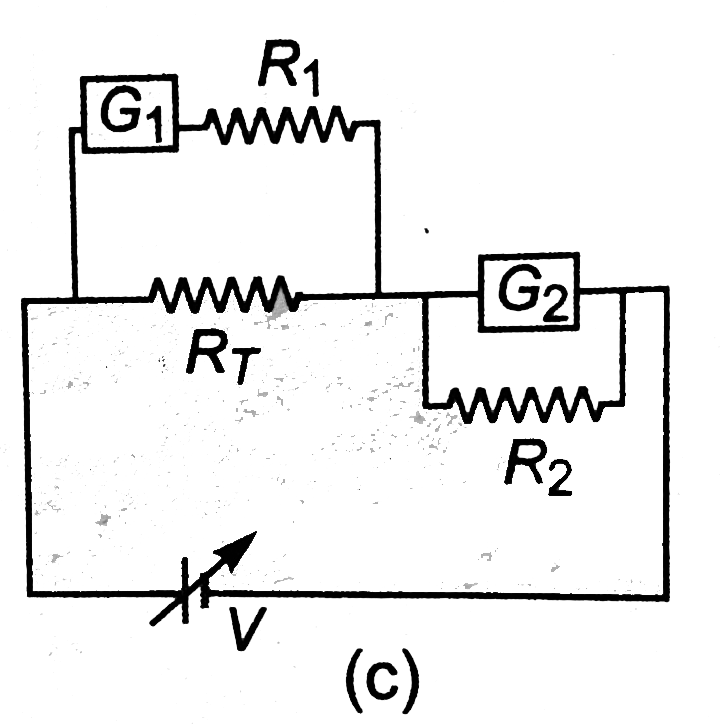
(d)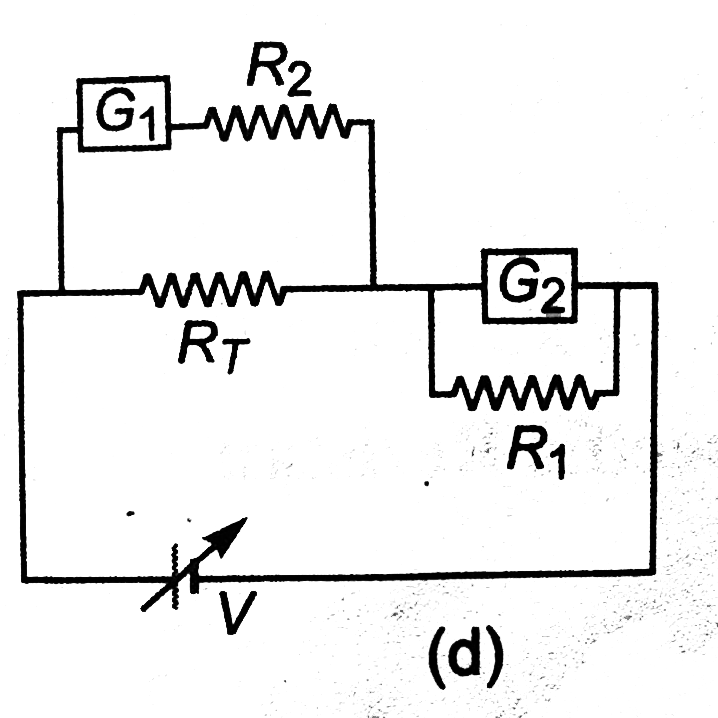 .
.
View SolutionA straight cylindrical wire lying along the X- axis has a length of 0.5m" and a diameter of 0.2mm" .It has a resistivity of "rho=4times10^(-8)Omega-m Let a potential of "4V" us maintained at "x" - "=0" to x=0.5m Resistance of the wire is
View SolutionIn the determination of Young's modulus (Y=4MLgπ/d2) by using Searle's method, a wire of length L= 2 m and diameter d = 0.5 mm is used. For a load M = 2.5 kg, an extension l = 0.25 mm in the length of the wire is observed. Quantities d and l are measured using a screw gauge and a micrometer, respectively. The have the same pitch of 0.5 mm. The number of divisions on their circular scale is 100. The contributions to the maximum probable error of the Y measurement
View SolutionTwo switch in the figurte is open for t<0 and is the thrown closed at time t=0. Assume R=4.00ω,L=1.00H, and ε=10.0V.

The current in the switch as functions of time t.
View Solution