In the experiment of Ohm's law, when potential difference of 10.0 V is applied, current measured is 1.00 A. If length of wire is found to be 10.0cm and diameter of wire 2.50 mm, then find maximum permissible percentage error in resistivity.
The correct Answer is:C
To solve the problem of finding the maximum permissible percentage error in resistivity based on the given parameters, we can follow these steps:
1. Understand Ohm's Law:
Ohm's law states that V=I⋅R, where V is the potential difference, I is the current, and R is the resistance.
2. Express Resistance in Terms of Resistivity:
The resistance R can be expressed in terms of resistivity ρ as:
R=ρ⋅LA
where L is the length of the wire and A is the cross-sectional area. For a wire with diameter D:
A=πD24
Thus, we can rewrite the resistance as:
R=ρ⋅LπD24=4ρLπD2
3. Rearranging for Resistivity:
Rearranging the above equation gives us:
ρ=V⋅πD24⋅I⋅L
4. Identify the Given Values:
- V=10.0V
- I=1.00A
- L=10.0cm=0.1m
- D=2.50mm=0.0025m
5. Determine the Errors in Measurements:
- The error in potential ΔV=0.1V
- The error in current ΔI=0.01A
- The error in length ΔL=0.1cm=0.001m
- The error in diameter ΔD=0.01mm=0.00001m
6. Calculate the Relative Errors:
The relative error in resistivity ρ can be expressed as:
Δρρ=ΔVV+2⋅ΔDD+ΔII+ΔLL
7. Substituting the Values:
- Calculate ΔVV=0.110=0.01
- Calculate ΔDD=0.000010.0025=0.004
- Calculate ΔII=0.011=0.01
- Calculate ΔLL=0.0010.1=0.01
Now substituting these values into the relative error equation:
Δρρ=0.01+2⋅0.004+0.01+0.01
Δρρ=0.01+0.008+0.01+0.01=0.038
8. Convert to Percentage:
To find the maximum permissible percentage error in resistivity:
Percentage Error=Δρρ×100%=0.038×100%=3.8%
Final Answer:
The maximum permissible percentage error in resistivity is 3.8%.
Ohm's law states that V=I⋅R, where V is the potential difference, I is the current, and R is the resistance.
The resistance R can be expressed in terms of resistivity ρ as:
R=ρ⋅LA
where L is the length of the wire and A is the cross-sectional area. For a wire with diameter D:
A=πD24
Thus, we can rewrite the resistance as:
R=ρ⋅LπD24=4ρLπD2
Rearranging the above equation gives us:
ρ=V⋅πD24⋅I⋅L
- V=10.0V
- I=1.00A
- L=10.0cm=0.1m
- D=2.50mm=0.0025m
- The error in potential ΔV=0.1V
- The error in current ΔI=0.01A
- The error in length ΔL=0.1cm=0.001m
- The error in diameter ΔD=0.01mm=0.00001m
The relative error in resistivity ρ can be expressed as:
Δρρ=ΔVV+2⋅ΔDD+ΔII+ΔLL
- Calculate ΔVV=0.110=0.01
- Calculate ΔDD=0.000010.0025=0.004
- Calculate ΔII=0.011=0.01
- Calculate ΔLL=0.0010.1=0.01
Δρρ=0.01+2⋅0.004+0.01+0.01
Δρρ=0.01+0.008+0.01+0.01=0.038
To find the maximum permissible percentage error in resistivity:
Percentage Error=Δρρ×100%=0.038×100%=3.8%
The maximum permissible percentage error in resistivity is 3.8%.
Topper's Solved these Questions
- EXPERIMENTSBook:DC PANDEYChapter:EXPERIMENTSExercise:Exercise 3.1
Explore 2 Videos
- EXPERIMENTSBook:DC PANDEYChapter:EXPERIMENTSExercise:Exercise 3.2
Explore 2 Videos
- ELECTROSTATICSBook:DC PANDEYChapter:ELECTROSTATICSExercise:Integer
Explore 17 Videos
- FLUID MECHANICSBook:DC PANDEYChapter:FLUID MECHANICSExercise:Medical entranes gallery
Explore 49 Videos
Similar Questions
In the experiment of Ohm's law, a potential difference of 5.0 V is applied across the end of a conductor of length 10.0 cm and diameter of 5.00 mm. The measured current in the conductor is 2.00 A. The maximum permissible percentage error in the resistivity of the conductor is :
View SolutionA potential difference of 100 V is applied across a wire of resistance 50 Ω for one minute. Find the heat produced in joule.
View Solution
Knowledge Check
The potential difference across the ends of a wire is found to be (100±2) volt and the current flowing in the wire is found to be (10±0.1)A. What is the maximum percentage error in the measurement of resistance?
A2%B3%C4%D5%The potential difference across the ends of a wire is found to be (100±2) volt and the current flowing in the wire is found to be (10±0.1)A. What is the maximum percentage error in the measurement of resistance?
A2%B3%C4%D5%In Searle's exp to find Young's modulus, the diameter of wire is mesured as D=0.05cm length of wire is L=125cm, and when a weight, m=20.0kg is put, extension in wire was found to be 0.100cm. Find maximum permissible percentage error in Young's modulus (Y)
A2.1%B3.2%C4.3%D5.4%
A potential difference of 100 V is applied across a wire for two minutes. If the current through the wire is 0.1 A , find the heat produced in houle .
View SolutionIn ohm's law experiment potential drop across a resistance was measured as v=5.0 volt and current was measured as I=2.0amp If least count of the volmeter and ammeter are 0.1V and 0.01A respectively then find the maximum permissible error in resistance .
View SolutionIn an experiment, current measured is, 1=10.0A, potential difference measured is V=100.0C, length of the wire is 31.4cm and the diameter of the wire 2.00mm (all in correct significant figures). Find resistivity of the wire in correct significant figures. [Take π=3.14, exact].
(a)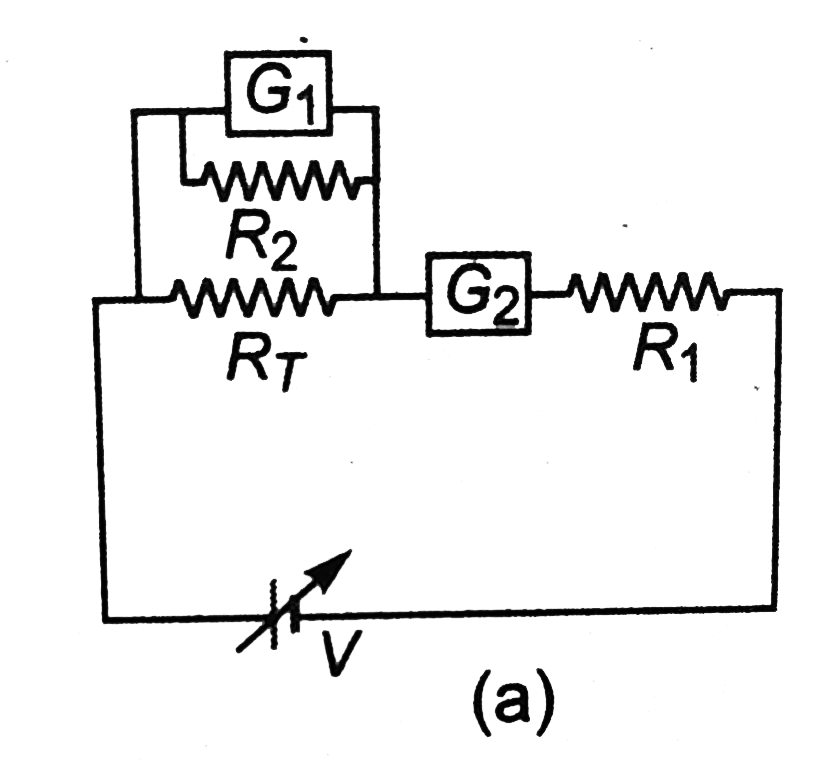
(b)
(c)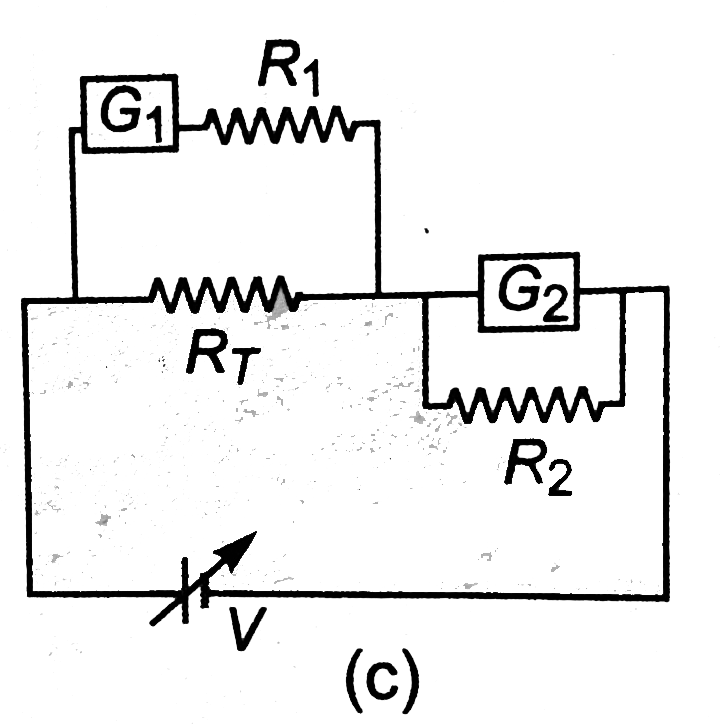
(d)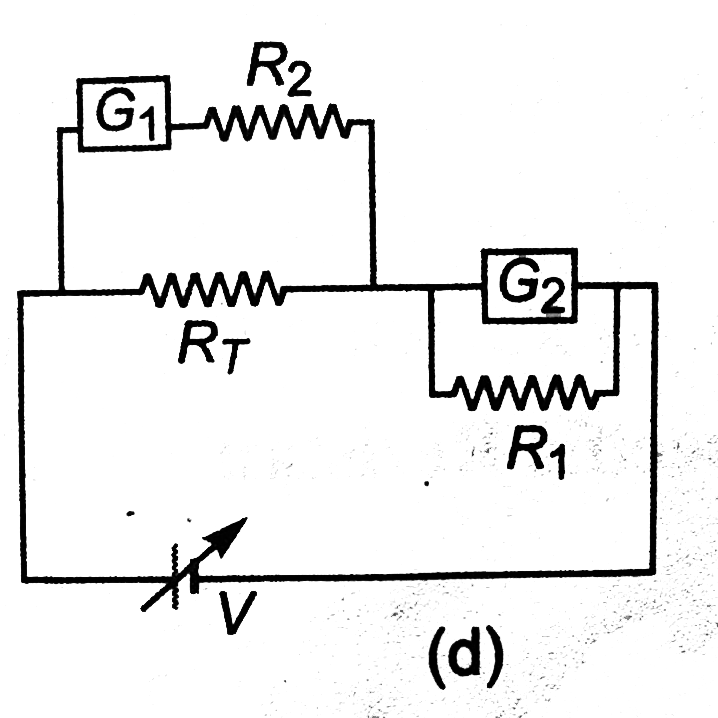 .
.
View SolutionIn u−v method to find focal length of a concave mirror, if object distance is found to be 10.0cm and image distance was also found to be 10.0cm, then find maximum permissible error in (f).
View SolutionIf the measured value of resistance R=1.05Ω, wire diameter d=0.60mm , and length l=75.3cm, then find the maximum permissible error in resistivity ,
ρ=R(πd2/4)l.
View Solution
