A sphere of radius 'a' substends an angle 60∘ at a point P. Then the distance of P from the centre of the sphere isSolution in Telugu
a√3
2a
a√32
2a√3
The correct Answer is:B
Step by step video, text & image solution for A sphere of radius 'a' substends an angle 60^(@) at a point P. Then the distance of P from the centre of the sphere is by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- HEIGHTS AND DISTANCESBOOK - AAKASH SERIESCHAPTER - HEIGHTS AND DISTANCESEXERCISE - PRACTICE SHEET Exercise-I (Level-I) (Straight Objective Type Questions)
39 Videos
- FUNCTIONS BOOK - AAKASH SERIESCHAPTER - FUNCTIONS EXERCISE - PRACTICE EXERCISE
50 Videos
- HYPERBOLIC FUNCTIONS BOOK - AAKASH SERIESCHAPTER - HYPERBOLIC FUNCTIONS EXERCISE - PRACTICE SHEET EXERCISE-II (STRAIGHT OBJECTIVE TYPE QUESTIONS)
18 Videos
Similar Questions
The angle of elevation of an electric pole from a point A on the ground is 60∘ and from a Point B towards the pole on the line joining the foot of the pole to the point A, is 75∘. If the distance AB = a, then the height of the pole is
View SolutionIf f(x)=3x−75x−3 then (fof)(x)=
View SolutionThe domain of cosh−1(2x) is
View SolutionA sphere of radius a subtends an angle 60^(@) at a point P. Then the distance of P from the centre of the sphere is.
View SolutionA uniform sphere has a mass M and radius R. Find the pressure p inside the sphere, caused by gravitational compression, as a function of the distance r from its centre. Evaluate p at the centre of the Earth, assuming it to be a uniform sphere.
View SolutionA cavity of radius r is made inside a solid sphere. The volume charge density of the remaining sphere is p. An electron (charge e, mass m) is released inside the cavity from point P as shown in figure. The centre of sphere and centre of cavity are separated by a distance a. The time after which the electron again touches the sphere is
View SolutionP is a point at a distance r from the centre of a solid sphere of radius a. The gravitational potential at P is V. IF V is plotted as a function of r, which is the correct curve ?
View SolutionA sphere of radius 2R and mas M has a spherical cavity of radius R as shown in the figure. Find the value of gravitational field at a point P at a distance of 6R from centre of the sphere.

View SolutionA uniform solid of valume mass density ρ and radius R is shown in figure.
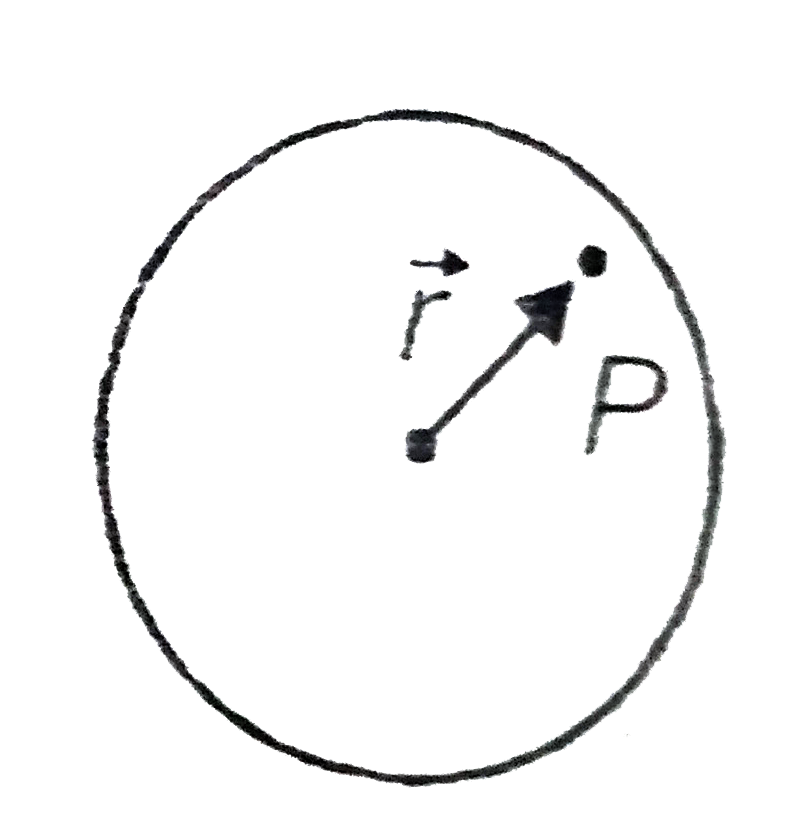
(a) Find the gravitational field at a point P inside the sphere at a distance r from the centre of the sphere. Represent the gravitational field vector →l in terms of radius vector →r of point P.
(b) Now a spherical cavity is made inside the solid sphere in such a way that the point P comes inside the cavity. The centre is at a distance a from the centre of solid sphere and point P is a distance of b from the centre of the cavity. Find the gravitational field →E at point P in vector formulationand interpret the result.
View SolutionIf charge q is induced on outer surface of sphere of radius R, then intensity at a point P at a distance S from its centre is
View SolutionIf charge q induced on outer surface of sphere of radius R, then intensity at point P at distance S from centre is
View SolutionThe centre of neutral conducting sphere of radius "R" is at distance 'd' from a point charge "Q" .The potential of the sphere is
View SolutionP is a point at a distance r from the centre of a solid sphere of radius a. The gravitational potential at P is V. If V is plotted as a function of r, which is the correct curve ?
View SolutionP is a point at a distance r from the centre of a solid sphere of radius a. The gravitational potential at P is V. If V is plotted as a function of r, which is the correct curve ?
View SolutionP is a point at a distance r from the centre of solid sphere of radius a. The gravitational potential at P is V. IF V is plotted as a function of r, which is the correct curve ?
View Solution
