Assertion: In the ideal case of zero damping, the amplitude of simpl harmonic motion at resonance is is infinite.
Reason: All real sysmtems have some damping.
If both assertion and reson are true and reason is the correct explanation of assertion
If both assertion and reason are true but reason is not the correct explanation of assertion.
If assertion is true but reason is false.
If both assertion and reason are false.
The correct Answer is:B
To solve the assertion and reason question, we will analyze both statements step by step.
Step 1: Analyze the Assertion
The assertion states that "In the ideal case of zero damping, the amplitude of simple harmonic motion at resonance is infinite."
- In a system undergoing simple harmonic motion (SHM), resonance occurs when the frequency of an external force matches the natural frequency of the system.
- In the absence of damping (ideal case), the energy supplied to the system can continuously increase the amplitude of oscillation.
- As a result, theoretically, if damping is zero, the amplitude can grow indefinitely, leading to the conclusion that the amplitude at resonance would indeed be infinite.
Conclusion for Assertion: True
Step 2: Analyze the Reason
The reason states that "All real systems have some damping."
- In reality, every physical system experiences some form of damping due to factors like friction, air resistance, or internal material properties.
- Damping acts to dissipate energy from the system, which prevents the amplitude from becoming infinite.
- Therefore, this statement is also true as it reflects the behavior of real-world systems.
Conclusion for Reason: True
Step 3: Determine the Relationship Between Assertion and Reason
Now, we need to determine if the reason provided correctly explains the assertion.
- While both statements are true, the reason does not explain why the assertion is true. The assertion talks about an ideal case (zero damping) leading to infinite amplitude, while the reason discusses the reality of damping in all systems.
- Thus, the reason does not provide a correct explanation for the assertion.
Final Conclusion
The correct option is that both the assertion and reason are true, but the reason is not the correct explanation of the assertion.
Summary of the Solution:
- Assertion: True (in zero damping, amplitude at resonance is infinite)
- Reason: True (all real systems have some damping)
- Relationship: Reason does not explain the assertion.
The assertion states that "In the ideal case of zero damping, the amplitude of simple harmonic motion at resonance is infinite."
- In the absence of damping (ideal case), the energy supplied to the system can continuously increase the amplitude of oscillation.
- As a result, theoretically, if damping is zero, the amplitude can grow indefinitely, leading to the conclusion that the amplitude at resonance would indeed be infinite.
The reason states that "All real systems have some damping."
- Damping acts to dissipate energy from the system, which prevents the amplitude from becoming infinite.
- Therefore, this statement is also true as it reflects the behavior of real-world systems.
Now, we need to determine if the reason provided correctly explains the assertion.
- Thus, the reason does not provide a correct explanation for the assertion.
The correct option is that both the assertion and reason are true, but the reason is not the correct explanation of the assertion.
- Assertion: True (in zero damping, amplitude at resonance is infinite)
- Reason: True (all real systems have some damping)
- Relationship: Reason does not explain the assertion.
Topper's Solved these Questions
- OSCILLATIONS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - OSCILLATIONS EXERCISE - Periodic And Oscillatory Motions
7 Videos
- OSCILLATIONS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - OSCILLATIONS EXERCISE - Simple Harmonic Motion
17 Videos
- OSCILLATIONS BOOK - NCERT FINGERTPS ENGLISHCHAPTER - OSCILLATIONS EXERCISE - Exemplar Problems
9 Videos
- MOTION IN A STRAIGHT LINEBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - NCERT Exemplar
6 Videos
- PHYSICAL WORLDBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - Assertion And Reason
10 Videos
Similar Questions
Assertion : Inside a conductor, electrons have no motion in the absence of some potential difference across it.
Reason : In the absence of potential difference no electrostatic force will act on the electrons inside the conductor.
View SolutionAssertion : At zero degree kelvin, the volume occupied by a gas is negligible.
Reason : All molecular motion ceases at 0K.
View Solution
Knowledge Check
Assertion: If the amplitude of a simple harmonic oscillator is doubled, its total energy becomes double.
Reason: The toal energy is directly proportional to the amplitude of vibration of the harmonic oscillator.AIf both assertion and reson are true and reason is the correct explanation of assertionBIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.Assertion: A combination of two simple harmonic motions with a arbitrary amplitudes and phases is not necessarily periodic.
Reason: A periodic motion can always be expressed as a sum of infinite number of harmonic motions with appropriate amplitudes.AIf both assertion and reson are true and reason is the correct explanation of assertionBIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false.Which of the below figure (s) represent damped simple harmonic motion.
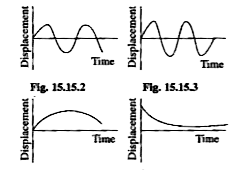
Select the correct answer using the codes given belowAFig. 1 aloneBFig. 2 aloneCFig. 4 aloneDFig. 3 and 4
Assertion : All small oscillation are simple harmonic in nature.
Reason : Oscillation of spring block system are always simple harmonic whether amplitude is small or large.
View SolutionAssertion : We can call circular motion also as simple harmonic motion.
Reason : Angular velocity in uniform circular motion and angular frequency in simple harmonic motion have the same meanings.
View SolutionAssertion : Path of a particle in SHM is always a straight line.
Reason : All straight line motions are not simple harmonic.
View SolutionA pendulum is executing simple harmonic motion and its maximum kinetic energy is K1. If the length of the pendulum is doubled and it perfoms simple harmonuc motion with the same amplitude as in the first case, its maximum kinetic energy is K2 Then:
View SolutionAssertion: The motion of a simple pendulum is simple harmoni for all angular displacement.
Reason: Motion of simple pendulum is independent of the angular displacement.
View Solution
