Two spherical conductors B and C having equal radii and cayying equal charges on them repel each other with a force F when kept apart at some distance. A third spherical conductor having same radius as that B but uncharged is brought in contact with B, then brought in contact with C and finally removed away from both. The new force of repulsion between B and C is
F4
3F4
F8
3F8
The correct Answer is:D
To solve the problem, we will follow these steps:
Step 1: Understand the Initial Setup
- We have two spherical conductors B and C, both carrying an equal charge Q and separated by a distance R.
- The force of repulsion between them is given as F.
Step 2: Apply Coulomb's Law
- According to Coulomb's law, the force F between two charges is given by:
F=14πϵ0Q1Q2R2
- Since both charges are equal, we can write:
F=14πϵ0Q2R2(1)
Step 3: Bring the Uncharged Conductor A in Contact with B
- When the uncharged conductor A (with charge 0) comes into contact with B (with charge Q), the charges will redistribute equally because they are identical in size.
- After contact, the charge on both B and A will be:
QA=QB=Q2
Step 4: Bring Conductor A in Contact with C
- Now, conductor A (with charge Q2) is brought in contact with conductor C (with charge Q).
- The total charge when A and C are in contact is:
Qtotal=Q+Q2=3Q2
- Since they are identical, the charge will redistribute equally:
QC=QA=3Q4
Step 5: Determine the New Charges
- After the process, we have:
- Charge on B: QB=Q2
- Charge on C: QC=3Q4
Step 6: Calculate the New Force of Repulsion
- Now, we need to find the new force of repulsion F′ between B and C:
F′=14πϵ0QBQCR2
- Substituting the values of QB and QC:
F′=14πϵ0(Q2)(3Q4)R2
- Simplifying this gives:
F′=14πϵ03Q28R2
Step 7: Relate the New Force to the Original Force
- From equation (1), we know that:
F=14πϵ0Q2R2
- Thus, we can express F′ in terms of F:
F′=38F
Final Answer
- The new force of repulsion between B and C after the process is:
F′=38F
- We have two spherical conductors B and C, both carrying an equal charge Q and separated by a distance R.
- The force of repulsion between them is given as F.
- According to Coulomb's law, the force F between two charges is given by:
F=14πϵ0Q1Q2R2
- Since both charges are equal, we can write:
F=14πϵ0Q2R2(1)
- When the uncharged conductor A (with charge 0) comes into contact with B (with charge Q), the charges will redistribute equally because they are identical in size.
- After contact, the charge on both B and A will be:
QA=QB=Q2
- Now, conductor A (with charge Q2) is brought in contact with conductor C (with charge Q).
- The total charge when A and C are in contact is:
Qtotal=Q+Q2=3Q2
- Since they are identical, the charge will redistribute equally:
QC=QA=3Q4
- After the process, we have:
- Charge on B: QB=Q2
- Charge on C: QC=3Q4
- Now, we need to find the new force of repulsion F′ between B and C:
F′=14πϵ0QBQCR2
- Substituting the values of QB and QC:
F′=14πϵ0(Q2)(3Q4)R2
- Simplifying this gives:
F′=14πϵ03Q28R2
- From equation (1), we know that:
F=14πϵ0Q2R2
- Thus, we can express F′ in terms of F:
F′=38F
- The new force of repulsion between B and C after the process is:
F′=38F
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBook:NCERT FINGERTPS ENGLISHChapter:ELECTRIC CHARGES AND FIELDSExercise:NCERT Exemplar
Explore 5 Videos
- ELECTRIC CHARGES AND FIELDSBook:NCERT FINGERTPS ENGLISHChapter:ELECTRIC CHARGES AND FIELDSExercise:Application Of Gauss Law
Explore 9 Videos
- DUAL NATURE OF RADIATION AND MATTER Book:NCERT FINGERTPS ENGLISHChapter:DUAL NATURE OF RADIATION AND MATTER Exercise:Assertion And Reason
Explore 15 Videos
- ELECTROMAGNETIC INDUCTIONBook:NCERT FINGERTPS ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:NCERT Exemplar
Explore 6 Videos
Similar Questions
Suppose the spheres A and B in the above question have identical sizes. A third sphere of the same size but uncharged is brought in contact with the first, then brought in contact with the second, and finally removed from both. What is the new force of repulsion between A and B?
View SolutionTwo insulated identically sized charged copper spheres A and B have their centers separated by a distance of 50 cm. A third sphere of the same size but uncharged is brought in contact with the first, then in contact with the second and finally removed from both. What is the new force of repulsion between A and B?
View SolutionTwo equally charged, indentical metal spheres A and B repel each other with a force F. The spheres are kept fixed with a distance r between them. A third identical, but uncharged sphere C is brought in contact with A and removed. The magnitude of the net electric force on A is
View SolutionTwo identical conducting sphere carrying different charges attact each other with a force F when placed in air medium at a distance d apart. The spheres are brought into contact and then taken to their original positions. Now, the two sphere repel each other with a force whole magnitude is equal to the initial attractive force. The ratio between initial charges on the spheres is
View SolutionTwo identical conducting spheres A and B, carry equal charge. They are separated by a distance much larger than their diameters, and the force between them is F. A third identical conducting spheres, C,is uncharged. Sphere C is first touched to A, then to B, and then removed. As a result, the force between A and B would be equal to :
View SolutionTwo identical conducting balls A and B have charges- Q and + 3Q respectively. They are brought in contact with each other and then separated by a distance d part. Find the nature of the Coulomb force between them.
View SolutionThe force of repulsion between two point charges is F, when they are d distance apart. If the point charges are replaced by conducted sphereas each of radius r and the charges remain same. The separation between the centre of sphere isd, then force of repulsion between them is
View SolutionTwo identical conducting spheres 1 and 2 carry equal amounts of charge and are ficed apart at a certain distance larger than their diameters. The spheres repel each other with an electrical force of 88mN. A thired identical sphere 3, having an insulating handle and initially uncharged, is touched first to sphere 1 and then to sphere 2 and finally removed. Find the force between spheres 1 and 2 as shown if Figure.
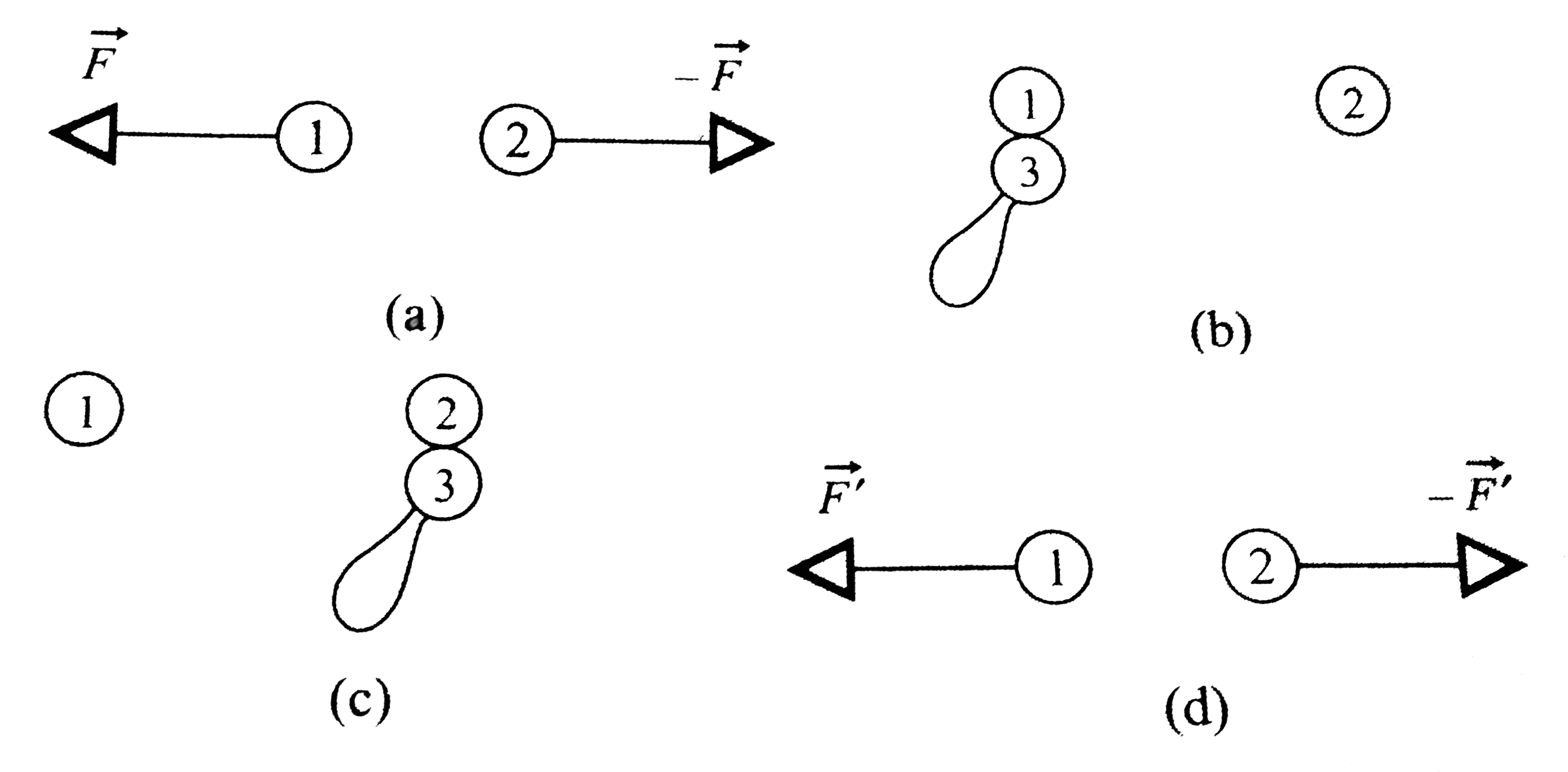 ,
,
View SolutionThe force of repulsion between two point charges is F, when these are at a distance of 1 m apart Now the point charges are replaced by spheres of radii 25 cm having the same charge as that of point charge and same distance apart. Then the new force of repulsion will
View Solution−10μC,40μC and q are the charges on three identical conductors P,Q and R respectively, Now P and Q attract each other with a force F when they are separated by a distance d. Now P and Q are made in contact with each other and then separated . Again Q and R are touched and they are separated by a distance 'd' . The repulsive force between Q and R is 4F . Then the charge q is:
View Solution