A wire of resistance 4Ω is used to wind a coil of radius 7 cm. The wire has a diameter of 1.4 mm and the specific resistance of its material is 2×10−7Ωm. Find the number of turns in the coil.
50
40
60
70
The correct Answer is:D
To find the number of turns in the coil wound with a wire of resistance 4Ω, we will follow these steps:
Step 1: Identify the given values
- Resistance of the wire, R=4Ω
- Radius of the coil, r=7cm=7×10−2m
- Diameter of the wire, d=1.4mm=1.4×10−3m
- Specific resistance (resistivity) of the material, ρ=2×10−7Ω⋅m
Step 2: Calculate the radius of the wire
The radius of the wire Rw can be calculated from the diameter:
Rw=d2=1.4×10−32=0.7×10−3m
Step 3: Calculate the cross-sectional area of the wire
The cross-sectional area A of the wire can be calculated using the formula for the area of a circle:
A=πR2w=π(0.7×10−3)2=π×(0.49×10−6)≈1.539×10−6m2
Step 4: Relate the total length of the wire to the number of turns
The total length L of the wire can be expressed in terms of the number of turns n and the circumference of the coil:
L=n⋅C=n⋅(2πr)=n⋅(2π×7×10−2)
Thus,
L=n⋅(2π×0.07)≈n⋅0.4398m
Step 5: Use the resistance formula
The resistance R of the wire is given by:
R=ρLA
Substituting the values we have:
4=(2×10−7)⋅LA
Substituting L from Step 4:
4=(2×10−7)⋅(n⋅0.4398)1.539×10−6
Step 6: Solve for n
Rearranging the equation:
4⋅1.539×10−6=(2×10−7)⋅(n⋅0.4398)
Calculating the left side:
6.156×10−6=(2×10−7)⋅(n⋅0.4398)
Now, divide both sides by 2×10−7:
n⋅0.4398=6.156×10−62×10−7=30.78
Finally, solve for n:
n=30.780.4398≈69.9≈70
Conclusion
The number of turns in the coil is approximately 70.
---
- Resistance of the wire, R=4Ω
- Radius of the coil, r=7cm=7×10−2m
- Diameter of the wire, d=1.4mm=1.4×10−3m
- Specific resistance (resistivity) of the material, ρ=2×10−7Ω⋅m
The radius of the wire Rw can be calculated from the diameter:
Rw=d2=1.4×10−32=0.7×10−3m
The cross-sectional area A of the wire can be calculated using the formula for the area of a circle:
A=πR2w=π(0.7×10−3)2=π×(0.49×10−6)≈1.539×10−6m2
The total length L of the wire can be expressed in terms of the number of turns n and the circumference of the coil:
L=n⋅C=n⋅(2πr)=n⋅(2π×7×10−2)
Thus,
L=n⋅(2π×0.07)≈n⋅0.4398m
The resistance R of the wire is given by:
R=ρLA
Substituting the values we have:
4=(2×10−7)⋅LA
Substituting L from Step 4:
4=(2×10−7)⋅(n⋅0.4398)1.539×10−6
Rearranging the equation:
4⋅1.539×10−6=(2×10−7)⋅(n⋅0.4398)
Calculating the left side:
6.156×10−6=(2×10−7)⋅(n⋅0.4398)
Now, divide both sides by 2×10−7:
n⋅0.4398=6.156×10−62×10−7=30.78
Finally, solve for n:
n=30.780.4398≈69.9≈70
The number of turns in the coil is approximately 70.
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:NCERT FINGERTPS ENGLISHChapter:CURRENT ELECTRICITYExercise:DRIFT OF ELECTRONS
Explore 2 Videos
- CURRENT ELECTRICITYBook:NCERT FINGERTPS ENGLISHChapter:CURRENT ELECTRICITYExercise:LIMITATIONS OF OHM S LAW
Explore 4 Videos
- CURRENT ELECTRICITYBook:NCERT FINGERTPS ENGLISHChapter:CURRENT ELECTRICITYExercise:ELECTRIC CURRENT IN CONDUCTORS
Explore 2 Videos
- COMMUNITCATION SYSTEMSBook:NCERT FINGERTPS ENGLISHChapter:COMMUNITCATION SYSTEMSExercise:Assertion And Reason
Explore 30 Videos
- DUAL NATURE OF RADIATION AND MATTER Book:NCERT FINGERTPS ENGLISHChapter:DUAL NATURE OF RADIATION AND MATTER Exercise:Assertion And Reason
Explore 15 Videos
Similar Questions
Knowledge Check
A wire of resistance 12Ωm−1 is bent to from a complete circle of radius 10cm. The resistance between its two diametrically opposite points, A and B as shown in the figure, is
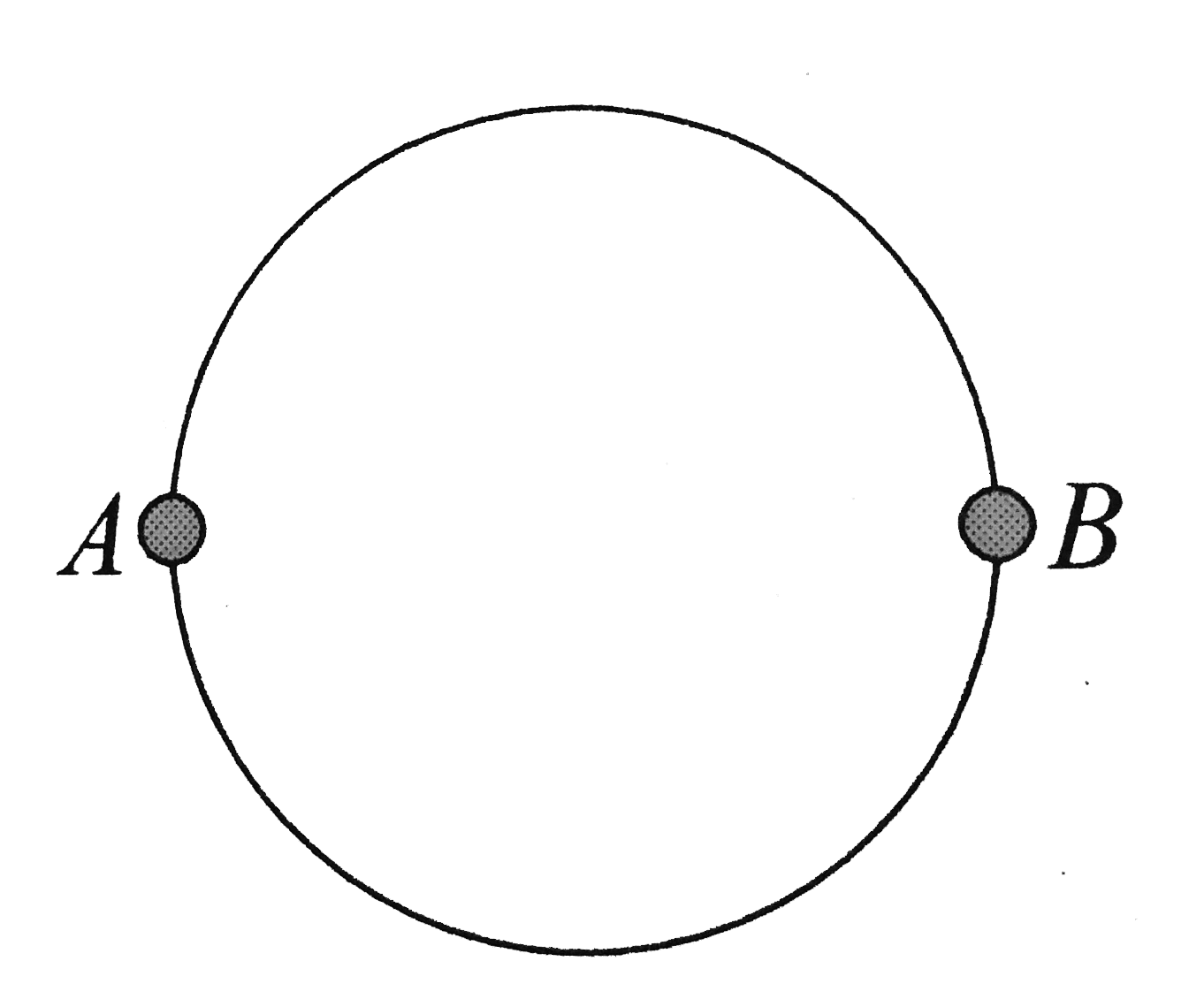 A3ΩB6πΩC6ΩD0.6πΩ
A3ΩB6πΩC6ΩD0.6πΩ
A wire of resistance 5Ω is uniformly stretched until its new length becomes 4 times the original length. Find its new resistance.
View SolutionWhen the resistance of copper wire is 0.1Ω and the radius is 1mm, then the length of the wire is (specific resistance of copper is 3.14×10−8ohm×m)
View SolutionDimensions of a block are 1cm×1cm×100cm. If specific resistance of its material is 3×10−7ohm−m, then the resistance between the opposite rectangular facesis
View SolutionDimensions of a block are 1cm×1cm×100cm. If specific resistance of its material is 3×10−7ohm−m, then the resistance between the opposite square faces is
View SolutionA wire of resistance 12Ωm−1 is bent to from a complete circle of radius 10cm. The resistance between its two diametrically opposite points, A and B as shown in the figure, is

View SolutionIn house wiring, copper wire 2.05mm in diameter is often used. Find the resistane of 35.0m long wire. Specific resistance of copper is 1.72×10−8Ω−m.
View SolutionA metallic wire has a resistance of 3.0Ω at 0∘C and 4.8Ω at 150∘C. Find the temperature coefficient of resistance of its material.
View Solution