In the question number 66, the tension in the string is
30N
36N
34N
32N
The correct Answer is:C
To solve the problem of finding the tension in the string, we can follow these steps:
Step 1: Understand the System
We have a block and a trolley system where the block is hanging and connected to the trolley via a string. We need to find the tension in the string.
Step 2: Identify Given Values
From the transcript, we have:
- The acceleration a=1.5m/s2
- The gravitational acceleration g=10m/s2
- The mass of the block m=4kg (since 4g is mentioned)
Step 3: Write the Equation of Motion
For the block hanging, we can write the equation of motion as:
4g−T=4a
Where:
- T is the tension in the string.
- 4g is the weight of the block.
Step 4: Substitute Known Values
Substituting the known values into the equation:
4(10)−T=4(1.5)
This simplifies to:
40−T=6
Step 5: Solve for Tension
Now, we can solve for T:
40−6=T
T=34N
Conclusion
The tension in the string is 34N.
We have a block and a trolley system where the block is hanging and connected to the trolley via a string. We need to find the tension in the string.
From the transcript, we have:
- The acceleration a=1.5m/s2
- The gravitational acceleration g=10m/s2
- The mass of the block m=4kg (since 4g is mentioned)
For the block hanging, we can write the equation of motion as:
4g−T=4a
Where:
- T is the tension in the string.
- 4g is the weight of the block.
Substituting the known values into the equation:
4(10)−T=4(1.5)
This simplifies to:
40−T=6
Now, we can solve for T:
40−6=T
T=34N
The tension in the string is 34N.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - HOTS
8 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Exemplar Problems
9 Videos
- KINETIC THEORYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason
10 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
Similar Questions
Knowledge Check
In the question number 66, the flux through the same square if the line normal to its plane makes a 60∘ angle with the x-axis is
A30NC−1m2B10NC−1m2C20NC−1m2D25NC−1m2In the question number 66, the charge on capacitors C1andC4 are
A4×10−3C,12×10−3CB6×10−3C,12×10−3CC2×10−3C,4×10−3CD3×10−3C,2×10−3C
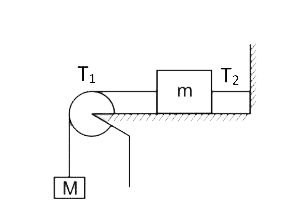
In the following figure, if the tension in the string AB is 51N, then the tension in the string BC will be :-
View SolutionFor the system shown in figure, the tensions in the string
View Solutionn number of waves are produced on a string in 0.5 s. Now, the tension in the string is doubled (Assume length and radius constant), the number of waves produced in 0.5s for the same harmonic will be
View SolutionThe tension in a string, obeying Hooke's law, is x. The speed of sound in the stretched string is v. If the tension in the string is increased to 1.5x, the speed of sound will be
View SolutionA string of mass per unit length μ is clamped at both ends such that one end of the string is at x=0 and the other is at x=L. When string vibrates in fundamental mode, amplitude of the midpoint of string is a and tension in the string is F. Find the total oscillation energy (in J) stored in the string. (Use L=1 m, F = 10N, a = 1/pi m).

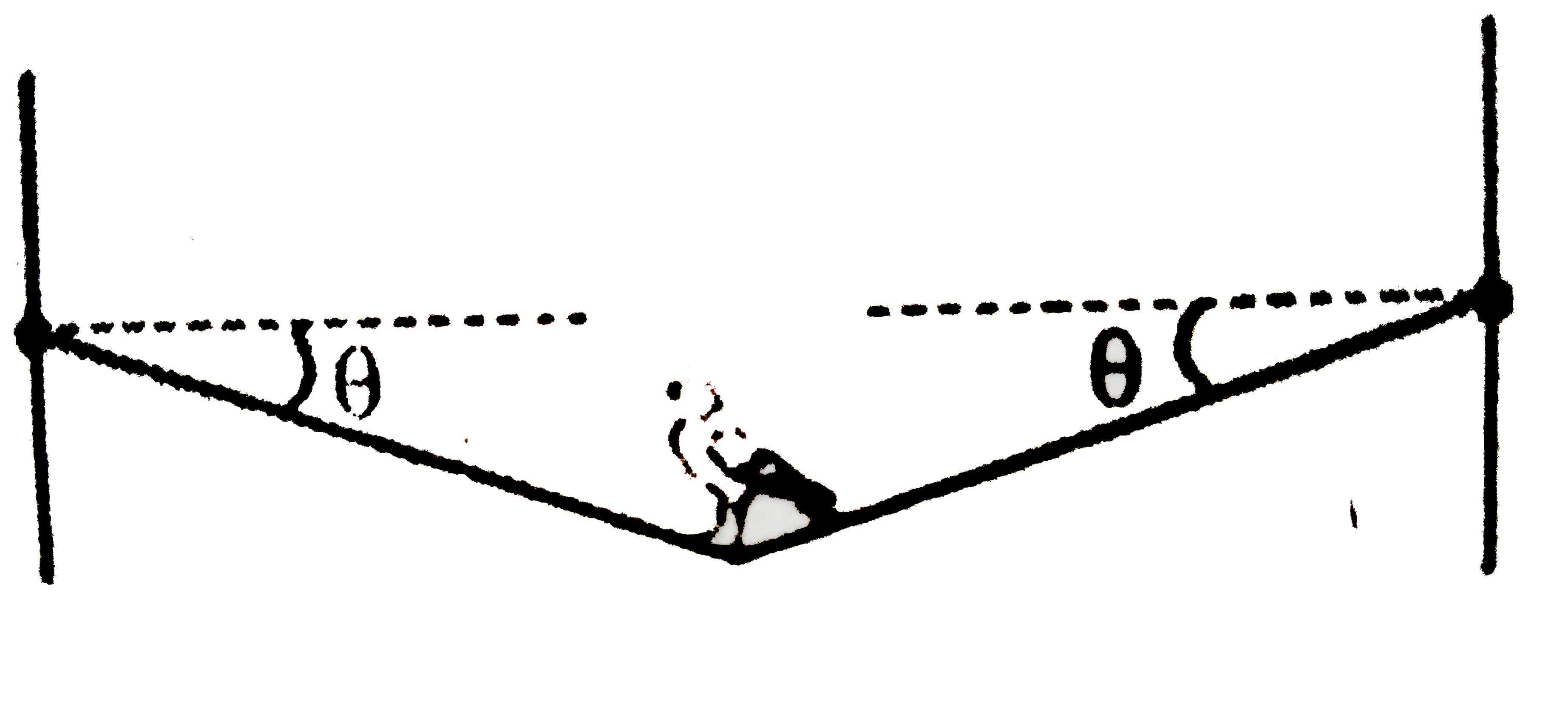
View SolutionA bird with mass m parches at the middle of a strectched string Show that the tension in the string is give by T=mg2sinθ. Assuming that each half of the string is straight.
View Solution
