Assertion : Static friction is a self-adjusting force upto its limit μsNwhereμs is the coefficient of static friction.
Reason: One can use the equation fs=μsN only when the maximum value of static friction comes into play
If both assertion and reason are true and reason is the correct explanation of assertion.
If both assertion and reason are true and reason is the not correct explanation of assertion.
If assertion is true but reason is false
If both assertion and reason are false.
The correct Answer is:B
To solve the question, we need to analyze both the assertion and the reason provided.
Step 1: Understand the Assertion
The assertion states that "Static friction is a self-adjusting force up to its limit μsN, where μs is the coefficient of static friction."
Explanation:
- Static friction acts to oppose the relative motion between two surfaces in contact.
- It can adjust its magnitude based on the applied external force, up to a maximum limit.
- The maximum static friction force is given by the formula:
\(
f_s^{max} = \mu_s N
\)
where \(N\) is the normal force.
Step 2: Understand the Reason
The reason states that "One can use the equation fs=μsN only when the maximum value of static friction comes into play."
Explanation:
- The equation \(f_s = \mu_s N\) applies only when the static friction is at its maximum limit.
- If the applied force is less than this maximum, the static friction force will equal the applied force, not necessarily \(μ_s N\).
Step 3: Analyze the Relationship Between Assertion and Reason
- Both the assertion and the reason are true.
- The assertion correctly describes the nature of static friction as a self-adjusting force.
- The reason accurately states the condition under which the equation for static friction can be applied.
Step 4: Determine the Logical Connection
- While both statements are true, the reason does not directly explain why static friction is self-adjusting. It merely states a condition for using the equation.
Conclusion
- The assertion is true, and the reason is also true, but the reason does not explain the assertion. Therefore, the final answer is:
- Option B: Both assertion and reason are true, but the reason is not the correct explanation for the assertion.
The assertion states that "Static friction is a self-adjusting force up to its limit μsN, where μs is the coefficient of static friction."
- Static friction acts to oppose the relative motion between two surfaces in contact.
- It can adjust its magnitude based on the applied external force, up to a maximum limit.
- The maximum static friction force is given by the formula:
\(
f_s^{max} = \mu_s N
\)
where \(N\) is the normal force.
The reason states that "One can use the equation fs=μsN only when the maximum value of static friction comes into play."
- The equation \(f_s = \mu_s N\) applies only when the static friction is at its maximum limit.
- If the applied force is less than this maximum, the static friction force will equal the applied force, not necessarily \(μ_s N\).
- Both the assertion and the reason are true.
- The assertion correctly describes the nature of static friction as a self-adjusting force.
- The reason accurately states the condition under which the equation for static friction can be applied.
- While both statements are true, the reason does not directly explain why static friction is self-adjusting. It merely states a condition for using the equation.
- The assertion is true, and the reason is also true, but the reason does not explain the assertion. Therefore, the final answer is:
- Option B: Both assertion and reason are true, but the reason is not the correct explanation for the assertion.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - The Law Of Inertia
5 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Newton'S First Law Of Motion
2 Videos
- LAWS OF MOTIONBOOK - NCERT FINGERTPS ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Exemplar Problems
9 Videos
- KINETIC THEORYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - KINETIC THEORYEXERCISE - Assertion And Reason
10 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - NCERT Exemplar
5 Videos
Similar Questions
Assertion: Static frictional force is a self adjusting force.
Reason: Force of static friction does not depend upon the mass of the body.
View SolutionIf μs,μk and μr are the coefficients of static, kinetic and rolling friction respectively then
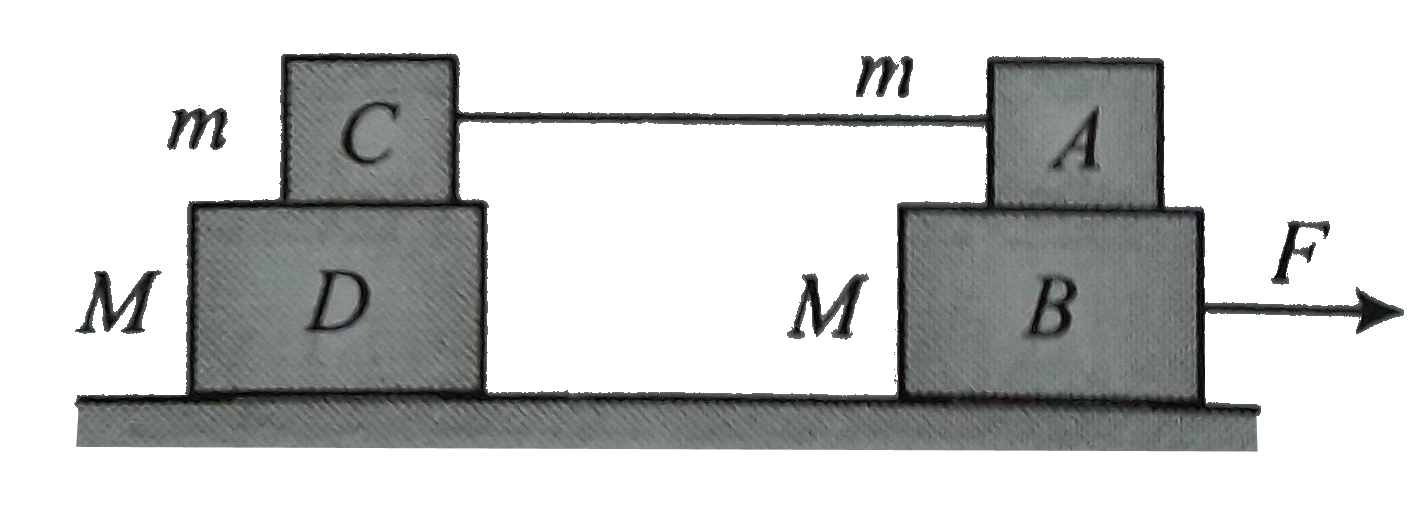
View SolutionFour block are arranged on a smooth horizontal surface as shown in figure .The masses of the blocks are given (see the fig ) The coefficient of static friction between the top and the bottom blocks is μs What is the maximum value of the horizontal force F applied to one of the bottom blocks as shown that makes all four block with the same acceleration ?
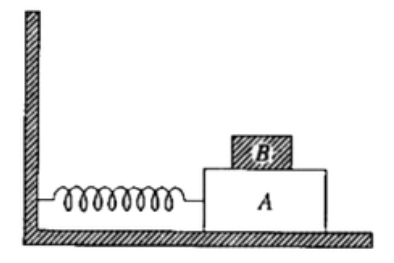
View SolutionIn Fig. A is a large block which executes shm by sliding across a frictionless surface with a frequency n. The block B is at rest on the surface of the block μs The coefficient of static friction between the two is H. Find the maximum amplitude of oscillation of the system so that the block B does not slip.
View SolutionA solid cylinder is rolling without slipping on a plane having inclination θ and the coefficient of static friction μs. The relation between θ and μs is
View SolutionA car has to move on a level turn of radius 45 m. If the coefficient of static friction between the tyre and the road is μs=2.0, find the maximum speed the car can take without skidding.
View SolutionA small coin of mass 40 g is placed on the horizontal surface of a rotating disc. The disc starts from rest and is given a constant angular acceleration α=2 rad s−2. The coefficient of static friction between the coin and the disc is μs=3/4 and the coefficient of kinetic friction is μk=0.5. The coin is placed at a distance r=1m from the centre of the disc. The magnitude of the resultant force on the coin exerted by the disc just before it starts slipping on the disc is?

View SolutionA horizontal force F acts on the sphere at its centre as shown. Coefficient of friction between ground and sphere is μ. What is maximum value of F for which there is no slipping?
View SolutionA horizontal force F acts on the sphere at its centre as shown. Coefficient of friction between ground and sphere is μ. What is maximum value of F for which there is no slipping?
View SolutionAn automobile is moving on a straight horizontal road with a speed u. If the coefficient of static friction between the tyres and the road is μs what is the shortest distance in which the automobiles can be stopped ?
View Solution
