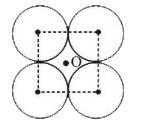
Four solid spheres, each of mass (m) and diameter (d) are stuck together such that the lines joining the centres form a square of side length (d). The ratio of I0 & IA , where I0 is the moment of inertia of the system about an axis through the centre of the square and IA is moment of inertia of the system about the centre of any one of the spheres, is equal to (both axes are perpendicular to plane of paper)
611
411
511
711
The correct Answer is:A
IA=25m(d2)2+2×[25m(d2)2+md2]+[25m(d2)2+md2]+[25m(d2)2+m×(√2d)2]=225m2
I0=4×⎡⎣25(d2)2+m(d√2)2⎤⎦=125md2 I0IA=125225=611
Similar Questions
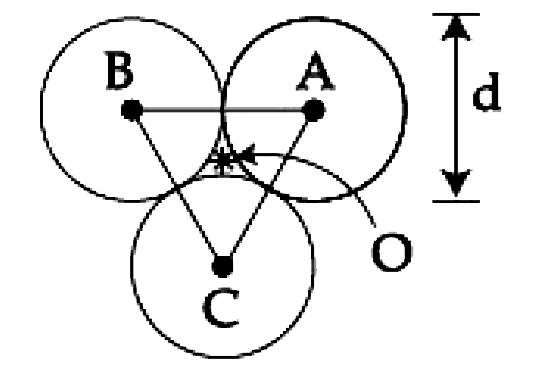
Three solids sphere each of mass m and diameter d are stuck together such that the lines connecting the centers from an equilateral triangle of side of length d. The ratio I0IA of moment of inertia I0 of the system about an axis passing the centroid and about center of any of the spheres IA and perpendicular to the plane of the triangle is
View SolutionThe moment of inertia of a thin uniform rod about an axis passing through its centre and perpendicular to its length is I0. What is the moment of inertia of the rod about an axis passing through one end and perpendicular to the rod ?
View Solution
Knowledge Check
Moment of inertia of a rigid body about an axis passing through its centre of mass is I0 and moment of inertia of the same body about another axis parallel to the first axis is I. Then
AI is always equal to I0BI is always smaller than I0CI is always greater than I0Dany of the above threeFour identical thin rods each of mass M and length l, from a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is
A13Ml2B43Ml2C23Ml2D133Ml2Four spheres of diameter 2a and mass M are placed with their centres on the four corners of a square of side b. Then the moment of inertia of the system about an axis along one of the sides of the square is
A45Ma2+2Mb2B85Ma2+2Mb2C85Ma2+Mb2D45Ma2+4Mb2
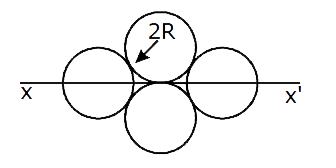
The moment of inertia of a sphere about its diameter is I. Four such spheres are arranged as shown in figure. Find the moment of inertia of the system about the axis XX'. (radius of each sphere is 2R).
View SolutionWhat is the moment of inertia of a rod of mass M, length 1 about an axis perpendicular to it through one end ? Given the moment of inertia about the centre of mass is 112Ml2.
View SolutionThere are four solid balls with their centres at the four comers of a square of side a. the mass of each sphere is m and radius is r. Find the moment of inertia of the system about (i) one of the sides of the square (ii) one of the diagonals of the square.
View SolutionThe moment of inertia of ring about an axis passing through its diameter is I. Then moment of inertia of that ring about an axis passing through its centre and perpendicular to its plane is
View SolutionFour identical thin rods each of mass M and length l3, form a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is---
View Solution