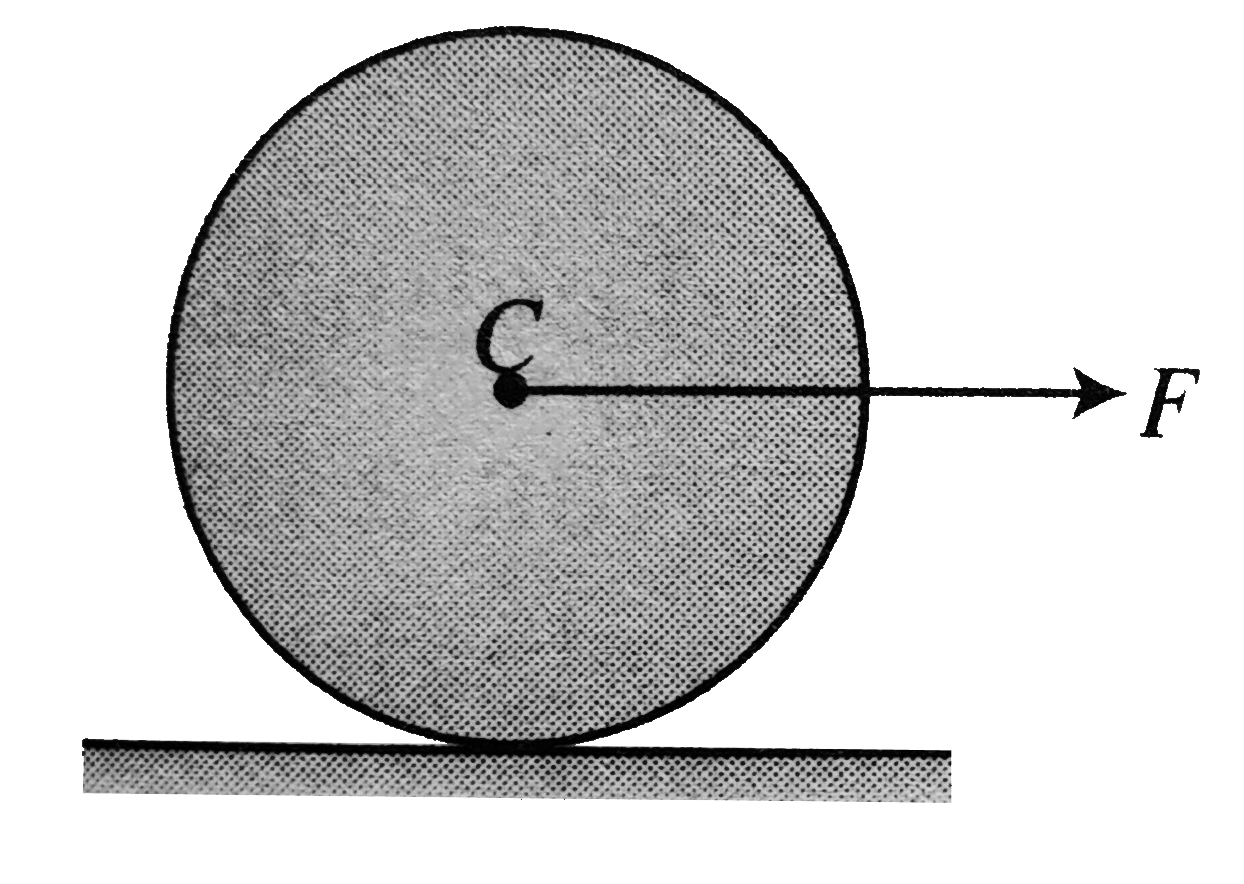
A force F acts tangentially at the highest point of a sphere of mass m kept on a rough horiozontal plane. If the sphere rolls withut slipping, find the accelerastioni of the centre of the sphere.
To solve the problem of finding the acceleration of the center of a sphere of mass m rolling without slipping on a rough horizontal plane when a force F acts tangentially at the highest point, we can follow these steps:
Step 1: Identify the forces acting on the sphere
- The force F acts tangentially at the highest point of the sphere.
- There is a frictional force f acting at the bottom point of the sphere, opposite to the direction of motion.
Step 2: Write the equations of motion
1. Translational motion: The net force acting on the sphere in the horizontal direction gives us:
F−f=ma(1)
where a is the linear acceleration of the center of mass of the sphere.
2. Rotational motion: The torque τ about the center of mass due to the forces is given by:
τ=F⋅r−f⋅r=Iα(2)
where I is the moment of inertia of the sphere and α is the angular acceleration. For a solid sphere, I=25mr2.
Step 3: Relate linear and angular acceleration
Since the sphere rolls without slipping, the relationship between linear acceleration a and angular acceleration α is given by:
a=αr(3)
Step 4: Substitute α in terms of a
From equation (3), we can express α as:
α=ar
Step 5: Substitute α into the torque equation
Substituting α into equation (2):
F⋅r−f⋅r=(25mr2)(ar)
This simplifies to:
F⋅r−f⋅r=25mar
Dividing through by r:
F−f=25ma(4)
Step 6: Solve the equations simultaneously
Now we have two equations (1) and (4):
1. F−f=ma
2. F−f=25ma
Setting these equal to each other:
ma=25ma
This implies:
F−f=ma(from (1))
Step 7: Combine the equations
Adding equations (1) and (4):
F−f+F−f=ma+25ma
This gives:
2F−2f=75ma
Thus:
2F=75ma+2f
Step 8: Solve for acceleration a
Rearranging gives:
a=107F−fm
Assuming f is small or negligible, we can approximate:
a=107Fm
Final Answer
The acceleration of the center of the sphere is:
a=107Fm
- The force F acts tangentially at the highest point of the sphere.
- There is a frictional force f acting at the bottom point of the sphere, opposite to the direction of motion.
1. Translational motion: The net force acting on the sphere in the horizontal direction gives us:
F−f=ma(1)
where a is the linear acceleration of the center of mass of the sphere.
τ=F⋅r−f⋅r=Iα(2)
where I is the moment of inertia of the sphere and α is the angular acceleration. For a solid sphere, I=25mr2.
Since the sphere rolls without slipping, the relationship between linear acceleration a and angular acceleration α is given by:
a=αr(3)
From equation (3), we can express α as:
α=ar
Substituting α into equation (2):
F⋅r−f⋅r=(25mr2)(ar)
This simplifies to:
F⋅r−f⋅r=25mar
Dividing through by r:
F−f=25ma(4)
Now we have two equations (1) and (4):
1. F−f=ma
2. F−f=25ma
ma=25ma
This implies:
F−f=ma(from (1))
Adding equations (1) and (4):
F−f+F−f=ma+25ma
This gives:
2F−2f=75ma
Thus:
2F=75ma+2f
Rearranging gives:
a=107F−fm
Assuming f is small or negligible, we can approximate:
a=107Fm
The acceleration of the center of the sphere is:
a=107Fm
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -1
26 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective -2
14 Videos
- ROTATIONAL MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - ROTATIONAL MECHANICSEXERCISE - Questions for short Answer
21 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMA ENGLISHCHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Question for short Answer
13 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMA ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Question for short Answer
15 Videos
Similar Questions
A force F acts tangentially at the highest point of a sphere f mass m kept on a rough horizontal plane. If the sphere rolls without slipping, find the acceleration of the centre of the sphere.

View SolutionA force F acts tangentially at the highest point of a disc of mass m kept on a rough horizontal plane. If the disc rolls without slipping, the acceleration of centre of the disc is:

View Solution
Knowledge Check

A tangential force F acts at the top of a thin spherical shell of mass m and radius R. Find the acceleration of the shell if it rolls without slipping.Aa. Acceleration of disc = 2F/3mBb. Friction force between disc and surface = 2F/3Cc. Acceleration of disc = 6F/5mDd. Friction force between disc and surface is F/3A uniform ring of mass m and radius a is placed directly above a uniform sphere of mass M and of equal radius. The centre of the ring is at distance √3a from the centre of the sphere. Find the gravitational force exerted by the sphere on the ring.
AGMm8r2BGMm4r2C√3GMm8r2DGMm8r3√3
A horizontal force F=14N acts at the centre of mass of a sphere of mass m=1kg. If the sphere rolls without sliding, find the frictional force (in N)
View Solution
A tangential force F acts at the top of a thin spherical shell of mass m and radius R. Find the acceleration of the shell if it rolls without slipping.
View SolutionTwo uniform solid spheres having unequal radii are released from rest from the same height on a rough incline. If the spheres roll without slipping
View SolutionTwo uniform solid spheres having unequal radii are released from rest from the same height on a rough incline. the spheres roll without slipping
View SolutionA solid sphere of mass m rolls without slipping on an inclined plane of inclination θ. The linear acceleration of the sphere is
View SolutionA solid sphere of mass M and radius R is pulled by a force F as shown in figure . If the sphere does not slip over the surface , then frictional force acting on the sphere is
View Solution
