Question

A wheel of radius r and moment of inertia I about its axis is fixed at top of an inclined plane of inclination $\theta$ as shown in figure. A string is wrapped round the wheel and its free end supports a block of mass M which can slide on the plane. Initially, the wheel is rotating at a speed $\omega$ in direction such that the block slides up the plane. How far will the block move before stopping?

Explanation

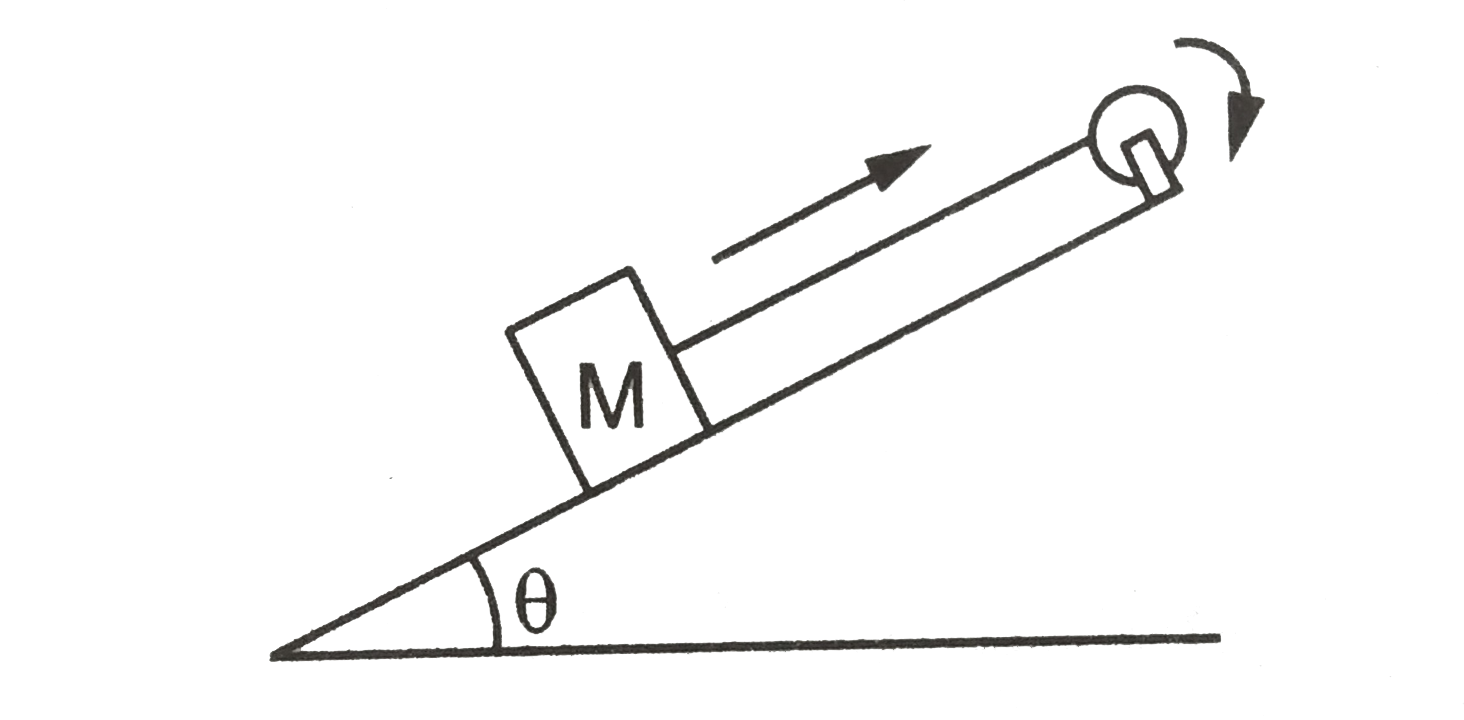
Suppose the deceleration of the block is a. The linear decelerationof the rim of the wheel is also a. The angular decelratinof the wheel is `alpha=a/r`. I the tension in the string is T, the equations of motion are as follows:
`Mgsintheta-T=Ma`
and `Tr=Ialpha=Ia/r`
ElimiN/Ating T from these equations
`Mgsintheta-Ia/r^2=Ma`
giving `a=(Mgr^2sintheta)/(I+Mr^2)`
THe initial velocity of the block up the incline is v=omegar`. Thus the distance moved by the block before stopping is
`x=v^2/(2a) =(omega^2r^2(I+Mr^2))/(2Mgr^2sintheta)=((I+Mr^2)omega^2)/(2Mgsintheta)`