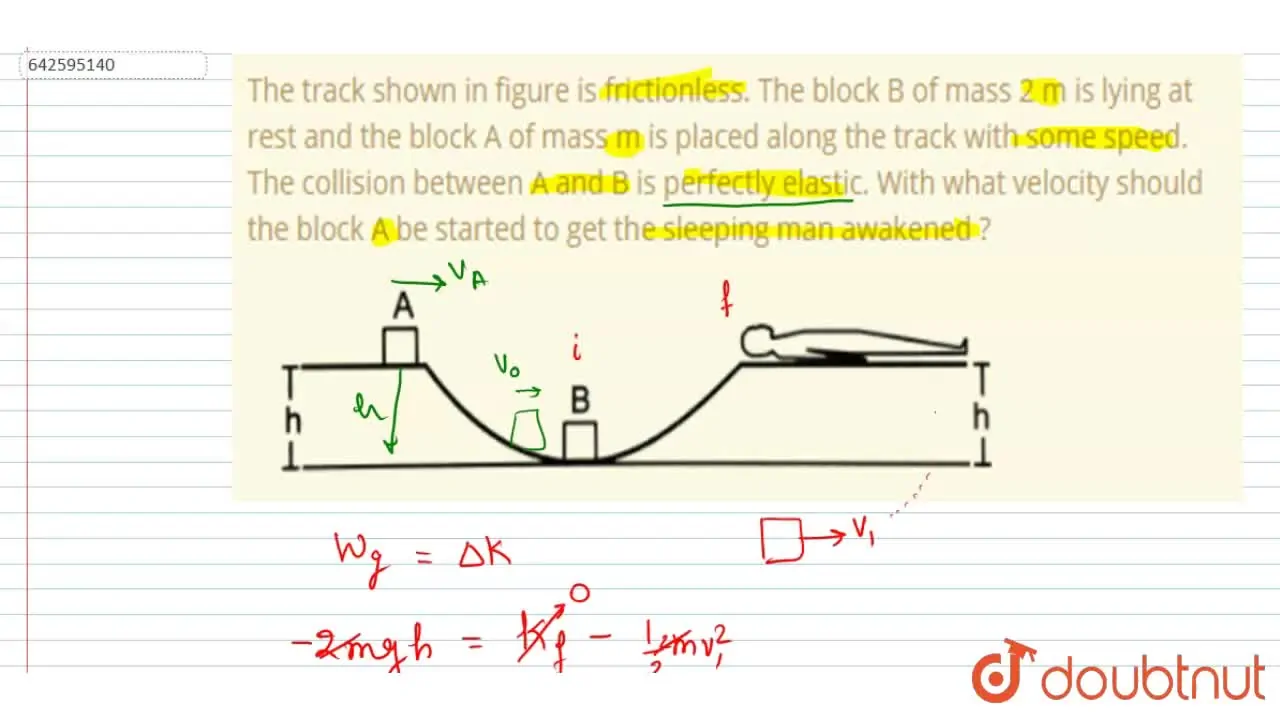
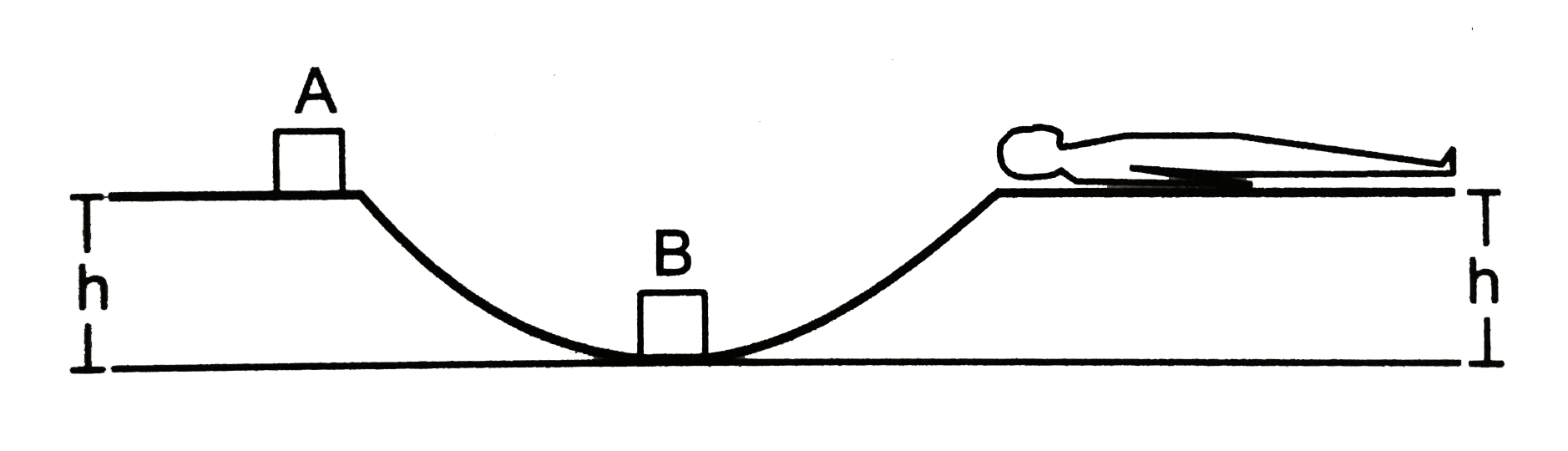
The track shown in figure is frictionless. The block B of mass 2 m is lying at rest and the block A of mass m is placed along the track with some speed. The collision between A and B is perfectly elastic. With what velocity should the block A be started to get the sleeping man awakened ?
The correct Answer is:A, B
Let the velocity of
Let the fiN/Al velocity when reachign at B become collision
……..i
When the block B reached at the upper man's hed, the velocity of B is just zero.
rarr
ltbr>
After collision velocity fo v_A=V(say)v_B=sqrt(2gh):.mxxv_1+2mxx0=mxxv+2m+sqrt(2gh)rarr v_1-v=2sqrt(2gh)(1/2)xxmxxv_1^2+(1/2)xx2m(sqrt(2gh)^2)rarr v_1^2-v^2=2xxsqrt(2gh)xx2msqrt(2gh)((v_1+v)(v_1-v))/(v_1+v)=(2xxsqrt(2gh)xsqrt(2gh))/(2xxsqrt(2gh))rarr v_1+v=sqrt(2gh)2v_1=3sqrt(2gh)rarr v_1=(3/2)sqrt(2gh)But v_1=sqrt(2gh+u^2)=(3/2)sqrt(2gh)
So the block will travel with a velocity greater than i.e., man must awake.
Related Playlists
- BOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Question For short Answer25videos
CENTRE OF MASS, LINEAR MOMENTUM, COLLISION
- BOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -211videos
CENTRE OF MASS, LINEAR MOMENTUM, COLLISION
- BOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Objective -118videos
CENTRE OF MASS, LINEAR MOMENTUM, COLLISION
- BOOK - HC VERMA ENGLISHCHAPTER - CALORIMETRYEXERCISE - Short answer9videos
CALORIMETRY
- BOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer10videos
CIRCULAR MOTION
Similar Questions
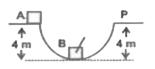
The track shown in the figure is frictionless. The block B of mass 4kg is lying at rest and block A of mass 2kg is pushed along the track with some speed. The collision between A and B is perfectly elastic. With that velocity should the block A start such that block B just reaches at point P ?
 View Solution
View SolutionFor shown situation, if collision between block A and B is perfectly elastic, then find the maximum energy stored in spring in joules.
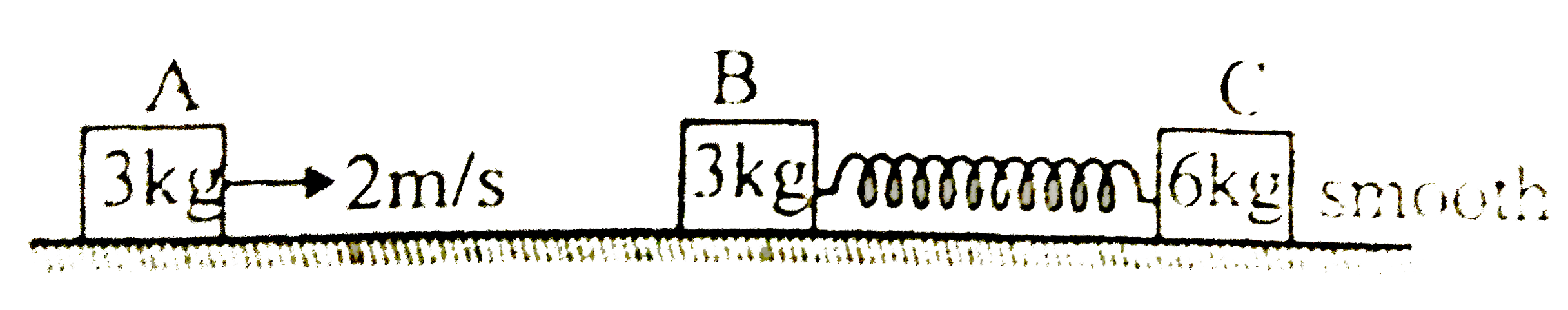 View Solution
View SolutionA block of mass m is placed on the top of another block of mass M as shown in the figure. The coefficient of friction between them is μ.

The maximum acceleration with which the block M may move so that m also moves along with it isView SolutionA block of mass 'm' is released on the top of a frictionless incline as shown in the figure. The time period of the oscillation of the block is
 View Solution
View SolutionA block of mass m moving at speed υ collides with another block of mass 2 m at rest. The lighter block comes to rest after the collision. Find the coefficient of restitution.
View SolutionDescribe the motion of the mass m shown in figure. The walls and the block are elastic.
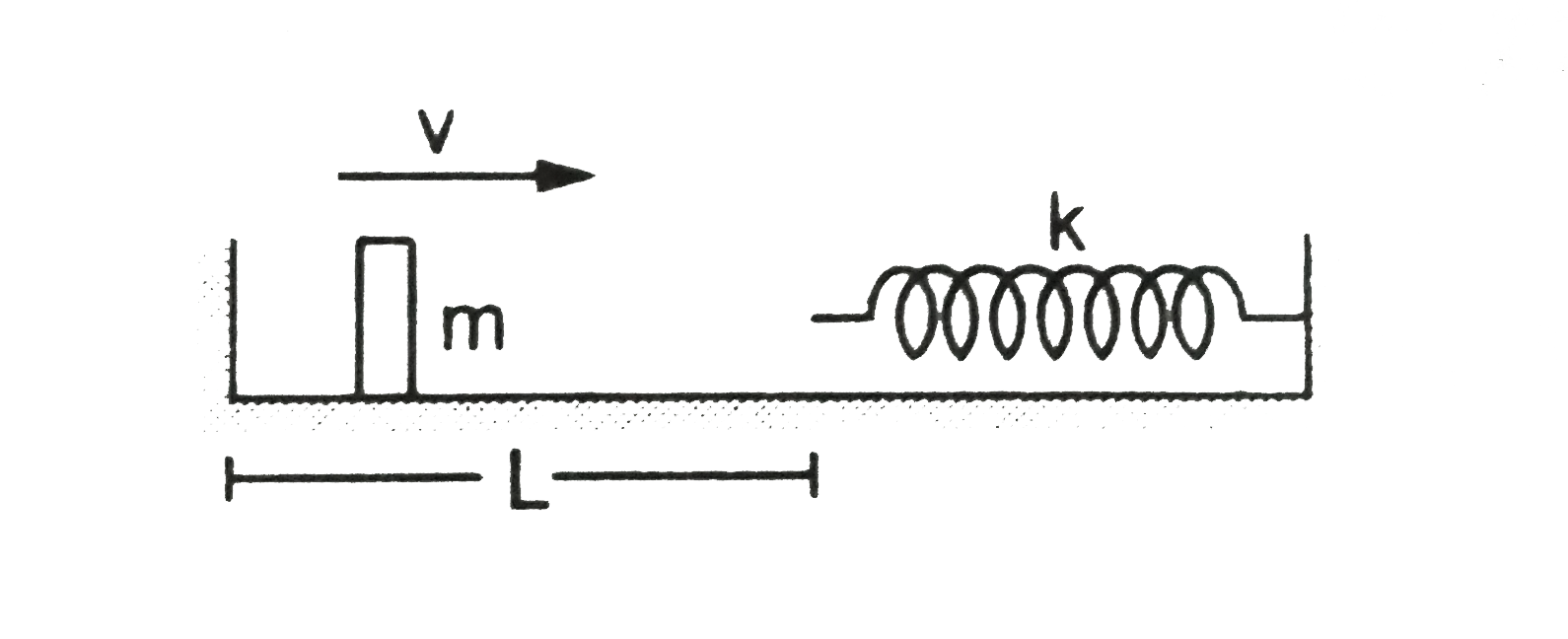 View Solution
View SolutionThe friction coefficient between the horizontal surfce and ech of the blocks shown in figure is 0.20. The collision between the blocks is perfectly elastic. Find the separation between the two blocks when they come to rest. Take g=10ms2.
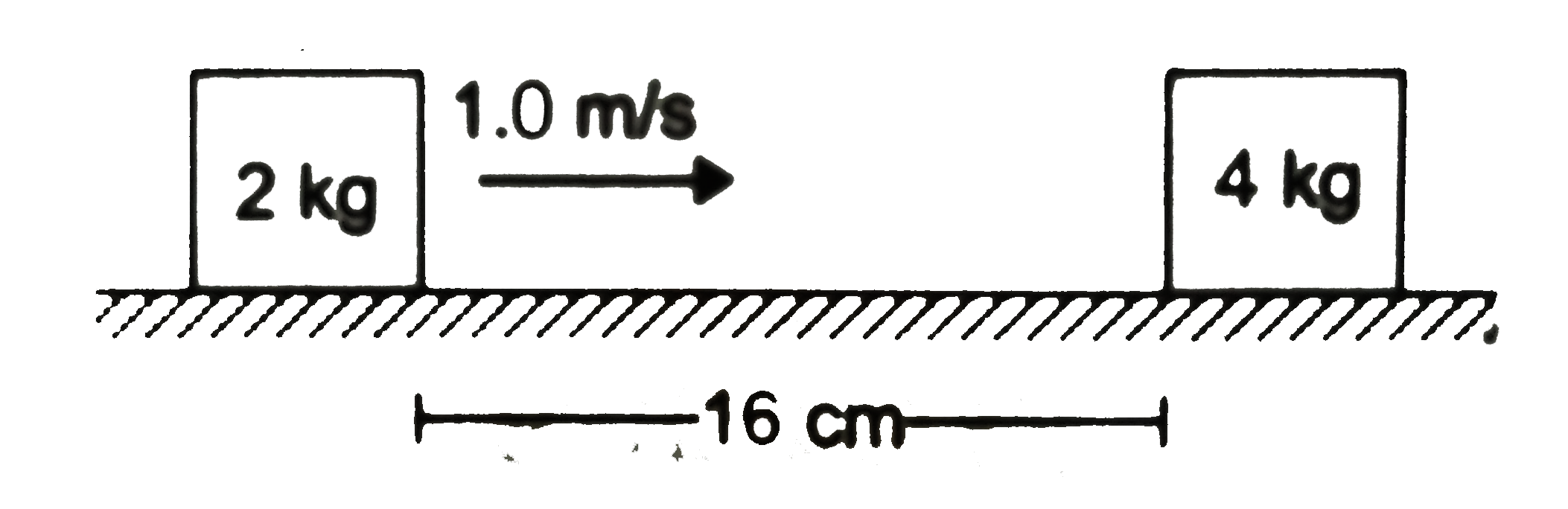 View Solution
View SolutionA block of mass m is lying at rest at point P of a wedge having a smooth semi-circular track of radius R. What should be the minimum value of a0 so that the mass can just reach point Q
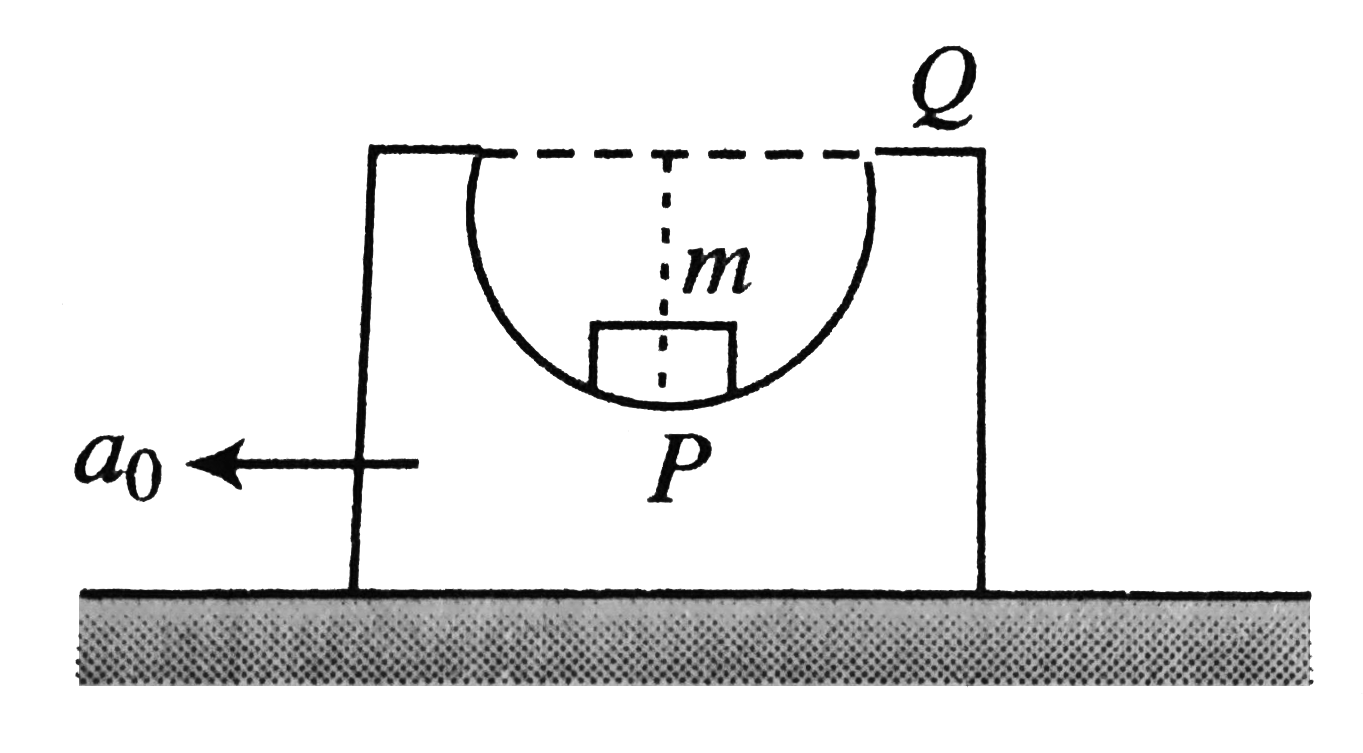 View Solution
View SolutionA small block B of mass m2 is placed on another block A of mass m1 A constant horizontal force F is applied to the block A. All the surfaces are assumed to be frictionless. Find the acceleration of center of mass of the system
View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?
 View Solution
View Solution