A ball kept in a close box moves in the box making collisions with the walls. The box is kept on a smooth surface. The velocity of the centre of mass
of the box remains constant
of the box plus the ball sytem remains constant
of the ball remains constant
of the ball relative to the box remains constant.
The correct Answer is:B
To solve the problem regarding the motion of a ball inside a closed box on a smooth surface, we will analyze the situation step by step.
1. Understanding the System:
- We have a ball inside a closed box. The box is placed on a smooth surface, which means there is negligible friction acting on the box.
2. Identifying Forces:
- When the ball collides with the walls of the box, it exerts a force on the wall, and the wall exerts an equal and opposite force on the ball (Newton's Third Law). This interaction is an internal force within the system (ball + box).
3. Momentum Conservation:
- Since the forces acting during the collision are internal to the system (ball and box), the total momentum of the system (ball + box) is conserved. There are no external forces acting on the system.
4. Center of Mass (COM) Concept:
- The center of mass of a system is defined as the point where the total mass of the system can be considered to be concentrated. The velocity of the center of mass (V_COM) is given by the total momentum of the system divided by the total mass.
5. Acceleration of the Center of Mass:
- Since there are no external forces acting on the system, the acceleration of the center of mass (a_COM) is zero. This implies that the velocity of the center of mass remains constant.
6. Conclusion:
- Therefore, the velocity of the center of mass of the system (ball + box) does not change as the ball moves and collides with the walls of the box. It remains constant throughout the motion.
Final Answer:
The velocity of the center of mass of the system (ball + box) remains constant.
- We have a ball inside a closed box. The box is placed on a smooth surface, which means there is negligible friction acting on the box.
- When the ball collides with the walls of the box, it exerts a force on the wall, and the wall exerts an equal and opposite force on the ball (Newton's Third Law). This interaction is an internal force within the system (ball + box).
- Since the forces acting during the collision are internal to the system (ball and box), the total momentum of the system (ball + box) is conserved. There are no external forces acting on the system.
- The center of mass of a system is defined as the point where the total mass of the system can be considered to be concentrated. The velocity of the center of mass (V_COM) is given by the total momentum of the system divided by the total mass.
- Since there are no external forces acting on the system, the acceleration of the center of mass (a_COM) is zero. This implies that the velocity of the center of mass remains constant.
- Therefore, the velocity of the center of mass of the system (ball + box) does not change as the ball moves and collides with the walls of the box. It remains constant throughout the motion.
The velocity of the center of mass of the system (ball + box) remains constant.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Exercises
64 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - Question For short Answer
25 Videos
- CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONBOOK - HC VERMA ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM, COLLISIONEXERCISE - worked out Examples
23 Videos
- CALORIMETRYBOOK - HC VERMA ENGLISHCHAPTER - CALORIMETRYEXERCISE - Short answer
9 Videos
- CIRCULAR MOTIONBOOK - HC VERMA ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Question for short Answer
10 Videos
Similar Questions
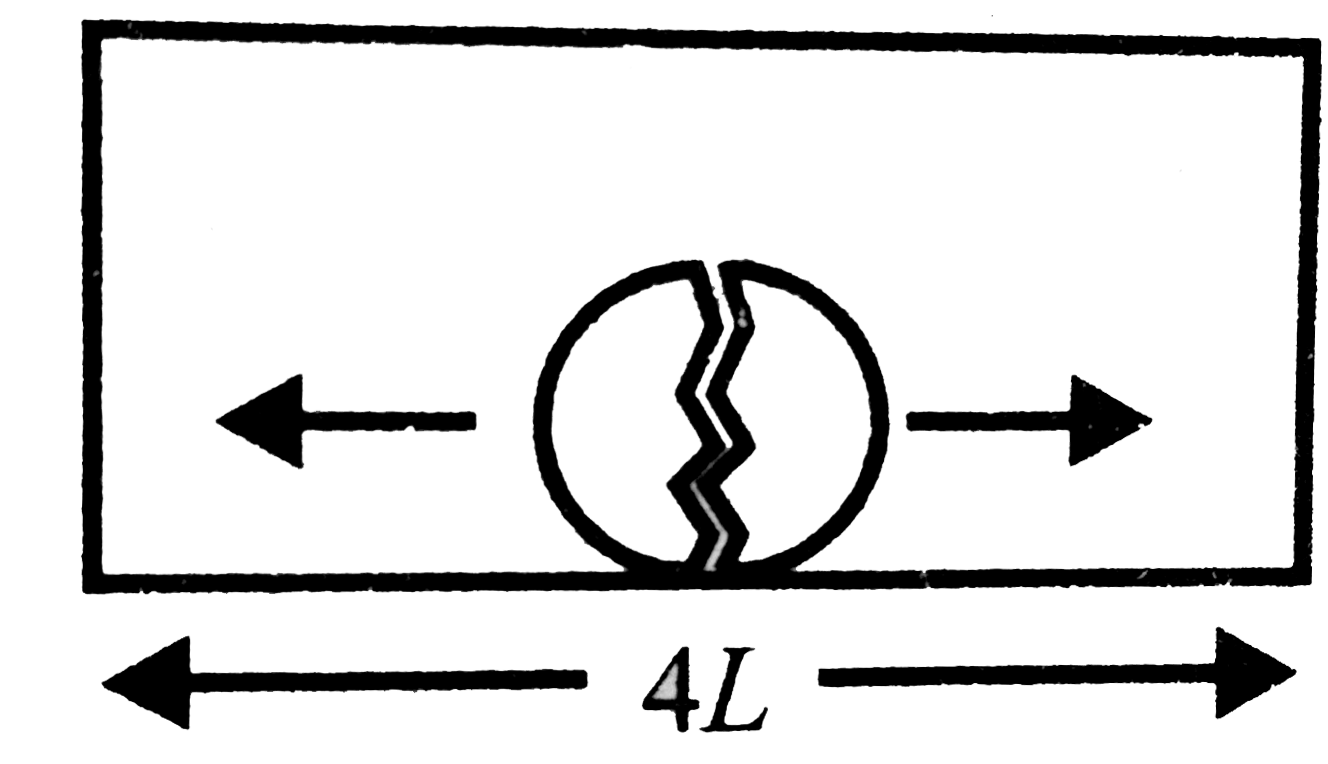
A bomb of mass 3m is kept inside a closed box of mass 3m and length 4L at its centre. It explodes in two parts of mass m and 2m. The two parts move in opposite directions and stick to the opposite sides of the walls of box. The box is kept on a smooth horizontal surface. What is the distance moved by the box during this time interval.
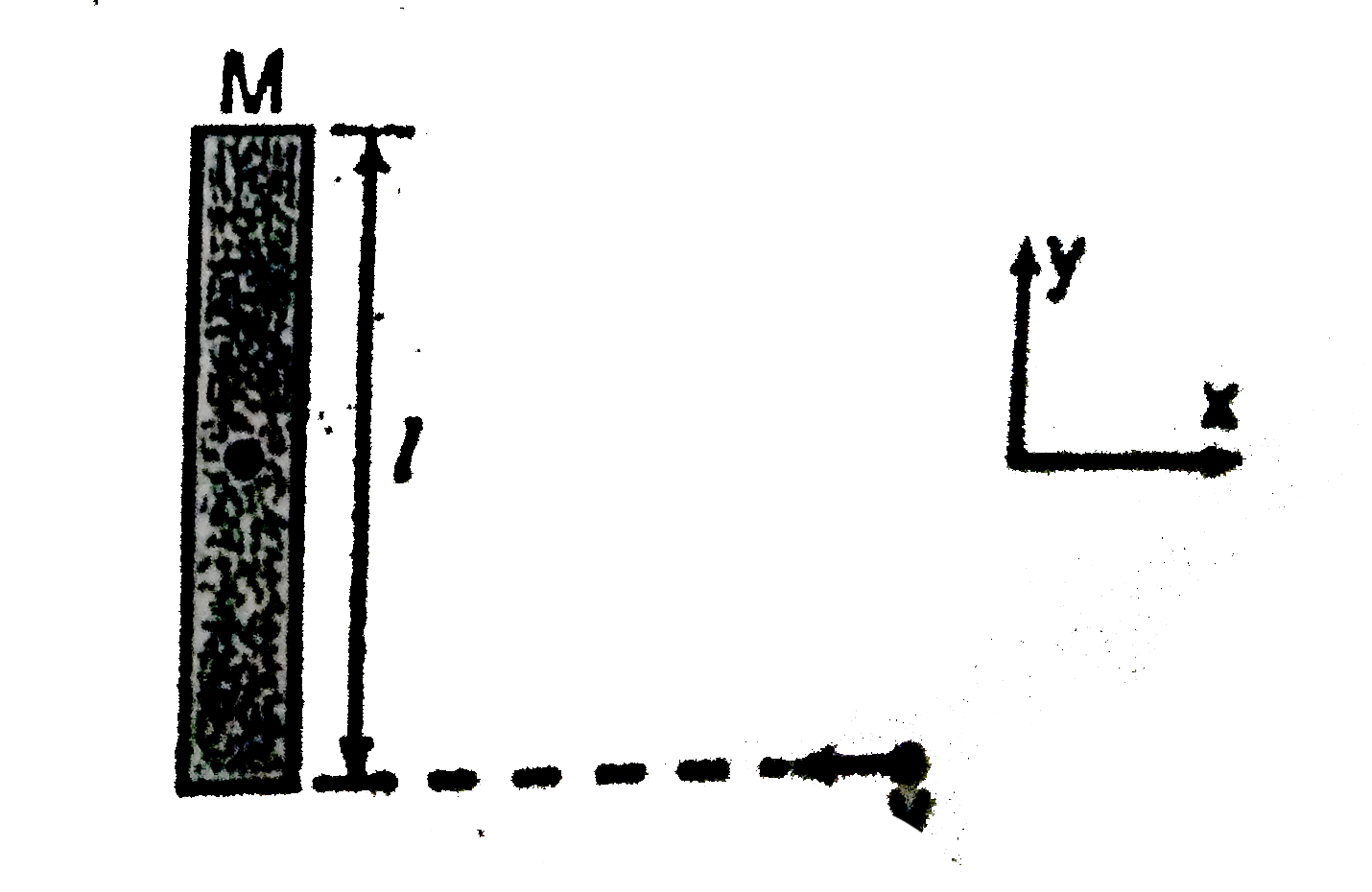
View SolutionA rod of mass m and length l is kept on a surface that has no friction. If a ball comes & hits the lower end with a velocity v, then the velocity of centre of mass will be
View SolutionTwo equal rods joined at one end are kept on a smooth surface as shown and released Trajectory of centre of mass of both rods is :−

View SolutionA cubical box containing 2 mole of He gas moves with constant speed u on a smooth horizontal surface. If the box suddenly stops, then determine the change in temperature of the gas by assuming mass of box to be negligible.
View SolutionA box of negligible mass containing 2 moles of an ideal gas of molar mass M and adiabatic exponent γ moves with constant speed v on a smooth horizontal surface. If the box suddenly stops, then change in temperature of gas will be :
View SolutionA ball of mass 4 kg moving on a smooth horizontal surface makes an elastic collision with another ball of mass m at rest in the line of motion of first ball. If after collision first ball moves in the same direction with one fourth of its velocity before collision, then mass of second ball is
View SolutionThere are 6 balls of different colours and 3 boxes of different sizes. Each box can hold all the 6 balls. The balls are put in the boxes so that no box remains expty. The number of ways in which this can be done is (A) 534 (B) 543 (C) 540 (D) 28
View SolutionA turck is moving on the ground with a velocity v=12m/s and a box is moving on the truck with respect to it with a velocity u=5m/s as shown in the figure. What is the velocity of the box with respect to the ground?

View SolutionA ball, rolling purely on a horizontal floor with centre's speed v, hits a smooth vertical wall surface elastically. Answer the following questions.

Just after the collision is over, the velocity of the lowest point of the ball is
View Solution7 different coloured balls to be kept in 5 different boxes. A box masy contain any number of balls and no box is to be empty (order of balls is considered in the boxes). Then number of such ways are (A) ⌊7 .7C4 (B) ⌊7.⌊5 .7C2 (C) ⌊7.⌊5 (D) none of these
View Solution
