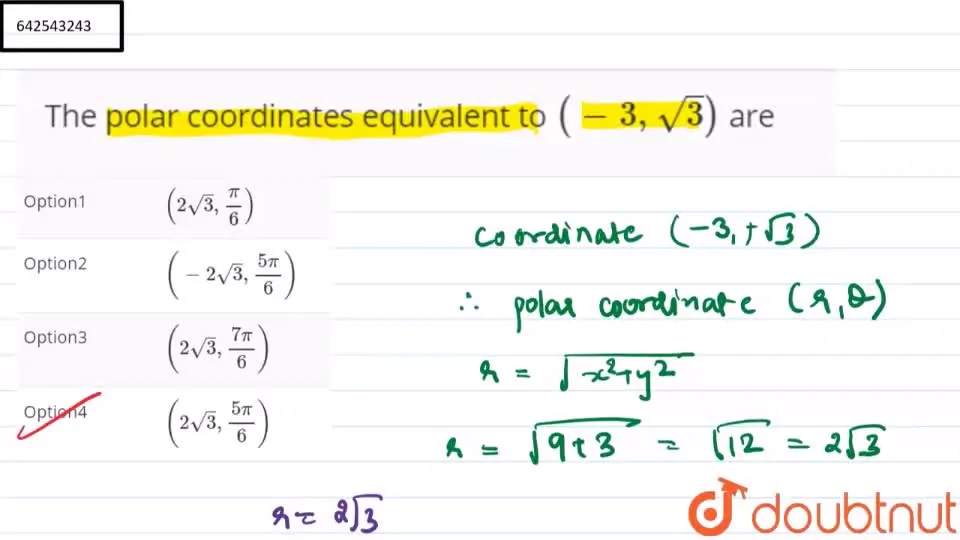
The polar coordinates equivalent to (−3,√3) are
(2√3,π6)
(−2√3,5π6)
(2√3,7π6)
(2√3,5π6)
The correct Answer is:D
r=√(x2+y2)=√9+3=2√3
α=tan−1.|y||x|
α=tan−1(√33)
α=tan−1(1√3)=π6
Point (−3,√3) lies in the second quadrant.
∴θ=π−α=π−π6=π6
So, polar coordinates are (2√3,5π2).
Related Playlists
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Multiple correct13videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Linked10videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.69videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE ENGLISHCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
Convert the polar coordinates to its equivalent Cartesian coordinates(2,π).
View SolutionLe n be the number of points having rational coordinates equidistant from the point (0,√3), the
View SolutionThe polar coordinates of the point whose cartesian coordinates are (-1, -1) is
View SolutionFind the polar coordinates of the points whose cartesian coordinates are
(−3,4)View SolutionA point has rectangular coordinates (3,4) . The polar coordinates are (5,θ) . What is the value of θ ?
View SolutionFind the polar coordinates of the points whose cartesian coordinates are
(−2,−2)View SolutionThe straight line 3x+4y−12=0 meets the coordinate axes at AandB . An equilateral triangle ABC is constructed. The possible coordinates of vertex C (a) (2(1−3√34),32(1−4√3)) (b)(−2(1+√3),32(1−√3)) (c)(2(1+√3),32(1+√3)) (d)(2(1+3√34),32(1+4√3))
View SolutionConvert the following Cartesian coordinates to the cooresponding polar coordinates using positive r.
(i) (1,−1)
(ii) (−3,−4)View SolutionExpress point P whose rectangular coordinates are (3,3√3) in terms of polar coordinates
r2=x2+y2=9+27=36
r=6
rcosθ=x
cosθ=36=12
Therefore θ=60∘ and (6,60∘) are the polar coordinates of PView SolutionThe polar coordinates of a point P are (2,200∘) The rectangular coordinates of P are
View Solution