(a) Draw a ray diagram to show the image formation by a combination of two thin convex
lenses in contact . Obtain the expression for the power of this combination in terms of the
focal lengths of the lenses.
(b) A ray of light passing from air through an equilateral glass prism undergoes minimum
deviation when the angle of incidence is 3(4)th of the angle of prism . Calculate the speed of ltbRgt light in the prism.
Step-by-Step Solution
1. Draw the Ray Diagram:
- Draw two thin convex lenses (L1 and L2) in contact with each other.
- Mark the object (O) placed in front of the first lens (L1).
- Draw the rays from the object passing through L1 and converging to form an image (P).
- The image formed by L1 acts as the object for L2.
- Draw the rays from this image passing through L2 and converging to form the final image (I).
2. Apply the Lens Formula for L1:
- The lens formula is given by:
1V′−1U=1F1
- Here, V′ is the image distance from L1, U is the object distance from L1, and F1 is the focal length of L1.
3. Apply the Lens Formula for L2:
- The image formed by L1 acts as the object for L2. Let this image distance be V.
- The lens formula for L2 is:
1V−1V′=1F2
- Here, F2 is the focal length of L2.
4. Combine the Equations:
- Adding the two equations gives:
1V−1U=1F1+1F2
- Let 1F=1F1+1F2, where F is the focal length of the combination.
5. Calculate the Power of the Combination:
- The power P of a lens is given by:
P=1F
- Therefore, the power of the combination of two lenses is:
P=P1+P2=1F1+1F2
Part (b): Speed of Light in the Prism
1. Given Information:
- The angle of the prism A=60∘.
- The angle of incidence I=34A=34×60∘=45∘.
2. Use the Minimum Deviation Condition:
- At minimum deviation, the angle of incidence I is equal to the angle of emergence E.
- Therefore, we have:
I+E=A+Dmin
- Substituting the values:
45∘+45∘=60∘+Dmin
- This gives:
Dmin=30∘
3. Calculate the Refractive Index:
- The formula for the refractive index μ of the prism is:
μ=sin(A+Dmin2)sin(A2)
- Substituting the values:
μ=sin(60∘+30∘2)sin(60∘2)=sin(45∘)sin(30∘)=√2212=√2≈1.41
4. Calculate the Speed of Light in the Prism:
- The speed of light in the prism V is given by:
V=Cμ
- Where C is the speed of light in vacuum (3×108m/s).
- Therefore:
V=3×1081.41≈2.13×108m/s
Final Answers:
- Part (a): The power of the combination of two thin convex lenses is given by:
P=P1+P2=1F1+1F2
- Part (b): The speed of light in the prism is approximately:
V≈2.13×108m/s
- Draw two thin convex lenses (L1 and L2) in contact with each other.
- Mark the object (O) placed in front of the first lens (L1).
- Draw the rays from the object passing through L1 and converging to form an image (P).
- The image formed by L1 acts as the object for L2.
- Draw the rays from this image passing through L2 and converging to form the final image (I).
- The lens formula is given by:
1V′−1U=1F1
- Here, V′ is the image distance from L1, U is the object distance from L1, and F1 is the focal length of L1.
- The image formed by L1 acts as the object for L2. Let this image distance be V.
- The lens formula for L2 is:
1V−1V′=1F2
- Here, F2 is the focal length of L2.
- Adding the two equations gives:
1V−1U=1F1+1F2
- Let 1F=1F1+1F2, where F is the focal length of the combination.
- The power P of a lens is given by:
P=1F
- Therefore, the power of the combination of two lenses is:
P=P1+P2=1F1+1F2
- The angle of the prism A=60∘.
- The angle of incidence I=34A=34×60∘=45∘.
- At minimum deviation, the angle of incidence I is equal to the angle of emergence E.
- Therefore, we have:
I+E=A+Dmin
- Substituting the values:
45∘+45∘=60∘+Dmin
- This gives:
Dmin=30∘
- The formula for the refractive index μ of the prism is:
μ=sin(A+Dmin2)sin(A2)
- Substituting the values:
μ=sin(60∘+30∘2)sin(60∘2)=sin(45∘)sin(30∘)=√2212=√2≈1.41
- The speed of light in the prism V is given by:
V=Cμ
- Where C is the speed of light in vacuum (3×108m/s).
- Therefore:
V=3×1081.41≈2.13×108m/s
- Part (a): The power of the combination of two thin convex lenses is given by:
P=P1+P2=1F1+1F2
- Part (b): The speed of light in the prism is approximately:
V≈2.13×108m/s
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION-C
50 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) DELHI BOARD -2016 [SET -I][COMPTT.]
11 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION-A
28 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
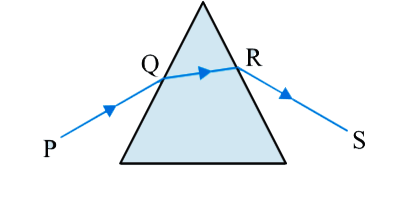
A ray of light passing through an equilateral triangular glass prism from air undergoes minimum deviation when angle of incidence is 34th of the angle of prism. Calculate speed of light in prism.
View SolutionA ray of light passing through an equilateral triangular glass prism from air undergoes minimum deviation when angle of incidence is 3/4 th of the angle of prism. Calculate the speed of light in the prism.
View Solution
Knowledge Check
A ray of light passes through an equilateral prism (refractive index 1.5) such that angle of incidence is equal to angle of emergence and the latter is equal to 3/4th of the angle of prism. Calculate the angle of deviation.
A60∘B30∘C45∘D120∘
(a) Draw a ray diagram showing the image formation by a compound microscope. Hence obtain expression for total magnification when the image is formed at infinity.
View SolutionA ray of light passes through the equilateral prism such that angle of incidence is equal to the angle of emergence if the angle of incidence is 45∘. The angle of deviation will be
View SolutionDraw ray diagrams showing the image formation by a convex mirror when an object is placed
(a) at infinity (b) at finite distance from the mirror
View SolutionA ray of light is incident on an equilateral glass prism placed on a horizontal table. For minimum deviation which of the following is true:
View SolutionWrite a relation for the angle of deviation (δ) for a ray of light passing through an equilateral prism in terms of the angle of incidence (i1), angle of emergence (i2) ang angle of prism (A).
View SolutionA ray of light passes through an equilateral glass prism in such a manner that the angle of incidence is equal to the angle of emergence and each of these angles is equal to 3/4 of the angle of the prism. The angle of deviation is
View SolutionA ray of light passing through a prism having refractive index √2 suffers minimum deviation. It is found that the angle of incidence is double the angle of refraction within the prism. What is the angle of prism?
View Solution
