A charge q is placed at the centre of a cube of side l what is the electric flux passing through two opposite faces of the cube ?
To solve the problem of finding the electric flux passing through two opposite faces of a cube with a charge q placed at its center, we can follow these steps:
1. Understand the Setup:
- We have a cube with side length l.
- A charge q is placed at the center of this cube.
2. Apply Gauss's Law:
- According to Gauss's Law, the total electric flux Φ through a closed surface is given by:
Φ=Qencϵ0
- Here, Qenc is the charge enclosed by the surface, and ϵ0 is the permittivity of free space.
3. Calculate Total Flux Through the Cube:
- Since the charge q is at the center of the cube, the total flux through the entire surface of the cube is:
Φtotal=qϵ0
4. Determine Flux Through One Face:
- The cube has 6 faces, and due to symmetry, the electric flux is uniformly distributed across all faces.
- Therefore, the flux through one face of the cube is:
Φone face=Φtotal6=q6ϵ0
5. Calculate Flux Through Two Opposite Faces:
- To find the total flux through two opposite faces, we simply add the flux through each of these faces:
Φtwo opposite faces=Φone face+Φone face=2×q6ϵ0=2q6ϵ0=q3ϵ0
Final Answer:
The electric flux passing through two opposite faces of the cube is:
Φ=q3ϵ0
- We have a cube with side length l.
- A charge q is placed at the center of this cube.
- According to Gauss's Law, the total electric flux Φ through a closed surface is given by:
Φ=Qencϵ0
- Here, Qenc is the charge enclosed by the surface, and ϵ0 is the permittivity of free space.
- Since the charge q is at the center of the cube, the total flux through the entire surface of the cube is:
Φtotal=qϵ0
- The cube has 6 faces, and due to symmetry, the electric flux is uniformly distributed across all faces.
- Therefore, the flux through one face of the cube is:
Φone face=Φtotal6=q6ϵ0
- To find the total flux through two opposite faces, we simply add the flux through each of these faces:
Φtwo opposite faces=Φone face+Φone face=2×q6ϵ0=2q6ϵ0=q3ϵ0
The electric flux passing through two opposite faces of the cube is:
Φ=q3ϵ0
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) [SET - II]
6 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) [SET - III]
9 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - [Set-III]
3 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
A charge q is placed at the centre of a cube. What is the electric flux passing through the cube?
View SolutionCharge of 2 C is placed at the centre of a cube. What is the electric flux passing through one face ?
View SolutionA charge +q is placed at the mid point of a cube of side L. The electric flux emerging from cube is
View SolutionA charge qμC is placed at the centre of a cube of a side 0.1m, then the electric flux diverging from each face of the cube is
View SolutionAn electric dipole is placed at the centre of a sphere. Find the electric flux passing through the sphere.
View SolutionThe length of each side of a cubical closed surface is l If charge q is situated on one of the vertices of the cube , then find the electric flux passing through shaded face of the cube.
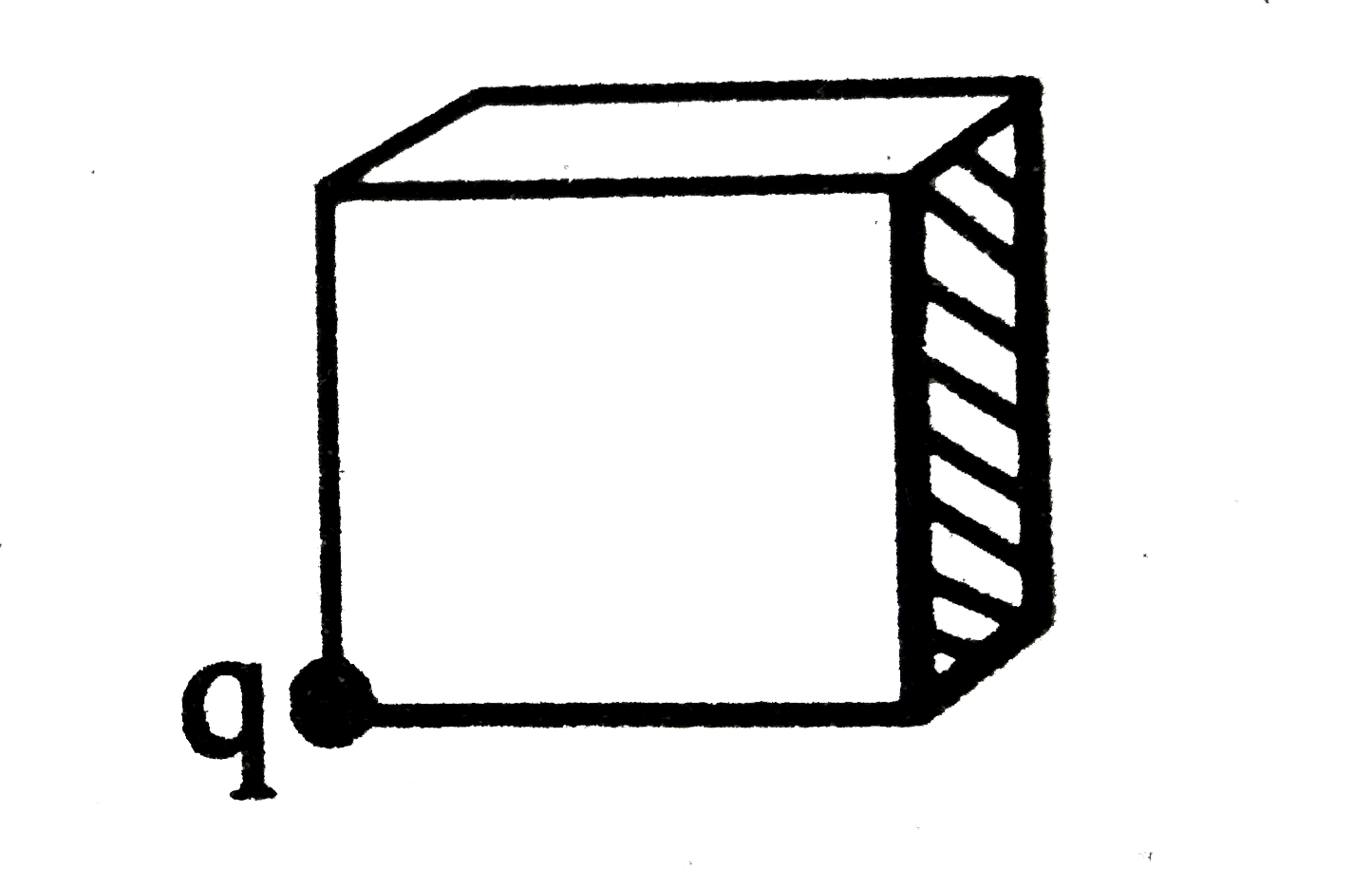 .
.
View SolutionA charge q is located at the centre of a cube. The electric flux through any face is
View SolutionA charge q is located at the centre of a cube. The electric flux through any face is
View SolutionA charge Q is situated at the corner of a cube, the electric flux passing through all the six faces of the cube is :
View SolutionA charge q is placed at point D of the cube. Find the electric flux passing through the EFGH and face AEHD.
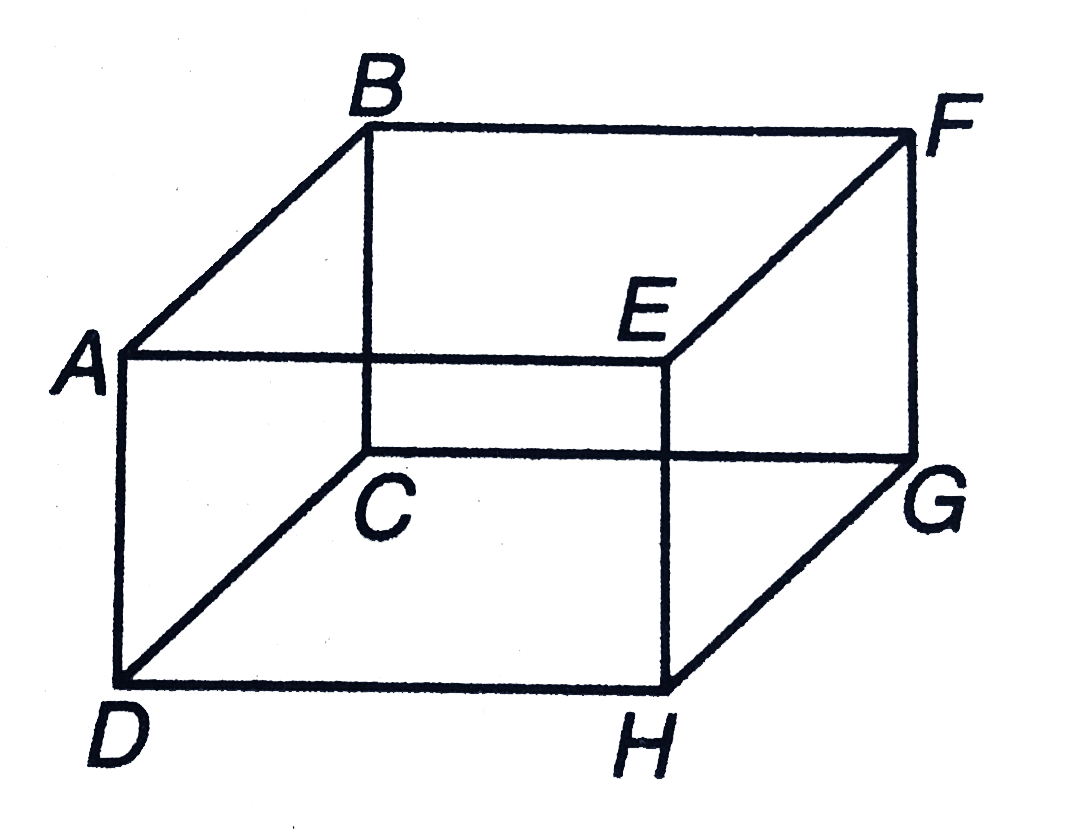
View Solution
